- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
Andrew Browder in his book: "Mathematical Analysis: An Introduction" ... ... defines a differential form in Section 13.1 which reads as follows:
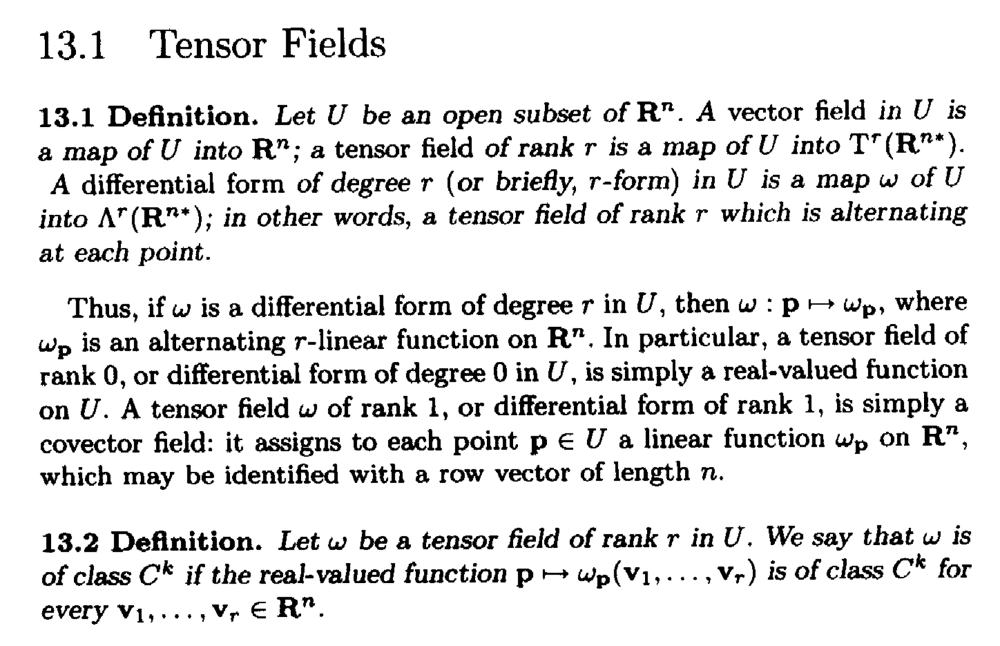
In the above text from Browder we read the following:
" ... ... A differential form of degree ##r## (or briefly an ##r##-form) in ##U## is a map ##\omega## of ##U## into ## { \bigwedge}^r V ( \mathbb{R}^{ n \ast } )## ... ... "In other words if ##\omega## is a differential form of degree ##r## in ##U##, then we have
##\omega : p \to \omega_p ( v_1, \cdot \cdot \cdot , v_r )##Browder, unfortunately, gives no example of a differential form ... but I found an introductory example of a ##2##-form on page 6 of Steven Weintraub's book: Differential Forms: Theory and Practice ... the example (Example 1.1.3 (2) ) reads as follows:
" ... ...
##\phi = xyz \text{ dy } \text{ dz } + x e^y \text{ dz } \text{ dx } + 2 \text{ dx } \text{ dy }##
My question is as follows:
How do we interpret Weintraub's example in terms of Browder's definition of a differential form ... and further, how do we translate Weintraub's example into an example in Browder's notation/definition ... ...Help will be much appreciated ... ...
Peter=========================================================================================
So that readers can access Weintraub's definition and notation I am providing the relevant text ... as follows:

Hope that helps,
Peter
In the above text from Browder we read the following:
" ... ... A differential form of degree ##r## (or briefly an ##r##-form) in ##U## is a map ##\omega## of ##U## into ## { \bigwedge}^r V ( \mathbb{R}^{ n \ast } )## ... ... "In other words if ##\omega## is a differential form of degree ##r## in ##U##, then we have
##\omega : p \to \omega_p ( v_1, \cdot \cdot \cdot , v_r )##Browder, unfortunately, gives no example of a differential form ... but I found an introductory example of a ##2##-form on page 6 of Steven Weintraub's book: Differential Forms: Theory and Practice ... the example (Example 1.1.3 (2) ) reads as follows:
" ... ...
##\phi = xyz \text{ dy } \text{ dz } + x e^y \text{ dz } \text{ dx } + 2 \text{ dx } \text{ dy }##
My question is as follows:
How do we interpret Weintraub's example in terms of Browder's definition of a differential form ... and further, how do we translate Weintraub's example into an example in Browder's notation/definition ... ...Help will be much appreciated ... ...
Peter=========================================================================================
So that readers can access Weintraub's definition and notation I am providing the relevant text ... as follows:
Hope that helps,
Peter
Attachments
Last edited:




