- #1
Pakano
- 3
- 0
What happen if a radially polarized beam is extremely focused to a single spot?
Is it disappeared because E-fields in opposite direction subtract each other?
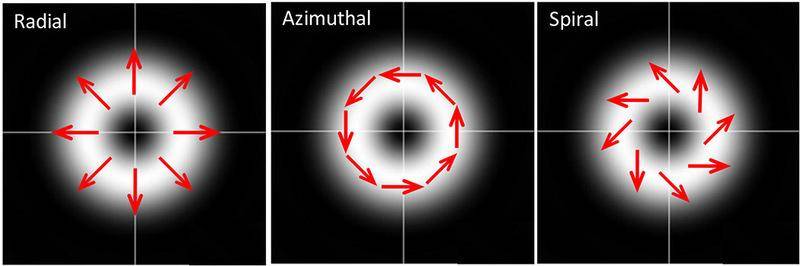
Is it disappeared because E-fields in opposite direction subtract each other?
