- #1
chwala
Gold Member
- 2,650
- 351
- Homework Statement
- Find the second derivative, ##\dfrac{d^2y}{dx^2}## for the relation;
##x^2+y^4=10##
- Relevant Equations
- differentiation
Find text (question and working to solution here ...this is very clear to me...on the use of implicit differentiation and quotient rule to solution). I am seeking an alternative approach.
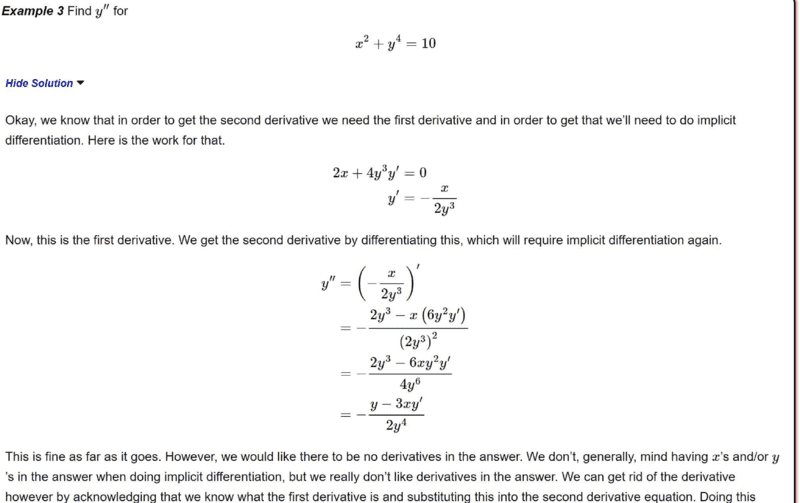
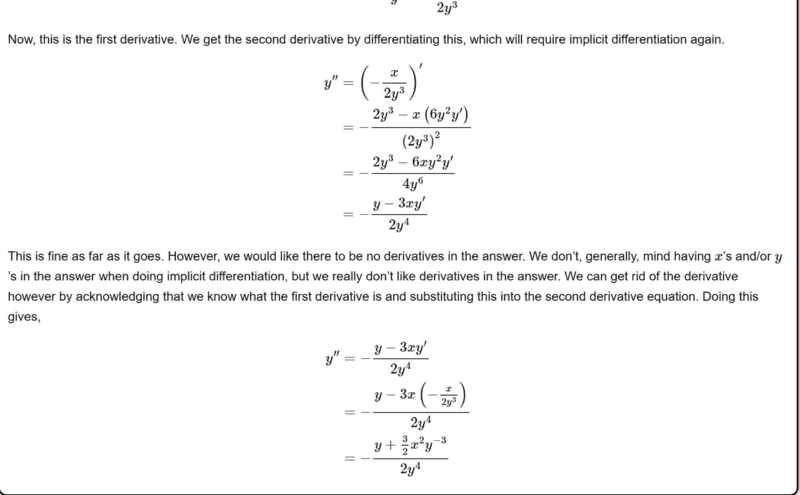
Now from my study we can also have; using partial derivatives...
##\dfrac{d^2y}{dx^2}=\dfrac{-1}{2y^3}+\left[\dfrac{-x}{2}⋅\dfrac{-3}{y^4}⋅\dfrac{-x}{2y^3}\right]##
##\dfrac{d^2y}{dx^2}=-\dfrac{1}{2y^3}-\dfrac{3x^2}{4y^7}##
##\dfrac{d^2y}{dx^2}=\dfrac{-2y^4-3x^2}{2y^7}##
##\dfrac{d^2y}{dx^2}=-\left[\dfrac{2y^4+3x^2}{2y^7}\right]##
your thoughts...any other approach is welcome guys...
the text book solution is;
##\dfrac{d^2y}{dx^2}=-\left[\dfrac{y+1.5x^2y^{-3}}{2y^4}\right]## ...just in case it is not visible enough...
Now from my study we can also have; using partial derivatives...
##\dfrac{d^2y}{dx^2}=\dfrac{-1}{2y^3}+\left[\dfrac{-x}{2}⋅\dfrac{-3}{y^4}⋅\dfrac{-x}{2y^3}\right]##
##\dfrac{d^2y}{dx^2}=-\dfrac{1}{2y^3}-\dfrac{3x^2}{4y^7}##
##\dfrac{d^2y}{dx^2}=\dfrac{-2y^4-3x^2}{2y^7}##
##\dfrac{d^2y}{dx^2}=-\left[\dfrac{2y^4+3x^2}{2y^7}\right]##
your thoughts...any other approach is welcome guys...
the text book solution is;
##\dfrac{d^2y}{dx^2}=-\left[\dfrac{y+1.5x^2y^{-3}}{2y^4}\right]## ...just in case it is not visible enough...
Last edited: