- #1
mateomy
- 307
- 0
My classmates and I are having a debate on how to solve the following problem. We are told that (and this has been posted on this message board before) that a planet of mass M and radius R is moving SLOWLY through a dust cloud of density (rho). Some of the particles will be attracted to the planet. Find the retarding force on the planet from the dust particles.
I think that if you set up a typical gravitational force problem and solve the smaller mass in terms of the mass of the dust you get something as such:
[tex]
\frac{-4GM\rho\pi R}{3}
[/tex]
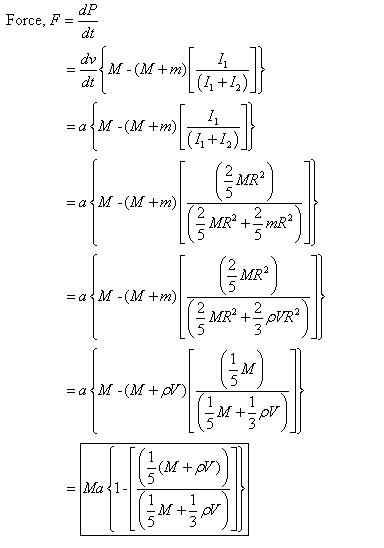
On the other hand a few of the others think that it can be solved using angular momentum….as so:
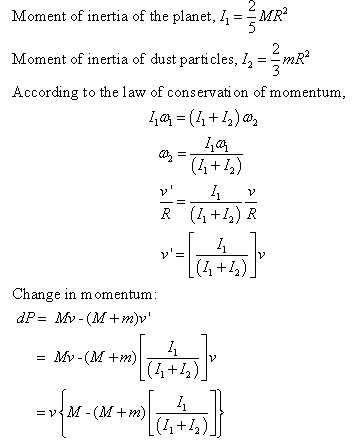
Adding…
The argument against my idea is that it only treats the planet as if it were going through a cylinder picking up what was only directly in front of it. I don't see that validity in that argument. Can anybody put in a fresh word on the subject? Please?
I think that if you set up a typical gravitational force problem and solve the smaller mass in terms of the mass of the dust you get something as such:
[tex]
\frac{-4GM\rho\pi R}{3}
[/tex]
On the other hand a few of the others think that it can be solved using angular momentum….as so:
Adding…
The argument against my idea is that it only treats the planet as if it were going through a cylinder picking up what was only directly in front of it. I don't see that validity in that argument. Can anybody put in a fresh word on the subject? Please?