- #1
thatsmessedup
- 27
- 0
- Homework Statement
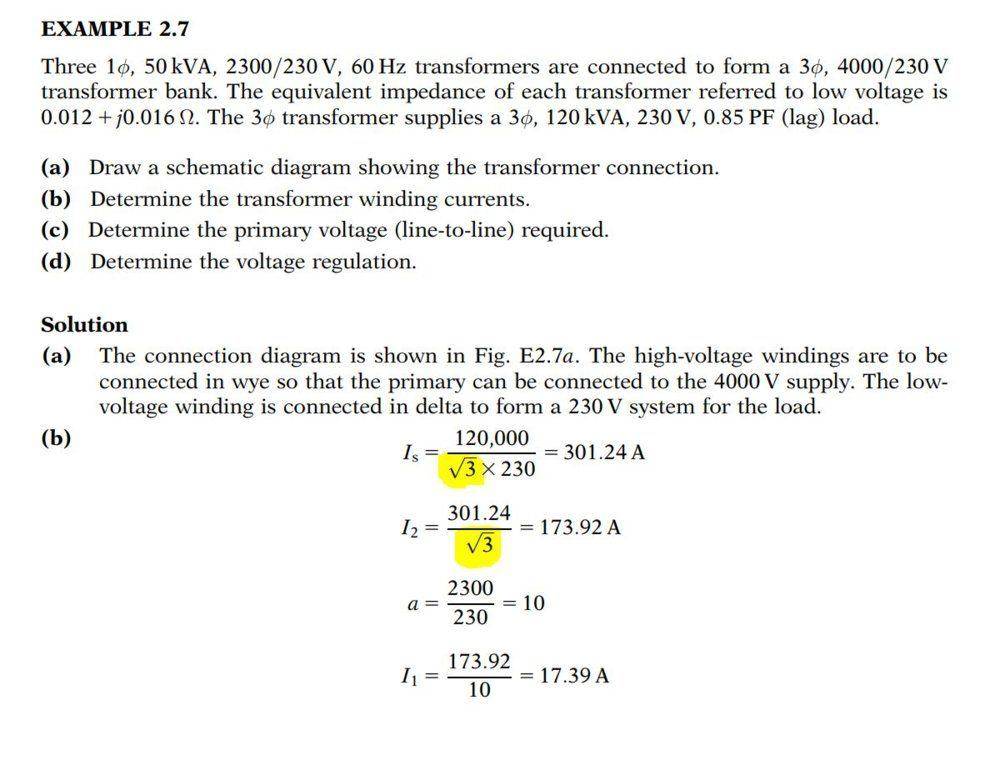
- EXAMPLE 2.7
Three 1φ, 50 kVA, 2300=230 V, 60 Hz transformers are connected to form a 3φ, 4000=230 V
transformer bank. The equivalent impedance of each transformer referred to low voltage is
0:012 + j0:016 Ω. The 3φ transformer supplies a 3φ, 120 kVA, 230 V, 0:85 PF (lag) load.
(a) Draw a schematic diagram showing the transformer connection.
(b) Determine the transformer winding currents.
(c) Determine the primary voltage (line-to-line) required.
(d) Determine the voltage regulation
- Relevant Equations
- Please see attached
In this example I am thoroughly confused on why Is is divided by sqrt(3). My understanding is that the transformer supplies 3 phase (which is always described in line to line or Y connected). So the current in the winding should be 120[KVA]/230[V] then multiplied by sqrt(3) to convert it to delta line current. Then I2 would be Is divided by sqrt(3) to get the phase current.
Where are the appropiate formulas or diagrams that will make this problem make more sense?
Thanks!
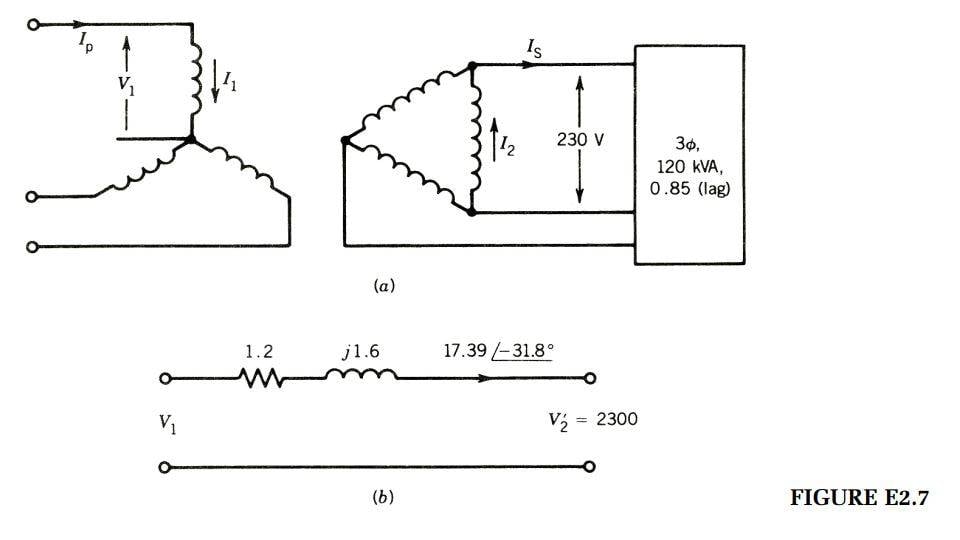
This is where I am seeing that I should multiply sqrt(3) to get the delta line current. (exuding the a because I am not going from one transformer to the other)
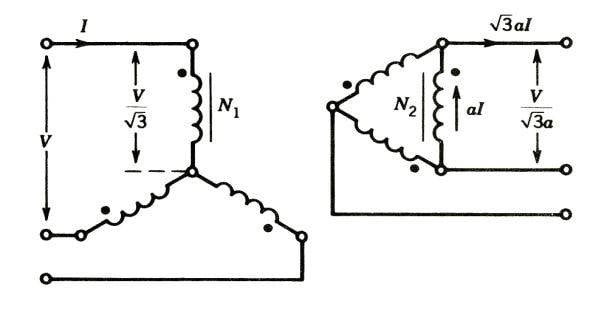
Where are the appropiate formulas or diagrams that will make this problem make more sense?
Thanks!
This is where I am seeing that I should multiply sqrt(3) to get the delta line current. (exuding the a because I am not going from one transformer to the other)
Attachments
Last edited by a moderator:
