- #1
Luke Tan
- 29
- 2
In his book, Landau derives the Lorentz transformations using the invariance of the interval, and I have some questions about it that I would like to clarify

1. What is a parallel displacement of a coordinate system?
Does it refer to moving along any axis?
I don't see how any arbitrary translation of the origin will change the interval between any two events
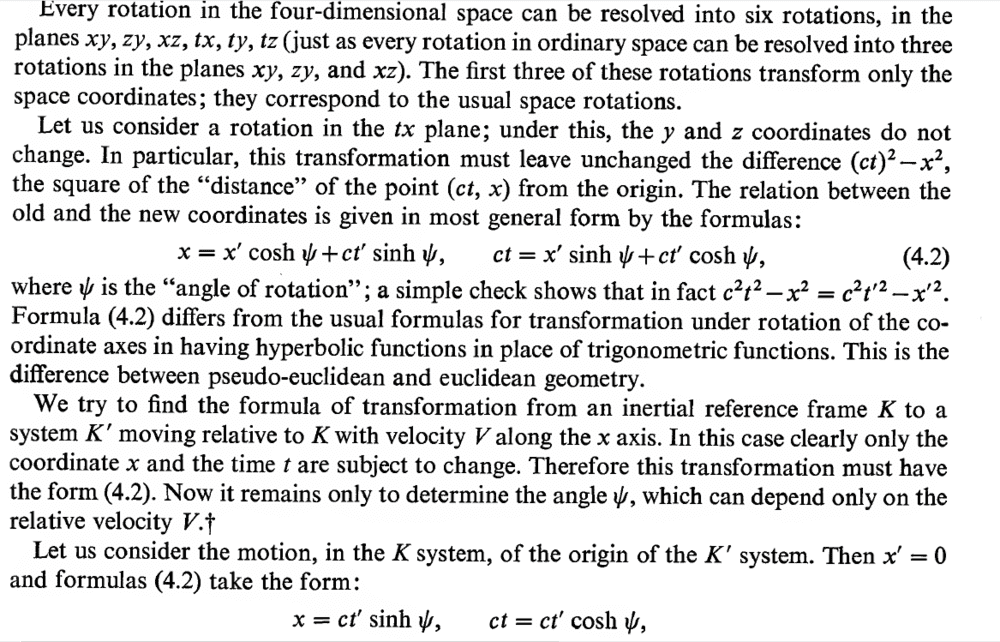
2. Does this assume that the origins of the two coordinate systems coincide?
The interval between two events is ##s^2=c^2(t_2-t_1)^2-(x_2-x_1)^2##
For this to reduce to ##s = c^2t^2-x^2## this requires that ##x_1## is zero. However, considering that ##x_1## and ##t_1## are the coordinates of the origin, this would imply that in his equations he has assumed that the origin of both systems coincide.
While I understand that this is correct at some instant, would this not become inaccurate as time passes and the origin of one coordinate system moves away?
Any help would be appreciated
Thanks!
1. What is a parallel displacement of a coordinate system?
Does it refer to moving along any axis?
I don't see how any arbitrary translation of the origin will change the interval between any two events
2. Does this assume that the origins of the two coordinate systems coincide?
The interval between two events is ##s^2=c^2(t_2-t_1)^2-(x_2-x_1)^2##
For this to reduce to ##s = c^2t^2-x^2## this requires that ##x_1## is zero. However, considering that ##x_1## and ##t_1## are the coordinates of the origin, this would imply that in his equations he has assumed that the origin of both systems coincide.
While I understand that this is correct at some instant, would this not become inaccurate as time passes and the origin of one coordinate system moves away?
Any help would be appreciated
Thanks!