Acceleration of a Block Sliding on a Wedge

[Saitama]

Homework Statement

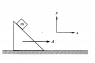
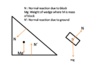
A 45 degree wedge is pushed along a table with constant acceleration A. A block of mass m slides without friction on the wedge. Find its acceleration. (Gravity is directed downwards.)

Homework Equations

The Attempt at a Solution

Let the acceleration of block with respect to wedge be $b$. Then in the ground frame acceleration of block in x and y direction is
$$\ddot{x}=A+b\sin(45^o)$$
$$\ddot{y}=-b\cos(45^o)$$
But what do I have to substitute for b here? gsin(45)? Pardon if this is a simple question but I don't have the final answers to this problem so I need someone to check my work.

Thank you.

 

[TSny]

You'll need to find b using some dynamics.

(If you're allowed to use fictitious forces, you can go to a frame moving with the wedge.)

 

[WannabeNewton]

Make sure you remember to write down the constraint equation for the system, in whatever frame you're working in.

 

[haruspex]

Make sure you remember to write down the constraint equation for the system, in whatever frame you're working in.

Seems to me that's what has been done so far. What's missing are equations involving g.
Pranav-Arora, why not just do the usual free-body analysis?

 

[Saitama]

Seems to me that's what has been done so far. What's missing are equations involving g.
Pranav-Arora, why not just do the usual free-body analysis?

I have attached the FBD's but I still don't see what I have to do next.

 

[BruceW]

The FBD for the wedge is not so important, since you know it is being forced to move along at constant acceleration. But the FBD of the block is good. Now what do you usually do as the next step in this type of question? Also, the equation you wrote in your first post is useful, but the parameter b is annoying. (hint).

 

[Enigman]

Perhaps the rotation of coordinate system may make them problem simpler?

 

[Saitama]

The FBD for the wedge is not so important, since you know it is being forced to move along at constant acceleration. But the FBD of the block is good. Now what do you usually do as the next step in this type of question? Also, the equation you wrote in your first post is useful, but the parameter b is annoying. (hint).

If the wedge is fixed, b would be gsin(45) but here the wedge is moving so plugging in b=gsin(45) would be incorrect, I think. Any more hints?

 

[Eats Dirt]

If the wedge is fixed, b would be gsin(45) but here the wedge is moving so plugging in b=gsin(45) would be incorrect, I think. Any more hints?

I think to solve it you must first find the acceleration from gravity only, a=gcos(45).
But this is in the wrong plane for vector addition.

So then I think you must split it (a=gcos(45)) into components that are parallel and perpendicular to A. Which you can add to the acceleration of the wedge A. Then find the overall acceleration using the Pythagorean theorem.

 

[voko]

As suggested by TSny, use a frame co-moving with the wedge. In that frame, you have some net force. And, as WannabeNewton remarked, the block is constrained to move along a particular line.

 

[BruceW]

If the wedge is fixed, b would be gsin(45) but here the wedge is moving so plugging in b=gsin(45) would be incorrect, I think. Any more hints?

You don't need to find b to answer the question. I suppose you could do it that way if you want. Also, I would use the reference frame of the ground, but I guess you could use a non-inertial reference frame instead. Anyway, you have $\ddot{x}$ and $\ddot{y}$ in the equation in your first post. That's a start. How do they relate to your FBD?

 

[haruspex]

I have attached the FBD's but I still don't see what I have to do next.

You can do all sorts of fancy things using different reference frames and axis rotations, but there's a risk of getting confused that way. It really isn't hard doing it by everyday methods.
Based on your FBD for the block, what are the horizontal and vertical accelerations (in terms of N etc.)? Equating those to what you originally posted will give you two equations with two unknowns (N, b).

 

[Saitama]

As suggested by TSny, use a frame co-moving with the wedge. In that frame, you have some net force. And, as WannabeNewton remarked, the block is constrained to move along a particular line.

I will do that once I have done this question in the ground frame. The book I currently follow hasn't yet started with fictitious forces.

You can do all sorts of fancy things using different reference frames and axis rotations, but there's a risk of getting confused that way. It really isn't hard doing it by everyday methods.
Based on your FBD for the block, what are the horizontal and vertical accelerations (in terms of N etc.)? Equating those to what you originally posted will give you two equations with two unknowns (N, b).

$$\ddot{x}+\ddot{y}=\frac{N}{\sqrt{2}m}\hat{i}+\frac{1}{m}\left(-mg+\frac{N}{\sqrt{2}}\right)\hat{j}$$
Equating.
$$\frac{N}{\sqrt{2}m}=A+\frac{b}{\sqrt{2}} \Rightarrow b=\frac{N}{m}-\sqrt{2}A$$
$$-g+\frac{N}{\sqrt{2}m}=-\frac{b}{\sqrt{2}} \Rightarrow \frac{N}{m}=\frac{A+g}{\sqrt{2}}$$
Hence,
$$b=\frac{g-A}{\sqrt{2}} \Rightarrow \ddot{y}=\frac{g-A}{2}$$

I think this is the correct answer as it satisfies the given clue about the answer. Thanks haruspex!

In the frame of moving wedge, a fictitious force, mA acts on the block in the direction of negative x-axis. The acceleration of the block (b) is along the incline hence,
$$\frac{mg}{\sqrt{2}}-\frac{mA}{\sqrt{2}}=mb \Rightarrow b=\frac{g-A}{\sqrt{2}}$$
The y component of b can be easily calculated. I think this method is much shorter but doing this problem in ground frame is also nice. Thanks! :)

 

[BruceW]

Hence,
$$b=\frac{g-A}{\sqrt{2}} \Rightarrow \ddot{y}=\frac{g-A}{2}$$

Nice work man. Just one small mistake at the last step - b is the opposite sign to the y acceleration. But here, you've neglected the change of sign. Also, yeah I see what you mean, the problem is quite nice in the non-inertial frame. I tend to avoid them though, to reduce the chance I will get confused :)

 

[voko]

I should say I am puzzled by the "confused" attitude. If something gets confusing in a problem, then avoid that in the problem. Non-inertial frames are a tool, and like any tool, may be good for some tasks but not too good for others. Being able to choose the right tool to attack a problem is as important in studying physics as it is to apply a particular tool.

In this problem, using an appropriate non-inertial frame is straight-forward and is clearly superior to using a ground frame.

 

[WannabeNewton]

I would have to agree with voko that employing a non-inertial frame makes this problem particularly simple in form (both the constraint and the equations of motion). But if you haven't gotten a handle on the conceptual nature of linearly accelerating frames yet (you mentioned that it only shows up later in your text) then you should just do what you feel most comfortable doing.

As an aside, this problem (or rather a variant of it where there is a sphere instead of a block) is usually a very standard example when introducing Lagrangian mechanics because Lagrangian mechanics also provides an incredibly simple way to solve this kind of problem, so don't be surprised if you see it crop up again

 

[BruceW]

@voko yeah I guess that's true. I think it is because I've always been discouraged from using non-inertial reference frames, which is why I am distrustful towards them. Also, if the problem is relativistic, then it introduces annoying time-dilation. But that can be neglected as long as the acceleration is not too great. So for non-relativistic problems I suppose it is alright.

edit: also, even in non-relativistic problems, things like energy and momentum are not conserved. This might make a large problem become more confusing. But yeah, it is OK for a short problem.

 

[Saitama]

But if you haven't gotten a handle on the conceptual nature of linearly accelerating frames yet (you mentioned that it only shows up later in your text) then you should just do what you feel most comfortable doing.

I already know about working in an accelerating frame. The point is, the book hasn't used it in any of the examples in the current chapter and there's a separate chapter about the fictitious forces. I thought you might have recognised the source of the problem. It is from the book An Introduction to Mechanics by Daniel Kleppner and Robert Kolenkow.

 

[WannabeNewton]

I did recognize it as one of the problems from chapter 2 of Kleppner but I didn't know if you had gotten it from some other source or not because it is not an uncommon problem in various texts. But if you already know about working in a linearly accelerating frame then you can at least compare and contrast the two methods and see which one you like better. For this problem it doesn't make that much of a difference but you might come across later problems in which linearly accelerating frames make a huge difference and it is easier to appreciate the use of linearly accelerated frames. Kleppner gives some examples in chapter 8.

 

[Saitama]

But if you already know about working in a linearly accelerating frame then you can at least compare and contrast the two methods and see which one you like better.

Obviously switching to a non-inertial frame is better. :tongue2:

 

[voko]

If we go off the relativistic tangent, then we can employ the equivalence principle and conclude that for the block acceleration A is indistinguishable from gravity, so all the block experiences is just gravity - with its acceleration given by vector $(-A, -g)$. Its magnitude is $\sqrt{A^2 + g^2}$. Defining the line perpendicular to the direction of effective gravity to be the effective horizontal, its angle with the ground is $\frac {\pi} {2} - \arctan \frac g A$, and the effective angle of the incline is $\arctan \frac g A - \frac {\pi} {4}$

Then we can just use our "normal physics", where the acceleration of a mass sliding down an incline is given by $g \sin \theta$, which in this case becomes $\sqrt{A^2 + g^2} \sin (\arctan \frac g A - \frac {\pi} {4}) = \frac {A - g} {\sqrt{2} }$.

If we had to use any conservation laws, we could use those that we can normally use when dealing with gravity.

 

[BruceW]

ah yeah. That's a nice way to think about it. There is still the time dilation that bugs me. But it's negligible as long as the acceleration is not too large (which is true in most classical problems).