- #1
TeslaPow
- 40
- 1
Hello.
I need some guidance on how to find the fraction of molecules with KE between K1 and K2 from the Maxwell kinetic energy distribution function.
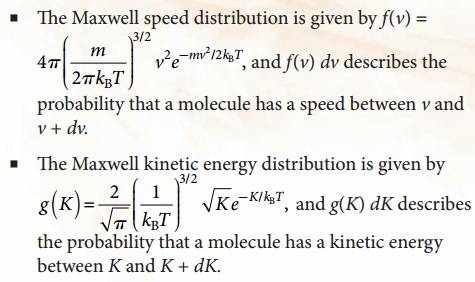
Here's an link to an earlier post where the speed distribution was integrated, how will I proceed with the kinetic energy distribution?
https://www.physicsforums.com/threads/maxwell-boltzmann-distribution.757539/#post-4772356
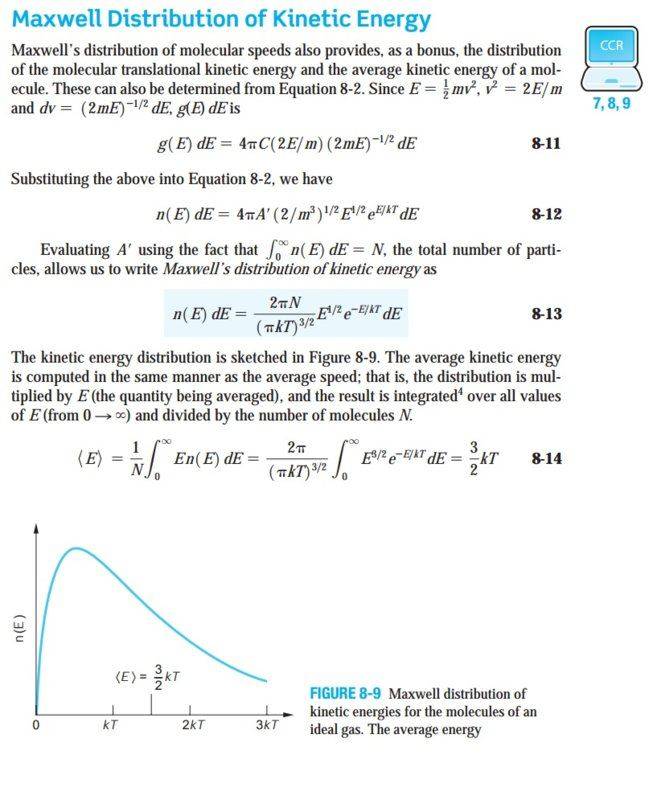
I need some guidance on how to find the fraction of molecules with KE between K1 and K2 from the Maxwell kinetic energy distribution function.
Here's an link to an earlier post where the speed distribution was integrated, how will I proceed with the kinetic energy distribution?
https://www.physicsforums.com/threads/maxwell-boltzmann-distribution.757539/#post-4772356
