- #1
JC2000
- 186
- 16
- Homework Statement
- Not sure how to use latex formatting for the question so I have moved it to the main body ....
- Relevant Equations
- \begin{array}{c}
1 \leq x^{2}+y^{2} \leq e \\
1 \leq x^{2} \leq e \quad 1 \leq y^{2} \leq e \\
1 \leq x \leq \sqrt{e} \quad 1 \leq y \leq \sqrt{e} \\
x=1 \\
x=\sqrt{e}
\end{array}
*Similarly for y which gives a rectangular region in the first quadrant.
Converting to a polar integral : Integrate ##\(f(x, y)=\) \(\left[\ln \left(x^{2}+y^{2}\right)\right] / \sqrt{x^{2}+y^{2}}\)## over the region ##\(1 \leq x^{2}+y^{2} \leq e\)##
So,
\begin{array}{c}
1 \leq x^{2}+y^{2} \leq e \\
1 \leq x^{2} \leq e \quad 1 \leq y^{2} \leq e \\
1 \leq x \leq \sqrt{e} \quad 1 \leq y \leq \sqrt{e} \\
x=1 \\
x=\sqrt{e}
\end{array}
*Similarly for y which gives a rectangular region in the first quadrant.
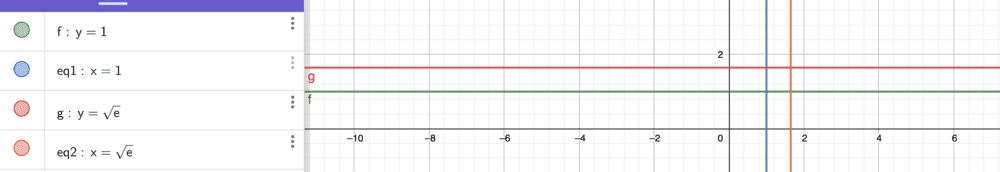
Is the above simplification the correct way to proceed?
So,
\begin{array}{c}
1 \leq x^{2}+y^{2} \leq e \\
1 \leq x^{2} \leq e \quad 1 \leq y^{2} \leq e \\
1 \leq x \leq \sqrt{e} \quad 1 \leq y \leq \sqrt{e} \\
x=1 \\
x=\sqrt{e}
\end{array}
*Similarly for y which gives a rectangular region in the first quadrant.
Is the above simplification the correct way to proceed?
Last edited: