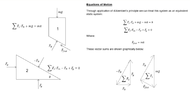
- #1
engineer5lyfe
- 6
- 1
I have seen a few posts on this subject before, but none have really answered my question. For clarity, I will refer to the 1st example as a wedge, and the second as a ramp (although both are of course inclined planes). With both examples that I outline below, we will assume no friction, and a massless wedge/ramp for the sake of simplicity. First, we can begin with the normal force generated from a wedge, for which we know the IMA to be >= 1 provided 45° > theta > 0:
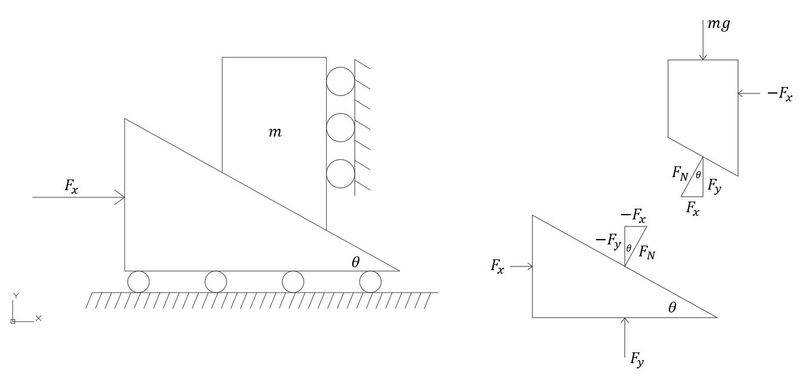
In the case of the wedge, the horizontal force required to maintain equilibrium (F_x) is less than the vertical force applied to the wedge (mg). In this case (static equilibrium), F_y=mg, therefore F_N=mg/cos(theta). Intuitively, this makes sense as we would expect the mechanical advantage of the wedge to be greater than unity for angles < 45°, and therefore F_N should be greater than the input force, F_x.
Now, consider the case in which the block is placed on a ramp. There are two reasonable choices for our reference frames - one in which the x-axis is parallel to the base of the ramp, and one in which the x-axis is parallel to the slope of the ramp. In this case, the choice of reference frame should not affect the description of the normal force. In general, it is more advantageous to choose the latter, which is what we have done below:
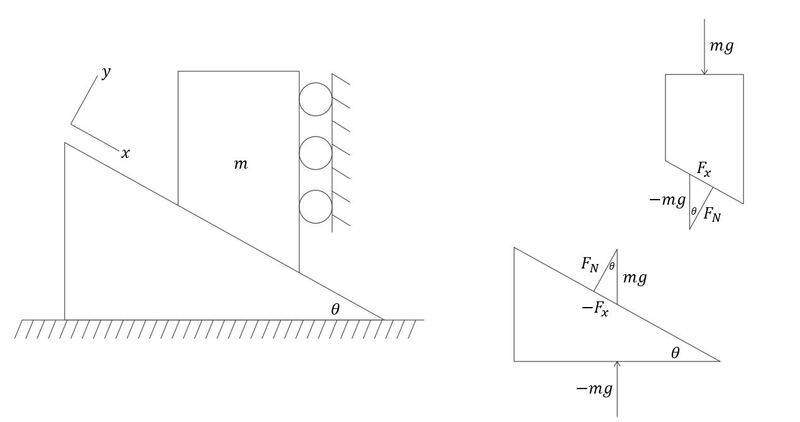
Shown above is the typical force decomposition that is seen for "ramp" problems, which is similar to the diagram from the wikipedia page on inclined planes, shown below:

In the above "ramp" case, the normal force is given as F_N=mg*cos(theta). And this is where my quandary lies - for two similar problems, why do we have different results for the normal force? Which is the correct description of the normal force for both cases?
For the case of the wedge, it makes sense that the normal force is greater than the applied horizontal force, because we are essentially trading distance for force to accomplish the same amount of work (if the wedge was allowed to lift the block).
For the case of the ramp, it also makes sense intuitively that the normal force of the block acting on the ramp and vise-versa is less than m*g. For example, we can look at the extremes of the ramp where theta = 90° and theta=0°. For the case in which theta = 0°, the normal force will be equal to m*g, whereas for the case where theta = 90° the normal force is zero, and the block must either be in free fall, or constrained by some friction, if it exist.
I am trying to build both physical intuition, and concrete mathematical evidence of which case is correct, or why the normal forces actually are different for each case. Any help is greatly appreciated, thank you.
In the case of the wedge, the horizontal force required to maintain equilibrium (F_x) is less than the vertical force applied to the wedge (mg). In this case (static equilibrium), F_y=mg, therefore F_N=mg/cos(theta). Intuitively, this makes sense as we would expect the mechanical advantage of the wedge to be greater than unity for angles < 45°, and therefore F_N should be greater than the input force, F_x.
Now, consider the case in which the block is placed on a ramp. There are two reasonable choices for our reference frames - one in which the x-axis is parallel to the base of the ramp, and one in which the x-axis is parallel to the slope of the ramp. In this case, the choice of reference frame should not affect the description of the normal force. In general, it is more advantageous to choose the latter, which is what we have done below:
Shown above is the typical force decomposition that is seen for "ramp" problems, which is similar to the diagram from the wikipedia page on inclined planes, shown below:
In the above "ramp" case, the normal force is given as F_N=mg*cos(theta). And this is where my quandary lies - for two similar problems, why do we have different results for the normal force? Which is the correct description of the normal force for both cases?
For the case of the wedge, it makes sense that the normal force is greater than the applied horizontal force, because we are essentially trading distance for force to accomplish the same amount of work (if the wedge was allowed to lift the block).
For the case of the ramp, it also makes sense intuitively that the normal force of the block acting on the ramp and vise-versa is less than m*g. For example, we can look at the extremes of the ramp where theta = 90° and theta=0°. For the case in which theta = 0°, the normal force will be equal to m*g, whereas for the case where theta = 90° the normal force is zero, and the block must either be in free fall, or constrained by some friction, if it exist.
I am trying to build both physical intuition, and concrete mathematical evidence of which case is correct, or why the normal forces actually are different for each case. Any help is greatly appreciated, thank you.