- #1
Order
- 97
- 3
Homework Statement
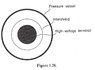
Figure 1.26 (will describe it later on) shows a cross section of a van de Graaf generator, surrounded by an 'intershield' and a pressure vessel, both of which are also cylindrical. The gas in the pressure vessel breaks down in Electric fields greater than 1.6×107 volts/m. If the radii of the terminal, intershield and pressure vessel are a=1.5 m, b=2,5 m and c=4 m respectively, what is the highest potential difference that can be maintained between the terminal and the pressure vessel? (Hint: the intershield must be maintained at a potential such that breakdown is about to occur on its own outer Surface as well as on the Surface of the terminal.)
Homework Equations
[tex] \int_{S} \textbf{E} \cdot d \textbf {S} = \frac{q}{\epsilon_{0}} [/tex]
[tex] \phi (\textbf{r}_{B}) - \phi (\textbf{r}_{A}) = \int_{A}^{B} \textbf{E} \cdot d \textbf{l} [/tex]
The Attempt at a Solution
I evaluate the Surface integral over a length l>>b of the cylinder where i suppose the field will break down. That is I suppose [itex]E_{max}=E_{b}[/itex]
[tex] \int_{S} \textbf{E}_{max} \cdot d \textbf {S} = E_{max} \pi b^{2} l = \frac{q}{\epsilon_{0}}[/tex]
At any Point outside the inner terminal
[tex] \int_{S} \textbf{E} \cdot d \textbf {S} = E(r) \pi r^{2} l = \frac{q}{\epsilon_{0}}[/tex]
combining these two equations i arrive at
[tex] \textbf{E} = E_{max} \frac{b^{2}}{r^{2}} \hat{r} [/tex]
And evaluating the potential
[tex]\Delta \phi = - \int_{a}^{c} E_{max} \frac{b^{2}}{r^{2}} dr = E_{max} b^{2} \left( \frac{1}{c}-\frac{1}{a} \right) = 41 MV [/tex]
But the answer should be 31 MV.
What I don't understand in the hint is that breakdown should occur at two places at once. This must be impossible because the Electric field should be higher Close to the cylinder Before there is breakdown at the intershield (Point b).
ps. sorry that I don't know how my scanner works but I Think the generator i described in the text. ds.

 Much ado about nothing then.
Much ado about nothing then.