- #1
Happiness
- 679
- 30
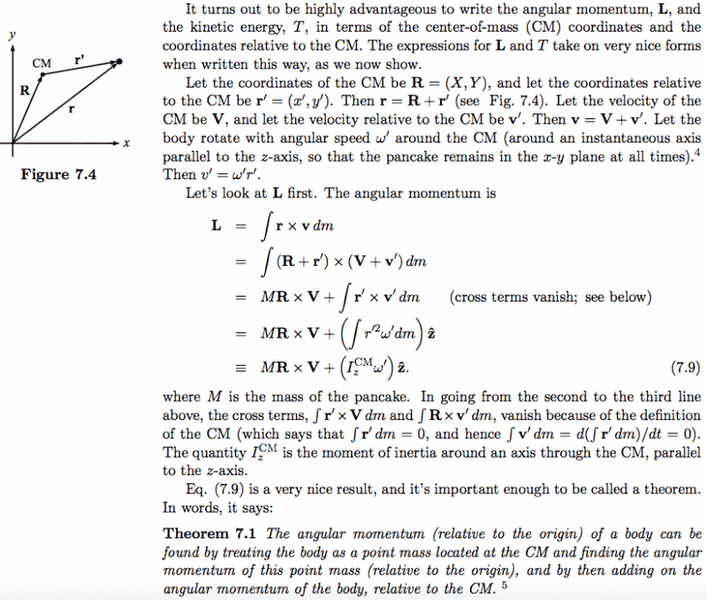
Consider a flat 2D rigid body rotating about an axis perpendicular to the body passing through a point P that is
(1) in the same plane as the body and
(2) different from the body's center of mass (CM).
In this case does Theorem 7.1 (eqn 7.9) still apply?
In the last step of the derivation of (7.9), ##\omega'## is taken out of the integral with respect to ##m##. This is valid as long as each mass element ##dm## has the same angular velocity ##\omega'## with respect to the perpendicular axis passing through the CM. But this is not the case here, since here each mass element ##dm## has the same angular velocity with respect to the perpendicular axis passing through point P.
On the other hand, I'm thinking the theorem may still apply because any rotation about point P (with angular velocity ##\omega##) can be viewed as a superposition of a rotation of the CM about point P (with angular velocity ##\Omega## in a reference frame where P is stationary) and a rotation of the rigid body about the CM (with angular velocity ##\omega'## in a reference frame where the CM is stationary). Is this true? Why? (A rotation about point P means a rotation of the rigid body about the axis perpendicular to the object and passing through point P. EDIT: When I look at this again, I find this useless because ##\omega'=0##. And it only shows the theorem is true if we take the origin/pivot to be at point P but not at a different point Q for the calculation of angular momentum.)
Second question
Suppose a force is applied and then removed such that it causes a rigid body to rotate (with or without the translation of the CM). The object can only rotate about an axis passing through the CM and not any other point. Is it true? Why?
(1) in the same plane as the body and
(2) different from the body's center of mass (CM).
In this case does Theorem 7.1 (eqn 7.9) still apply?
In the last step of the derivation of (7.9), ##\omega'## is taken out of the integral with respect to ##m##. This is valid as long as each mass element ##dm## has the same angular velocity ##\omega'## with respect to the perpendicular axis passing through the CM. But this is not the case here, since here each mass element ##dm## has the same angular velocity with respect to the perpendicular axis passing through point P.
On the other hand, I'm thinking the theorem may still apply because any rotation about point P (with angular velocity ##\omega##) can be viewed as a superposition of a rotation of the CM about point P (with angular velocity ##\Omega## in a reference frame where P is stationary) and a rotation of the rigid body about the CM (with angular velocity ##\omega'## in a reference frame where the CM is stationary). Is this true? Why? (A rotation about point P means a rotation of the rigid body about the axis perpendicular to the object and passing through point P. EDIT: When I look at this again, I find this useless because ##\omega'=0##. And it only shows the theorem is true if we take the origin/pivot to be at point P but not at a different point Q for the calculation of angular momentum.)
Second question
Suppose a force is applied and then removed such that it causes a rigid body to rotate (with or without the translation of the CM). The object can only rotate about an axis passing through the CM and not any other point. Is it true? Why?
Last edited: