- #1
NightHaWk
- 3
- 1
- Homework Statement
- Consider an electric dipole consisting of two electric charges e and −e at a mutual distance 2a.
Consider also a particle of charge e and mass m with an incident wave vector k perpendicular to
the direction of the dipole.
1. Calculate the scattering amplitude in the Born approximation. Find the directions at which
the differential cross section is maximal.
- Relevant Equations
- -
So I am trying to understand and solve the problem mentioned in the title.I found a solution online:
https://physics.bgu.ac.il/COURSES/QuantumMechCohen/ExercisesPool/EXERCISES/ex_9011_sol_Y09.pdf
The problem is, I can't understand this step :
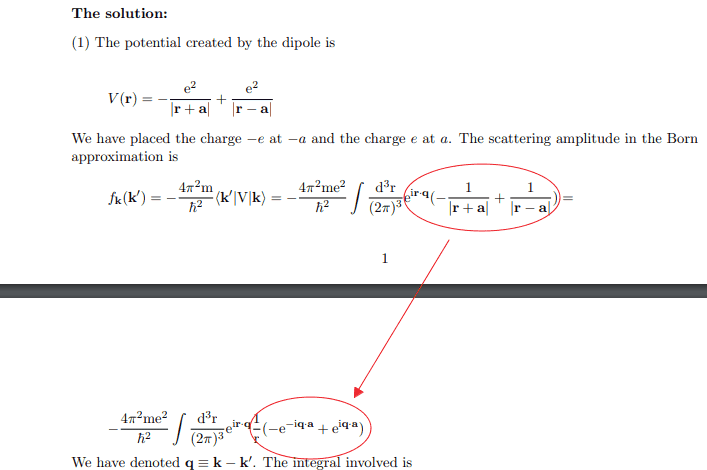
I relly can't find out how the two expontential terms in the parenthesis show up. Any help greatly appreciated.
https://physics.bgu.ac.il/COURSES/QuantumMechCohen/ExercisesPool/EXERCISES/ex_9011_sol_Y09.pdf
The problem is, I can't understand this step :
I relly can't find out how the two expontential terms in the parenthesis show up. Any help greatly appreciated.
Last edited by a moderator: