Visualizing Intrinsic Curvature: Is This a Good Practise?

[MatthijsRog]

TL;DR Summary

Can I visualize intrinsic curvature without resorting to embeddibgs, by drawing a non-exhaustive set of geodesics on my space?

I’m working through the books by Schutz and Renteln to get my differential geometry to the point where I can do general relativity. The author’s have just introduced metrics and notions of parallel transport and with some work I finally understand how intrinsic curvature can be defined by watching parallel transport on loops.

Up until now, when I see authors defining n-dimensional manifolds, they visualize the curvature in such a manifold by embedding it into n+1-dimensional Euclidean space. Now there’s nothing wrong with this, but since doing GR is my goal I felt like visualizing everything as embeddings would be an impairment in the long run.

I figured I could perhaps use another method to visualize a n-dimensional curved space without resorting to embeddings. I figured I could just make a map of my surroundings and then draw a (arbitrary, non-exhaustive) set of geodesics on that map, to express a notion of curvature.

For instance, I could make a badly-drawn map of my bedroom in blue. Drawing geodesics in red, a visualization of a flat metric could be:

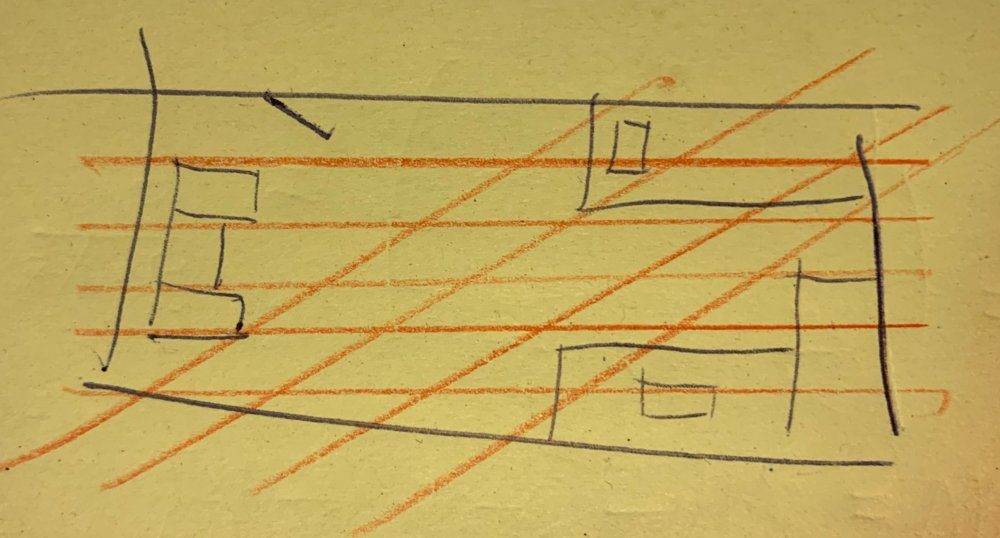
Should my bedroom for some weird, obscure reason become curved, I could depict the curved metric in an embedding, or keep the old blue map and draw new red geodesics on it:

The map isn't unique; I could've used other geodesics or kept the geodesics flat and warped the bedroom itself.

My question is: is this a good visualization method for curved metrics, that won't hurt me in the future? I'm asking this in the G.R. forums because I know there's a lot of bad G.R. visualization around, of which the heavy-ball-and-trampoline visualization is the prime example...I like my diagrams, but I wouldn't want to hurt my own learning curve by learning a bad visualization!

 

[Pencilvester]

If your plan is to visualize curved 2-dimensional spaces by physically drawing them, then drawing geodesics on a set of coordinates would probably be easier than trying to draw a representation of that curved surface in a faux 3-dimensional space.

But if your goal is to get an intuitive grasp on the concepts of GR, it would be better to just work on getting a more intuitive grasp on the mathematics since in GR we’re working with a 4-dimensional pseudo-Riemannian manifold. In certain cases, you might only be interested in looking at a slice of two of those four dimensions, in which case, feel free to draw that however you like.

 

[PeterDonis]

there's a lot of bad G.R. visualization around, of which the heavy-ball-and-trampoline visualization is the prime example

The key problem with visualizations like that is that they are only visualizations of space, not spacetime. Typically an important part of the curvature of a spacetime of interest in GR is in the time direction, so you have to include that direction. As @Pencilvester points out, spacetime in general is four dimensional and we can't draw or visualize four dimensional geometric figures, so we have to eliminate some dimensions; but often there will only be two (or sometimes three) dimensions of actual interest (of which time, as above, will be one), so you can actually draw diagrams.

As for drawing "grid lines" on a flat sheet of paper vs. trying to visualize an appropriate curved surface, I think both approaches can be useful, and whichever one works best for you should generally be fine.

 

[pervect]

I think the diagram needs a bit of math to aid in interpreting its meaning and to explain how it demonstrates curvature.

My thoughts on this is that one might refer to the geodesic deviation equation, which says that the relative acceleration between geodesics is

$$A^a = R^a{}_{bcd} u^b x^c u^d$$

Here, $A^a$ is the relative acceleration between geodesics, $u^b$ is the tangent vector to one geodesic (the 4-velocity in the space-time case), $u^d$ is the tangent vector to the other geodesic, and $x^c$ is the separation vector.

This isn't a complete discussion of the geodesic deviation equation by any means, just a quick summary.

Then the point of this is that in flat spaces, geodesics do not accelerate away from each other, while in curved spaces, they do. So if all the relative accelerations $A^a$ are zero, then the Riemann tensor is zero, and thus we have no curvature.

Your focus at the moment seems to be understanding differential geometry, which is OK. At some point, in moving to GR, one talks about a 4 dimensional space-time rather than a 3 dimensional space, and one replaces the notion of "distance" with the invariant Lorentz interval. Your technique might help you visualize the meaning of a curved three dimensional spaces without using embeddding diagrams, but it will still be difficult to use it to illustrate curved 4-dimensonal space-times, which is where you want to end up at to understand GR. I won't say that it's impossible to visualize 4 dimensional objects, one might use color shading on a diagram to "visualize" the fourth dimension, but it won't be terribly easy. At some point, the abstract methods take over. Basically, your approach does get rid of embedding diagrams, and it does allow you to go from the 2d case to the 3d case, but it won't easily go to the 4d case.

 

[MatthijsRog]

Thank you all very much for your comments. Thanks for the warning about the switch to 4D as well. I don’t think it’ll be massive impairment. Didactically, visualising lower-dimensional cases (working with projections, multivariable functions, etc) is usually a standard part of the roadmap to understanding the abstract, more complicated case.

Would a good summary be that for the two-dimensional cases (and only locally I suppose, the sphere doesn’t fit on there) my diagrams suffice, though they’re a tad simplistic? Then at least I’ll know I’m on the right track. ^^

 

[A.T.]

I figured I could perhaps use another method to visualize a n-dimensional curved space without resorting to embeddings. I figured I could just make a map of my surroundings and then draw a (arbitrary, non-exhaustive) set of geodesics on that map, to express a notion of curvature.

The problem is choosing that set of geodesics in a consistent way, that makes different cases comparable.

An idea:
1) Define a rectangular grid.
2) At each grid node find the geodesic that has the maximal local curvature (in the projection), and draw just a short piece of that geodesic. Optionally a piece of the geodesic perpendicular to that or with the least local projection curvature. If curvature is zero just draw a grid cross or the node as a point

 

[MatthijsRog]

An idea:
1) Define a rectangular grid.
2) At each grid node find the geodesic that has the maximal local curvature (in the projection), and draw just a short piece of that geodesic. Optionally a piece of the geodesic perpendicular to that or with the least local projection curvature. If curvature is zero just draw a grid cross or the node as a point

Sounds like a great improvement! You’re right that the current sketch is not really comparable at all; it just shows the shape of some arbitrary geodesics. This shouldn’t be completely “wrong” but it could potentially confuse the viewer of such a sketch.