What exactly is the reactive centrifugal force (split)

[A.T.]

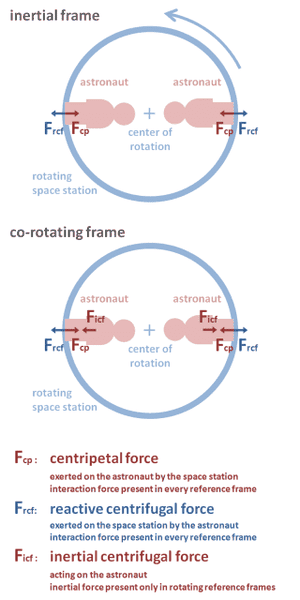
Mod note: This thread on the reactive centrifugal force was split from [thread=667584]this thread[/thread] on the (fictitious) centrifugal force.

Using the term 'Centrifugal Force' when I was at school was verboten.

It is really not that confusing if you make clear which reference frame you consider.

 

[Andrew Mason]

It is really not that confusing if you make clear which reference frame you consider.

There is only one force on each astronaut provided by the space station. That is the centripetal force provided by the tensions in space station structure. Each astronaut provides a force on the space station that creates tensions within the space station structure and result in a centripetal force on the other astronaut.

So one could say that the reaction force to the centripetal force on one astronaut is that astronaut's (centripetal) force on the other astronaut arising from the structural tensions within the space station structure. In that sense, all the forces are centripetal.

AM

 

[A.T.]

Each astronaut provides a force on the space station...

Which points outwards, away from the center of rotation. That's why it is called "centrifugal".

that creates tensions within the space station structure and result in a centripetal force on the other astronaut.

Irrelevant. There is a direct local interaction between astronaut and station with two equal/opposite forces: one is centripetal, one centrifugal.

So one could say that the reaction force to the centripetal force on one astronaut is that astronaut's (centripetal) force on the other astronaut arising from the structural tensions within the space station structure.

No, this is not how Newtons 3rd Law is applied. There is no direct interaction between the two astronauts. So there is no 3rd Law force pair acting on both.

In that sense, all the forces are centripetal.

Then "that sense" is obviously nonsense. Everyone can see that F_rcf is pointing outwards, away from the center of rotation. So it is centrifugal.

 

[Andrew Mason]

Which points outwards, away from the center of rotation. That's why it is called "centrifugal".

It depends on how you look at it. The force applied by astronaut1 causes astronaut2, who is mechanically connected to the space station, to accelerate toward the centre of the station. If astronaut1 stopped applying this force, astronaut2's acceleration (and the acceleration of the rest of the space station) toward the centre of the space station would decrease. So if you want to associate the direction of a force with the acceleration that it causes, the direction of the astronaut's force is centripetal.

No, this is not how Newtons 3rd Law is applied. There is no direct interaction between the two astronauts. So there is no 3rd Law force pair acting on both.


Then "that sense" is obviously nonsense. Everyone can see that F_rcf is pointing outwards, away from the center of rotation. So it is centrifugal.

Suppose we have two spherical moons orbiting a spherical planet and both moons are directly opposite each other (ie. a line through the moons' centres passes through the planet's centre) on identical orbits. Would you say that that the reaction forces of each moon on the planet are centrifugal?

AM

 

[sophiecentaur]

You guys are going to have to agree to differ. The 'other guy' is not actually wrong, in this case. Can't you both see that?

 

[A.T.]

...causes ...acceleration

Irrelevant. The term "centrifugal" has nothing to do with causing acceleration. It simply means that the force points away from the center of rotation. Acceleration is a matter of net force, not just one centrifugal force. So a force can be centrifugal without causing centrifugal acceleration.

So if you want to associate the direction of a force with the acceleration that it causes,

I don't. For the reason I state above.

Suppose...

No thanks. I gave an example where a centrifugal reaction force exists. Pointing out other cases where there is no centrifugal reaction force proves nothing. Nobody claimed it is always there.

 

[Andrew Mason]

I don't see how you can confuse the two. One is an interaction force that exists in every frame, the other is an inertial force that exist only in rotating frames. The diferences are listed in the table here:
http://en.wikipedia.org/wiki/Reactive_centrifugal_force#Relation_to_inertial_centrifugal_force

Calling it "centripetal" as you suggest, despite the fact that it points away from the center, that would be confusing.

We are not in disagreement over the direction of the reaction force. We disagree on the body that is experiencing the reaction. Whether a "reaction" points toward or away from the centre depends on the location of the body experiencing the reaction.

Newton's third law does not talk about tensions. Newton talks about "actions" and "reactions which mean "changes in motion". The third law says "To every action there is always opposed an equal reaction; or the mutual actions of two bodies upon each other are always equal, and directed to contrary parts." In other words, changes in motion cannot occur in just one body. The change in motion (momentum) of one body requires an opposing change in motion of another body. The "reaction" to any "action" is an equal and opposing change in momentum of another.

AM

 

[stevendaryl]

Newton's third law does not talk about tensions. Newton talks about "actions" and "reactions which mean "changes in motion". The third law says "To every action there is always opposed an equal reaction; or the mutual actions of two bodies upon each other are always equal, and directed to contrary parts." In other words, changes in motion cannot occur in just one body. The change in motion (momentum) of one body requires an opposing change in motion of another body. The "reaction" to any "action" is an equal and opposing change in momentum of another.

AM

Hmm. I guess I can see what you're saying, but the way that I've seen Newton's third law used in actual problems was in terms of equal and opposite forces rather than momentum changes. In practice, it worked something like this:

Let $\stackrel{\rightarrow}{F_I}$ be the force on object $I$. Then, if the only forces are two-body forces (which is the case for many problems), we can write:

$\stackrel{\rightarrow}{F_I} = \sum_J \stackrel{\rightarrow}{F_{IJ}}$ where $\stackrel{\rightarrow}{F_{IJ}}$ is the force on object $I$ due to object $J$.

The assumption of equal and opposite forces is just the assumption that

$\stackrel{\rightarrow}{F_{IJ}} = - \stackrel{\rightarrow}{F_{JI}}$

Ultimately, it's certainly true that changes of momenta have to cancel, because the total momentum can't change due to internal forces. However, it seems to me that accounting for the changes in momenta is more systematic by using pairwise forces, and using the principle of equal and opposite forces.

 

[Andrew Mason]

Hmm. I guess I can see what you're saying, but the way that I've seen Newton's third law used in actual problems was in terms of equal and opposite forces rather than momentum changes.

The forces and changes in momentum are necessarily directly proportional so changes in momentum must be equal and opposite.

Since, in non-relativistic interactions, the duration of interaction is the same for both interacting bodies, the changes in momentum of the interacting bodies are equal and opposite: Body 1: Force exerted by body 1 on body 2 = F1 = dp2/dt ⇔∫F1dt = ∫dp2 = Δp2; For body 2: F2 = - F1 ⇔ ∫F2dt = Δp1 = -∫F1dt = -Δp2

AM

 

[Dale]

The "reaction" to any "action" is an equal and opposing change in momentum of another.

Nonsense. The change in momentum is a net force. It is the individual forces which have action reaction pairs.

Consider a person standing on the ground. There is a gravitational force down and a normal force up, the net force is zero and therefore there is no change in momentum. So according to you there is no normal reaction force on the ground and also no gravitational reaction force since there is no change in momentum.

This is NOT how Newtons third law works. It is the individual forces which are in action reaction pairs, not the net force.

 

[Dale]

The forces and changes in momentum are necessarily directly proportional.

No, they are not. Standing on the ground, my change in momentum is zero, but the force of the ground on my feet is nonzero, as is the reaction force of my feet on the ground.

Your statement is a misrepresentation of Newtons third law.

 

[stevendaryl]

The forces and changes in momentum are necessarily directly proportional so changes in momentum must be equal and opposite.

Since, in non-relativistic interactions, the duration of interaction is the same for both interacting bodies, the changes in momentum of the interacting bodies are equal and opposite: Body 1: Force exerted by body 1 on body 2 = F1 = dp2/dt ⇔∫F1dt = ∫dp2 = Δp2; For body 2: F2 = - F1 ⇔ ∫F2dt = Δp1 = -∫F1dt = -Δp2

AM

But in general, there are more than two objects. So suppose we have three objects, $O_1, O_2$ and $O_3$, with $O_2$ in the center. Object $O_1$ exerts a force only on $O_2$, and $O_2$ exerts forces on $O_1$ and $O_3$. Object $O_3$ exerts a force only on $O_2$

In this setup, it's not the case that the change in momentum of $O_1$ is equal and opposite to the change in momentum of $O_2$, because $O_1$ is not the only object acting on $O_2$.

It's certainly true that the total change in momentum is zero, if you add up the changes for all three objects. But they don't come in action/reaction pairs. To me, what comes in pairs is "the force on $O_1$ due to $O_2$" and "the force on $O_2$ due to $O_1$"

 

[A.T.]

Indirectly, it is.

No, it's not affecting the prediction. As you said yourself, it is just rearranging the initial equation and naming some of the terms. To get a prediction you have to rearrange equation anyway to solve for certain terms, and the solutions are mathematically equivalent.

Come on. You know what the word "understand" means.

I know what it means to me in the context of physics: Understanding the world = Having a model of the world that can make quantitative predictions. But you seem to have a different definition of "understanding the world" in the context of physics, because you see a difference between the two.

I also don't understand what you mean by the gap between understanding and truth here:

Claiming that the goal of physics is understanding doesn't imply how close our current understanding is to the truth.

To me, in the context of physics, this would mean the gap between quantitative prediction and measurement. But since to you understanding is not about quantitative prediction, I have no idea what truth could mean here.

 

[A.T.]

We are not in disagreement over the direction of the reaction force.

You claim that all forces in my scenario are centripetal. That would include F_rcf which is obviously centrifugal.

Newton talks about "actions" and "reactions which mean "changes in motion".

Wrong. Action & reaction are forces, not changes in motion. Newton 3rd Law applies to static cases as well, where there are force pairs but no acceleration.

 

[stevendaryl]

No, it's not affecting the prediction. As you said yourself, it is just rearranging the initial equation and naming some of the terms. To get a prediction you have to rearrange equation anyway to solve for certain terms, and the solutions are mathematically equivalent.

AT, one of the first posts you made to this thread, you said:

"Fictitious force" is just an unfortunate name choice. It gives people the wrong idea that some forces are more "real" than others. But that is just philosophy, irrelevant to physics. That is why I prefer the terms "inertial forces" and "interaction forces".

So, way back then, you seem to agree that a choice of terminology can be "unfortunate" and that it can give people the "wrong idea", which I assume means that it can be misleading. Have you changed your mind about the possibility of terminology being unfortunate and misleading?

If you still believe in the possibility that terminology can be unfortunate and can give people the wrong idea, then why do you object so strenuously when I suggest that YOUR terminology can be unfortunate and can give people the wrong idea?

 

[stevendaryl]

I know what it means to me in the context of physics: Understanding the world = Having a model of the world that can make quantitative predictions. But you seem to have a different definition of "understanding the world" in the context of physics, because you see a difference between the two.

They are certainly two different activities: Constructing, refining, simplifying, clarifying concepts of models is one activity. Comparing a model's predictions with experiment is another activity. "Understanding" can apply to either a model, or to experimental results.

As I pointed out, one of your first posts made complaints about what you considered a bad way to think about models, when you said that the phrase "fictitious forces" is unfortunate, and can give people the wrong idea.

 

[A.T.]

So, way back then, you seem to agree that a choice of terminology can be "unfortunate" and that it can give people the "wrong idea", which I assume means that it can be misleading. Have you changed your mind about the possibility of terminology being unfortunate and misleading?

I admit that "wrong idea" is not the best way to put it. As I explain later in the post you mentioned, I meant that it leads to pointless philosophizing about what is "real", which is irrelevant to physics.

If you still believe in the possibility that terminology can be unfortunate and can give people the wrong idea, then why do you object so strenuously when I suggest that YOUR terminology can be unfortunate and can give people the wrong idea?

Because your justification for calling a certain terminology unfortunate, is exactly the pointless philosophizing about what is "real" or "the truth", which I don't see as relevant.

 

[stevendaryl]

I admit that "wrong idea" is not the best way to put it. As I explain later in the post you mentioned, I meant that it leads to pointless philosophizing about what is "real", which is irrelevant to physics.

In this particular case, we can certainly make the distinction between vectors, whose components are covariant under coordinate transformations, and terms that can be made to vanish by choosing a particular coordinate system. That's a mathematical, not a philosophical distinction, and it's an important distinction for working with the equations of motion.

 

[Dale]

So, way back then, you seem to agree that a choice of terminology can be "unfortunate" and that it can give people the "wrong idea", which I assume means that it can be misleading. Have you changed your mind about the possibility of terminology being unfortunate and misleading?

I actually think that this is an excellent example. I too think that the term "fictitious force" is unfortunate. Nonetheless, I recognize that it isn't up to me and that people who use it in the standard way are making correct statements from an authoritative definition. Similarly, you should realize that the definition of "physics", although you think it is unfortunate, it is not up to you and that people who use it in the standard way are making correct statements from an authoritative definition.

If I were having your argument with A.T. I would either reference a definition of physics that included the philosophical bits you like or I would challenge him to reference a definition of physics that excluded them. Many times terms have multiple authoritative definitions, but that doesn't mean that it is good practice to simply go about redefining them yourself, since nobody is likely to consider your definition authoritative.

 

[stevendaryl]

I actually think that this is an excellent example. I too think that the term "fictitious force" is unfortunate. Nonetheless, I recognize that it isn't up to me and that people who use it in the standard way are making correct statements from an authoritative definition. Similarly, you should realize that the definition of "physics", although you think it is unfortunate, it is not up to you and that people who use it in the standard way are making correct statements from an authoritative definition.

I disagree. There is nothing sacred about terminology, other than the fact that if one is using a technical term that is not standard, then there is time that must be spent explaining the terminology that could possibly be better spent on something else.

When it comes to technical terminology, such as "fictitious force" versus "inertial force" versus what I consider better "connection coefficients", different authors use different terminology. There is no absolute authority about what terms to use.

As for the definition of "physics" itself, I can't see any point whatsoever in worrying about it, or caring what the official definition is. A particular journal can decide to accept or reject a paper based on whatever criteria they choose; it doesn't need to be a universal definition of what counts as "physics".

 

[Andrew Mason]

Nonsense. The change in momentum is a net force. It is the individual forces which have action reaction pairs.

Consider a person standing on the ground. There is a gravitational force down and a normal force up, the net force is zero and therefore there is no change in momentum. So according to you there is no normal reaction force on the ground and also no gravitational reaction force since there is no change in momentum.

This is not a good example because the gravitational force and normal force are not third law pairs. They are not equal and opposite, for one thing. The normal force is always a bit less than the gravitational force except at the poles.

The third law pair to the gravitational force of the Earth on a body is necessarily the gravitational force of the body on the earth. Gravitational force supplies the centripetal acceleration required of the body on the surface of the earth. The body supplies the Earth with its centripetal acceleration about the body-earth centre of mass (which is obviously extremely close to the Earth's centre of mass).

This is NOT how Newtons third law works. It is the individual forces which are in action reaction pairs, not the net force.

I disagree. Your example illustrates why it can be difficult to apply Newton's third law. Where there is a body experiencing a change in motion there is always some other body experiencing an equal and opposite change in motion. Those are the third law pairs.

AM

 

[A.T.]

In this particular case, we can certainly make the distinction between vectors, whose components are covariant under coordinate transformations, and terms that can be made to vanish by choosing a particular coordinate system.

Who said something against making a distinction? I said that I prefer the terms "inertial forces" and "interaction forces". These are two distinct names.

Also note that I used the word "prefer", clearly indicating that it is just personal preference. Contrary to you, who is using terms like "falsehood", "crippled physics" etc. to denounce a naming convention which you don't like.

 

[A.T.]

The third law pair to the gravitational force of the Earth on a body is necessarily the gravitational force of the body on the earth. Gravitational force supplies the centripetal acceleration required of the body on the surface of the earth. The body supplies the Earth with its centripetal acceleration about the body-earth centre of mass (which is obviously extremely close to the Earth's centre of mass).

What acceleration? Consider a non-rotating planet with you on it. Newtonian gravity. There is no acceleration. It is all static. But there is one interaction (electro-magnetic) between your feet and the ground, with a 3rd law force pair. And there is a second interaction (gravitational) between you and the planet, with a second 3rd law force pair. Two times action & reaction without any change in motion.

Where there is a body experiencing a change in motion there is always some other body experiencing an equal and opposite change in motion. Those are the third law pairs.

Nope, the third law pairs are individual forces, not accelerations.
http://en.wikipedia.org/wiki/Newton's_laws_of_motion

Third law: When a first body exerts a force F1 on a second body, the second body simultaneously exerts a force F2 = −F1 on the first body.

Your problem is as usual: You think that the most trivial and primitive example (two bodies, one interaction) represents the general case. So you fail to apply the laws correctly to an even slightly more complex scenario (like the one above, with two interactions).

 

[Andrew Mason]

You claim that all forces in my scenario are centripetal. That would include F_rcf which is obviously centrifugal.

It depends on where the force acts. If one says that a force acts on the centre of mass of the body (ie. the space station), then the force is centripetal because it causes the space station and other astronaut (as the other body) to prescribe a centripetal acceleration towards the centre of rotation.

Wrong. Action & reaction are forces, not changes in motion. Newton 3rd Law applies to static cases as well, where there are force pairs but no acceleration.

It is not wrong. It is just a way of looking at the third law that differs slightly, but not fundamentally, from your view.

The fact is that Newton refers to "forces" in his first and second laws. He does not use the word "force" in the third law.

Your view, I gather, is that his omission of the word "force" and his use of "action" and "reaction" were not deliberate. My view is that this was deliberate.

The third law can be viewed as the law of interacting bodies. If there is a change in motion of a body there must be another equal and opposite change in motion of some other interacting body or bodies. That, it seems to me, is the very essence of the third law. If there are forces but no actions (changes in motion), there is no need for the third law. The first law covers the situation.

AM

 

[stevendaryl]

Who said something against making a distinction? I said that I prefer the terms "inertial forces" and "interaction forces". These are two distinct names.

Sure, but "connection coefficient" is what they are, mathematically, and has the advantage of having a precise definition saying how to compute them. Connection coefficients are needed whenever one needs to take a derivative of a vector or tensor field. Calling these terms "fictitious forces" doesn't make sense, because not all uses of derivatives have anything to do with forces. They really don't have anything to do with forces, in general.

 

[Dale]

the gravitational force and normal force are not third law pairs.

I never said they were. They act on the same body so they cannot be third law pairs. Each of those two forces (gravitational and normal) have their own third law reaction forces acting on the earth.

The normal force is always a bit less than the gravitational force except at the poles.

Fine. Consider the person to be standing on a turntable at the pole such that he is at rest in a Newtonian inertial frame. And consider the planet to be isolated far from any significant gravitational body.

The third law pair to the gravitational force of the Earth on a body is necessarily the gravitational force of the body on the earth.

Yes, this is correct. Note that the gravitational force (mg) is not equal to the change in momentum (ma=0). So your correct statement here contradicts your own incorrect statement elsewhere.

Where there is a body experiencing a change in motion there is always some other body experiencing an equal and opposite change in motion. Those are the third law pairs.

Not only is this not how Newtons third law works, it is not even true.

Consider three identical masses, each connected by identical springs of neglible mass to both of the others and starting at rest from a position where the springs are all equally stretched. Each object is experiencing a change in motion and there is no other body experiencing an equal and opposite change in motion.

 

[A.T.]

It depends on where the force acts.

Look at the picture again. Try to figure out where the blue arrows start. That's where the astronaut applies the force to the space station.

If one says that a force acts on the centre of mass of the body...

...then one obviously has a serious case of cognitive dissonance.

The fact is that Newton refers to "forces" in his first and second laws. He does not use the word "force" in the third law.

Doesn't matter. In today's physics the 3rd law refers to forces. Every physics book will confirm this.

 

[Andrew Mason]

What acceleration? Consider a non-rotating planet with you on it. Newtonian gravity. There is no acceleration. It is all static. But there is one interaction (electro-magnetic) between your feet and the ground, with a 3rd law force pair. And there is a second interaction (gravitational) between you and the planet, with a second 3rd law force pair. Two times action & reaction without any change in motion.

I agree. Those are the forces and the interactions. They all sum to 0. But you don't need the third law to analyse that. There is no "action" (ignoring the acceleration relative to the sun and other planets etc.) . Newton made the distinction between force and action. I didn't.

Nope, the third law pairs are individual forces, not accelerations.
http://en.wikipedia.org/wiki/Newton's_laws_of_motion

Wikipedia does not state Newton's the third law. It states one interpretation of Newton's third law but it does not use the terms "action" and "reaction" that Newton used. In my view, it implicitly combines the first, second and third laws of Newton so it makes it difficult to see how the third law is distinct from the first and second. If forces are balanced so there is no net force, the first law applies. You don't need the third law to determine that if a body's motion is not changing all the forces must sum to 0. You just need the first.

Your problem is as usual: You think that the most trivial and primitive example (two bodies, one interaction) represents the general case. So you fail to apply the laws correctly to an even slightly more complex scenario (like the one above, with two interactions).

I am not disagreeing with you on the physics. All I am saying is that if there is no acceleration, you don't need the third law. The first law suffices.

An approach that says that people who take a valid but different view of things are wrong is not particularly helpful.

AM

 

[stevendaryl]

Consider three identical masses, each connected by identical springs of neglible mass to both of the others and starting at rest from a position where the springs are all equally stretched. Each object is experiencing a change in motion and there is no other body experiencing an equal and opposite change in motion.

I agree with you that thinking of equal and opposite forces works better than thinking of equal and opposite changes of motion, but in the case you are talking about, the change in momentum of one of the masses is equal and opposite to the vectorial sum of the changes of momentum of the other two masses. That might be what AM meant.

 

[A.T.]

Wikipedia does not state Newton's the third law. It states one interpretation of Newton's third

It is the interpretation of Newton's third that you will find in every modern physics book. It doesn't matter how you call this Law ("3rd law" or "generalized 3rd law"). All your hand waving cannot change the fact that:
- It is a law of physics.
- It states two equal but opposite forces.
- It applies to static cases too.
- It means that my astronauts exert a centrifugal reaction force on the station

 

[stevendaryl]

I am not disagreeing with you on the physics. All I am saying is that if there is no acceleration, you don't need the third law. The first law suffices.

Hmm. Let's see if that's true. Suppose you have three objects 1, 2, 3. There are correspondingly 6 forces:

1. $\stackrel{\rightarrow}{F_{12}}$ = force of 1 on 2
2. $\stackrel{\rightarrow}{F_{21}}$ = force of 2 on 1
3. $\stackrel{\rightarrow}{F_{13}}$ = force of 1 on 3
4. $\stackrel{\rightarrow}{F_{31}}$ = force of 3 on 1
5. $\stackrel{\rightarrow}{F_{23}}$ = force of 2 on 3
6. $\stackrel{\rightarrow}{F_{32}}$ = force of 3 on 2

The fact that the situation is static means that the force on any object is zero. So we have:

1. $\stackrel{\rightarrow}{F_{12}} + \stackrel{\rightarrow}{F_{32}} = 0$
2. $\stackrel{\rightarrow}{F_{13}} + \stackrel{\rightarrow}{F_{23}} = 0$
3. $\stackrel{\rightarrow}{F_{21}} + \stackrel{\rightarrow}{F_{31}} = 0$

That's three constraints. I don't see how you can derive that
$\stackrel{\rightarrow}{F_{12}} + \stackrel{\rightarrow}{F_{21}} = 0$

 

[Dale]

Andrew Mason, please provide a mainstream scientific reference for Newton's 3rd law being as you describe, equal and opposite net forces or equal and opposite changes in momentum.

 

[Dale]

Hmm. Let's see if that's true. Suppose you have three objects 1, 2, 3. There are correspondingly 6 forces:

1. $\stackrel{\rightarrow}{F_{12}}$ = force of 1 on 2
2. $\stackrel{\rightarrow}{F_{21}}$ = force of 2 on 1
3. $\stackrel{\rightarrow}{F_{13}}$ = force of 1 on 3
4. $\stackrel{\rightarrow}{F_{31}}$ = force of 3 on 1
5. $\stackrel{\rightarrow}{F_{23}}$ = force of 2 on 3
6. $\stackrel{\rightarrow}{F_{32}}$ = force of 3 on 2

The fact that the situation is static means that the force on any object is zero. So we have:

1. $\stackrel{\rightarrow}{F_{12}} + \stackrel{\rightarrow}{F_{32}} = 0$
2. $\stackrel{\rightarrow}{F_{13}} + \stackrel{\rightarrow}{F_{23}} = 0$
3. $\stackrel{\rightarrow}{F_{21}} + \stackrel{\rightarrow}{F_{31}} = 0$

That's three constraints. I don't see how you can derive that
$\stackrel{\rightarrow}{F_{12}} + \stackrel{\rightarrow}{F_{21}} = 0$

In fact, you cannot derive it. Here are a set of forces that satisfy Newton's 1st law but not his 3rd:

1. $\stackrel{\rightarrow}{F_{12}}=2$
2. $\stackrel{\rightarrow}{F_{21}}=1$
3. $\stackrel{\rightarrow}{F_{13}}=3$
4. $\stackrel{\rightarrow}{F_{31}}=-1$
5. $\stackrel{\rightarrow}{F_{23}}=-3$
6. $\stackrel{\rightarrow}{F_{32}}=-2$

 

[Paul Andersen]

I think that there is no such thing as centrifugal force .

Am I right ? is this force fictitious ?

Yes.
You can study the centrifugal and Coriolis forces here:
http://www.gethome.no/paulba/Spaceship.html

Hit the "slow motion" button.

 

[Andrew Mason]

But in general, there are more than two objects. So suppose we have three objects, $O_1, O_2$ and $O_3$, with $O_2$ in the center. Object $O_1$ exerts a force only on $O_2$, and $O_2$ exerts forces on $O_1$ and $O_3$. Object $O_3$ exerts a force only on $O_2$

In this setup, it's not the case that the change in momentum of $O_1$ is equal and opposite to the change in momentum of $O_2$, because $O_1$ is not the only object acting on $O_2$.

It's certainly true that the total change in momentum is zero, if you add up the changes for all three objects. But they don't come in action/reaction pairs. To me, what comes in pairs is "the force on $O_1$ due to $O_2$" and "the force on $O_2$ due to $O_1$"

If there are three bodies interacting, it is much more difficult to analyse forces because the duration of the interactions between bodies may differ. What one can easily determine, however, are the changes in motion that result from the interactions. For three interacting bodies, A, B and C, if body A experiences a change in motion (momentum), bodies B and C must experience a change in motion that, combined, is equal in magnitude to the change in A's motion but opposite in direction.

AM