Why can't we define an eigenvalue of a matrix as any scalar value?

[ChiralSuperfields]

Homework Statement

Please see below

Relevant Equations

Please see below

For this,

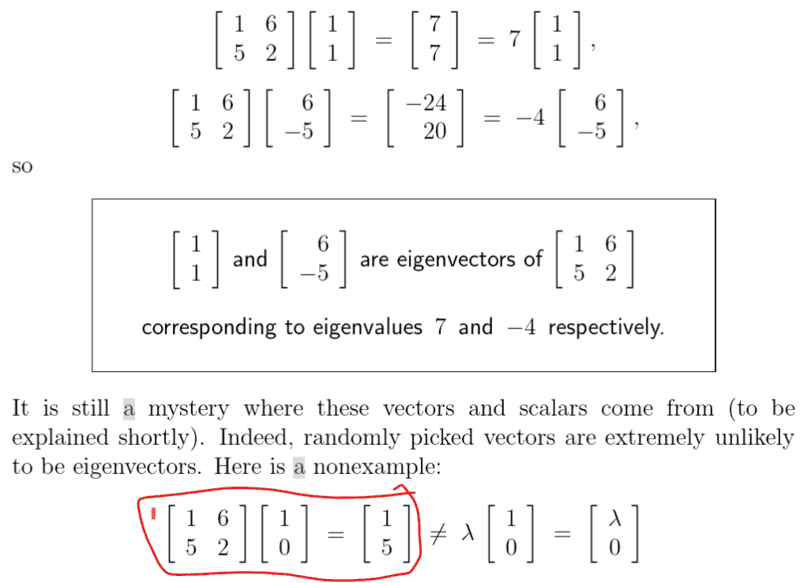
Dose anybody please know why we cannot say $\lambda = 1$ and then $1$ would be the eigenvalue of the matrix?

Many thanks!

 

[FactChecker]

The result of the multiplication is $\begin{bmatrix} 1 \\ 5 \end{bmatrix}$, not $\begin{bmatrix} \lambda \\ 0 \end{bmatrix}$, so it doesn't matter what the value of $\lambda$ is.

 

[Mark44]

Dose anybody please know why we cannot say λ=1 and then 1 would be the eigenvalue of the matrix?

"Dose" -- an amount of medicine.
"Does" -- third person singular conjugation of the infinitive verb "to do."

An eigenvalue $\lambda$ is a number such that for an eigenvector x, $A\mathbf x = \lambda \mathbf x$.

For the matrix you asked about $\begin{bmatrix}1 & 6 \\ 5 & 2\end{bmatrix} \begin{bmatrix}1 \\ 0 \end{bmatrix} = \begin{bmatrix}1 \\5 \end{bmatrix} \ne \lambda \begin{bmatrix}1 \\ 0 \end{bmatrix}$ for any value of $\lambda$.

 

[ChiralSuperfields]

Thank you for your replies @FactChecker and @Mark44!

Sorry I still don't I understand. I'll try to explain what my understanding is so that any misconception can be exposed. $\lambda$ is the constant in front that is factor out of the column vector $\vec x$ which is called the eigenvalue. For examples 1 and 2 below, the constant multiplied to the column vector is $\lambda = 7 , -4$ respectively.

However, for this example,

$\begin{bmatrix}1 & 6 \\ 5 & 2\end{bmatrix} \begin{bmatrix}1 \\ 0 \end{bmatrix} = \begin{bmatrix}1 \\5 \end{bmatrix}$, why can't we factor out a 1 from the column vector to get $\begin{bmatrix}1 & 6 \\ 5 & 2\end{bmatrix} \begin{bmatrix}1 \\ 0 \end{bmatrix} = 1 \begin{bmatrix}1 \\5 \end{bmatrix}$.

According to the textbook, $\lambda$ can be any real number, so why can't $1$ be an eigenvalue?

Many thanks!

 

[Mark44]

An eigenvalue $\lambda$ is a number such that for an eigenvector x, $A\mathbf x = \lambda \mathbf x$.

You didn't read what I wrote in my previous post carefully enough. An eigenvalue is closely associated with a specific eigenvector. In the equation above, x is an eigenvector that appears on both sides of the equation. For an eigenvalue/eigenvector pair, multiplication of the vector by the matrix produces a value that is a scalar multiple (i.e., the eigenvalue) of that same vector.

However, for this example,
$\begin{bmatrix}1 & 6 \\ 5 & 2\end{bmatrix} \begin{bmatrix}1 \\ 0 \end{bmatrix} = \begin{bmatrix}1 \\5 \end{bmatrix}$, why can't we factor out a 1 from the column vector to get $\begin{bmatrix}1 & 6 \\ 5 & 2\end{bmatrix} \begin{bmatrix}1 \\ 0 \end{bmatrix} = 1 \begin{bmatrix}1 \\5 \end{bmatrix}$.

Because $\begin{bmatrix}1 \\ 0 \end{bmatrix}$ isn't the vector that appears on both sides of the equation.

 

[ChiralSuperfields]

You didn't read what I wrote in my previous post carefully enough. An eigenvalue is closely associated with a specific eigenvector. In the equation above, x is an eigenvector that appears on both sides of the equation. For an eigenvalue/eigenvector pair, multiplication of the vector by the matrix produces a value that is a scalar multiple (i.e., the eigenvalue) of that same vector.

Because $\begin{bmatrix}1 \\ 0 \end{bmatrix}$ isn't the vector that appears on both sides of the equation.

Oh, thank you @Mark44! I see now. Sorry I forgot that the column vector has to be on both sides.