- #1
SahinTC
- 19
- 0
Let's make it simple and suppose you have a very, very, perfectly sturdy record or something, and a record player that can spin it at enough RPMs to give the edge of the disk a speed that is a significant fraction of the speed of light.
Since length shortens along the direction of motion, what would happen? Every spot on the circumference of the disk is moving perpendicular to the radius, which means that the length of the radius stays the same?
Do you now have a circle whose circumference isn't it's radius * 2 * pi?
What about points alongside the inside of the record? Their length contracts as well, but if it's a really big record, let's say it's radius is the size of the sun, the points along the inside are going to have drastically lower speeds, meaning less contraction.
It hurts my head thinking about it, but I was looking at my fan while I was lying down yesterday and the question came to me.
Can anyone set my mind at ease here?
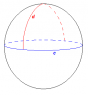
EDIT: I should mention I've been discussing it with my friends (my teacher didn't have an answer), and the theories we came up with were that space bends in such a way that the circle is resting on a dome (like you pushed an elastic circle with a rigid circumference up against the side of a ball). That would effectively make it so that the circumference is less than *pi times the diamater, but it seems kind of hokey. :/. Someone suggested that the rotating object collapses, but that seems even less viable.
Do either of these trains of thought have any merit?
Since length shortens along the direction of motion, what would happen? Every spot on the circumference of the disk is moving perpendicular to the radius, which means that the length of the radius stays the same?
Do you now have a circle whose circumference isn't it's radius * 2 * pi?
What about points alongside the inside of the record? Their length contracts as well, but if it's a really big record, let's say it's radius is the size of the sun, the points along the inside are going to have drastically lower speeds, meaning less contraction.
It hurts my head thinking about it, but I was looking at my fan while I was lying down yesterday and the question came to me.
Can anyone set my mind at ease here?
EDIT: I should mention I've been discussing it with my friends (my teacher didn't have an answer), and the theories we came up with were that space bends in such a way that the circle is resting on a dome (like you pushed an elastic circle with a rigid circumference up against the side of a ball). That would effectively make it so that the circumference is less than *pi times the diamater, but it seems kind of hokey. :/. Someone suggested that the rotating object collapses, but that seems even less viable.
Do either of these trains of thought have any merit?
Last edited: