- #1
FFX
- 8
- 0
Hey Everyone,
First time posting here, but you'll probably see me around quite a bit!
If this is in the wrong math spot please let me know, I don't understand the different types of maths.
I have an equation on the change of temperature, I substituted all the values in correctly and thought I was working it out correctly, but my answer came out far too low for me to think I did it correctly. If someone could have a look and give me guidance, that'd be great. The first picture is the full question, the second is me 'trying' to work it out! :(
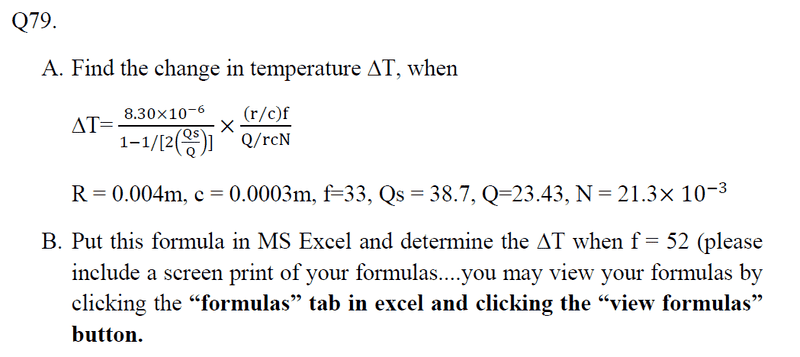
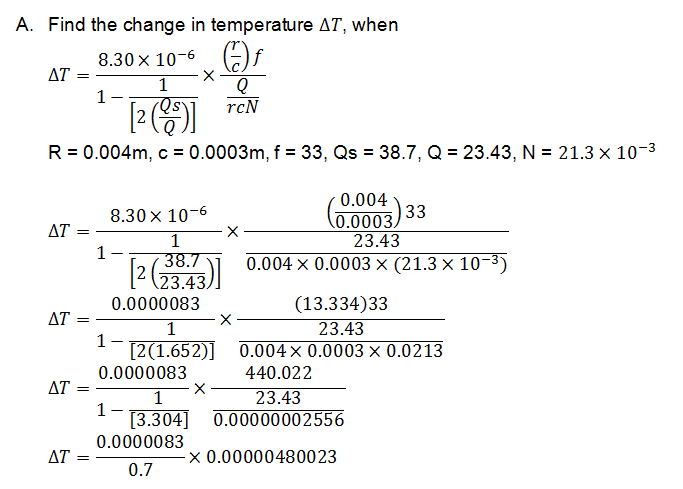
First time posting here, but you'll probably see me around quite a bit!
If this is in the wrong math spot please let me know, I don't understand the different types of maths.
I have an equation on the change of temperature, I substituted all the values in correctly and thought I was working it out correctly, but my answer came out far too low for me to think I did it correctly. If someone could have a look and give me guidance, that'd be great. The first picture is the full question, the second is me 'trying' to work it out! :(