- #1
vcsharp2003
- 897
- 176
- Homework Statement
- A charged circular non-conducting ring of radius ##R## has a charge density of ##\lambda## C/m. Would the electric field at point P as shown in diagram be zero? Explain the reason for your answer.
- Relevant Equations
- ##E=\dfrac {kq} {r^2}## where E is electric field magnitude due to a point charge q at a point that is distant r from the charge q
I have broken the ring into a top arc and a bottom arc.
First, let's assume an imaginary charge of +1 C is placed at point P. We will determine the force on this unit charge from top and bottom arcs.
The charges in the top arc will result in electric fields that will all cancel each other perpendicular to line CP leaving us with a net electric field pointing radially inward along the line CP. This is shown in diagram.
Similarly, the charges in bottom arc will result electric field vectors that will cancel each other perpendicular to line CP leaving us with a net electric field pointing radially outward along the line CP. This is shown in diagram.
After above analysis, I am confused since it's difficult to reason whether radial outward electric field at point P will be greater than or less than or equal to the radially inward electric field at the same point.

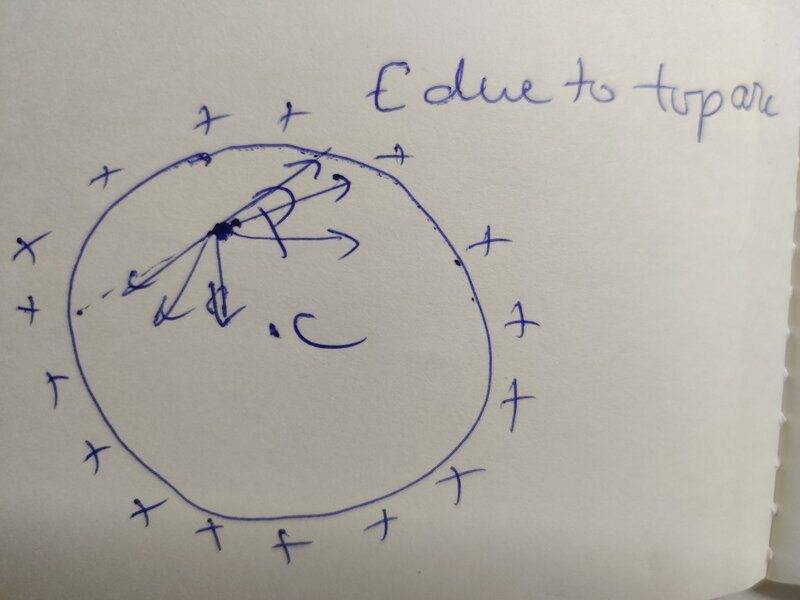

First, let's assume an imaginary charge of +1 C is placed at point P. We will determine the force on this unit charge from top and bottom arcs.
The charges in the top arc will result in electric fields that will all cancel each other perpendicular to line CP leaving us with a net electric field pointing radially inward along the line CP. This is shown in diagram.
Similarly, the charges in bottom arc will result electric field vectors that will cancel each other perpendicular to line CP leaving us with a net electric field pointing radially outward along the line CP. This is shown in diagram.
After above analysis, I am confused since it's difficult to reason whether radial outward electric field at point P will be greater than or less than or equal to the radially inward electric field at the same point.
Last edited: