- #1
Lavabug
- 866
- 37
Here goes a conceptual question that has been bugging me:
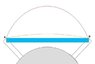
Consider the famous eclipse experiment that shows the Sun's gravitational lensing effect, allowing a star that would otherwise be obscured by the Sun to be visible from Earth.
Say an observer wanted to travel to the star from Earth and pointed his spaceship directly towards the star in question (at a speed v<<c).
If the observer did not look at the rest of the background stars and just did everything necessary to keep the spaceship pointed towards the target star, is there any way he/she would realize that the path being taken is not straight but in fact curved?
I presume if you had manual control over the ship's direction, you would progressively have to reorient the ship as the star changed position as you performed a close encounter with the Sun's gravitational field.
If the observer didn't know any GR/know that space was curved would he reasonably conclude that any steering he did was to correct any deflection from the Sun's gravity, and that the path was apparently straight?
What if the observer and ship were practically massless (but somehow still moved at v<<c, not sure if this is a non-sequitur on classical grounds), would he/she then be able to tell that the path was curved? (again without looking at background star motions)
Consider the famous eclipse experiment that shows the Sun's gravitational lensing effect, allowing a star that would otherwise be obscured by the Sun to be visible from Earth.
Say an observer wanted to travel to the star from Earth and pointed his spaceship directly towards the star in question (at a speed v<<c).
If the observer did not look at the rest of the background stars and just did everything necessary to keep the spaceship pointed towards the target star, is there any way he/she would realize that the path being taken is not straight but in fact curved?
I presume if you had manual control over the ship's direction, you would progressively have to reorient the ship as the star changed position as you performed a close encounter with the Sun's gravitational field.
If the observer didn't know any GR/know that space was curved would he reasonably conclude that any steering he did was to correct any deflection from the Sun's gravity, and that the path was apparently straight?
What if the observer and ship were practically massless (but somehow still moved at v<<c, not sure if this is a non-sequitur on classical grounds), would he/she then be able to tell that the path was curved? (again without looking at background star motions)