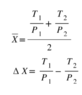- #1
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
How to find the change from a differentials problem (thermal)
- Thread starter Clara Chung
- Start date
-
- Tags
- Change Differentials Thermal
In summary, the conversation discusses a problem involving two systems in contact with different temperatures and pressures, with a constant total volume and internal energy. The systems are then allowed to re-equilibrate and the change in entropy is determined. It is noted that the equation used in the book only applies to closely neighboring equilibrium states, which may not be the case in this scenario. The conversation also touches upon the concept of Taylor expansion and applying it to a function of two variables.
Physics news on Phys.org
- #2
- 5,613
- 2,935
To me it looks like they got the signs wrong. ## \\ ## ## \Delta S_2=(\frac{1}{T_2}) \Delta U+ (\frac{p_2}{T_2}) \Delta V ##. ## \\ ## Meanwhile ## \Delta S_1=- [(\frac{1}{T_1})\Delta U+(\frac{p_1}{T_1}) \Delta V ] ##. ## \\ ## Then ## \Delta S _{system} =\Delta S_1+\Delta S_2 ## ## \\ ## @Chestermiller Did I get the sign wrong, or did the book get the sign wrong?
- #3
- 23,281
- 5,682
What is it supposed to mean for internal energy to be transferred from system 1 to system 2? Internal energy is supposed to be a physical property of a material, not a quantity of energy in transit? Do they mean heat rather than internal energy? I have major problems with this question statement. This is the kind of thing that confuses students to no end.Charles Link said:To me it looks like they got the signs wrong. ## \\ ## ## \Delta S_2=(\frac{1}{T_2}) \Delta U+ (\frac{p_2}{T_2}) \Delta V ##. ## \\ ## Meanwhile ## \Delta S_1=- [(\frac{1}{T_1})\Delta U+(\frac{p_1}{T_1}) \Delta V ] ##. ## \\ ## Then ## \Delta S _{system} =\Delta S_1+\Delta S_2 ## ## \\ ## @Chestermiller Did I get the sign wrong, or did the book get the sign wrong?
Chet
- #4
- 5,613
- 2,935
What I think you are saying, @Chestermiller is even if a quantity of heat ## \Delta Q ## goes from system 1 to system 2, there is no guarantee that it goes into the ## \Delta U ## term=it could also go into the ## p \Delta V ## term, so the problem really lacks definition. Did I understand your comments accurately?Chestermiller said:What is it supposed to mean for internal energy to be transferred from system 1 to system 2? Internal energy is supposed to be a physical property of a material, not a quantity of energy in transit? Do they mean heat rather than internal energy? I have major problems with this question statement. This is the kind of thing that confuses students to no end.
Chet
- #5
- 23,281
- 5,682
I think what the question meant to analyze was a case where you have two systems in contact with one another having different temperatures and pressures, but with a constant total volume and a constant total internal energy. The combined system is insulated and rigid on the outside. The barrier between the chambers is released and allowed to move freely, and the system is then allowed to re-equilibrate. The idea is to determine the change in entropy from the initial to the final state, and to show that the only way entropy doesn't increase is that if the initial temperatures and the initial pressures match. For ideal gases, this problem can be analyzed without too much trouble. But I strongly disagree with using Eqn. 14-24 to analyze this because that equation applies only to closely neighboring equilibrium states of a system, and the equilibrium states would not be closely neighboring for each of the two chambers involved.
If the OP would like to analyze the ideal gas problem I described, I would be glad to help.
If the OP would like to analyze the ideal gas problem I described, I would be glad to help.
Last edited:
- #6
- 23,281
- 5,682
Clara, Is your "like" an indication that you would like me to help you solve this problem correctly (rather than the way your book does it)?
- #7
Clara Chung
- 304
- 14
Dear Chestermiller,Chestermiller said:Clara, Is your "like" an indication that you would like me to help you solve this problem correctly (rather than the way your book does it)?
I am thinking about it
- #8
Clara Chung
- 304
- 14
I don't understand why the equation only applies to closely neighboring equilibrium states, could you explain a bit more?Chestermiller said:But I strongly disagree with using Eqn. 14-24 to analyze this because that equation applies only to closely neighboring equilibrium states of a system, and the equilibrium states would not be closely neighboring for each of the two chambers involved.
- #9
George Jones
Staff Emeritus
Science Advisor
Gold Member
- 7,643
- 1,598
Clara Chung said:I don't understand why the equation only applies to closely neighboring equilibrium states
Are you familiar (as covered in something like Calc 3) with the Taylor expansion about ##\left( x_0 , y_0 \right)## of a function of two variables, ##f \left( x , y \right)##?
- #10
- 23,281
- 5,682
The thermodynamic functions U and S apply only to thermodynamic equilibrium states.Clara Chung said:I don't understand why the equation only applies to closely neighboring equilibrium states, could you explain a bit more?
- #11
Clara Chung
- 304
- 14
Yes, f(x,y) = f(x0, y0) + ∂f/∂x (x-x0) + ∂f/∂y (y-y0) + error term, but what are the function and variables?George Jones said:Are you familiar (as covered in something like Calc 3) with the Taylor expansion about ##\left( x_0 , y_0 \right)## of a function of two variables, ##f \left( x , y \right)##?
- #12
Clara Chung
- 304
- 14
Chestermiller said:I think what the question meant to analyze was a case where you have two systems in contact with one another having different temperatures and pressures, but with a constant total volume and a constant total internal energy. The combined system is insulated and rigid on the outside. The barrier between the chambers is released and allowed to move freely, and the system is then allowed to re-equilibrate. The idea is to determine the change in entropy from the initial to the final state, and to show that the only way entropy doesn't increase is that if the initial temperatures and the initial pressures match. For ideal gases, this problem can be analyzed without too much trouble. But I strongly disagree with using Eqn. 14-24 to analyze this because that equation applies only to closely neighboring equilibrium states of a system, and the equilibrium states would not be closely neighboring for each of the two chambers involved.
If the OP would like to analyze the ideal gas problem I described, I would be glad to help.
If I use solve it as an ideal gas, let the system with T1 , P1, V1 and n1 be system 1.
Let the system with T2 , P2, V2 and n2 be system 2.
Let the final temperature be Tf and final pressure be Pf.
I try to find the change of entropy between two equilibrium states, so if change in entropy = 0, the two equilibrium states will be equivalent?(I am not sure about this statement.
The internal energy of the system 1 and system 2 remains the same, i.e. n1 T1 + n2 T2 = (n1 + n2 )Tf.
Consider system 1,
the first reversible step will be an isothermal expansion from V1 to V1 + V2,
the entropy change in this reversible process is n1 R ln (V1 + V2 / V1).
the second step will be an isochoric heating to slowly increase the temperature from T1 to Tf,
dQrev = Cv dT
ΔS1=Cv ln (Tf / T1) + n1 R ln (V1 + V2 /V1)
Similarly for system 2,
so the total change in entropy is:
ΔS1 + ΔS2
= Cv ln (Tf / T1) + n1 R ln ((V1 + V2) /V1) + Cv ln (Tf / T2) + n2 R ln ((V1 + V2) /V2)
Then I don't know how to preceed with the volume term...
- #13
Clara Chung
- 304
- 14
Sorry I have another question... Is entropy an unique property? Is there two different equilibrium states with different values of entropy? For example... Energy is a state function... but it is ok for two different equilibrium states to have the same temperature (but with different volume/ pressure) etc...
- #14
- 23,281
- 5,682
Very nice job, but the problem is a little over-specified and there should be n's in front of the Cv's. You need to use the ideal gas law for the initial conditions to express V1 and V2 in terms of P1, T1, n1 and P2, T2, n2. Also, if there is a sliding barrier present between the two gases, the final volume of each gas is not equal to the total volume of the container. For one of the gases, its volume increases, and, for the other gas, its volume decreases by an equal amount. The change in volume of each is determined by requiring that the final pressure is the same for each. So, with these comments, please go back and determine the final pressure.Clara Chung said:If I use solve it as an ideal gas, let the system with T1 , P1, V1 and n1 be system 1.
Let the system with T2 , P2, V2 and n2 be system 2.
Let the final temperature be Tf and final pressure be Pf.
I try to find the change of entropy between two equilibrium states, so if change in entropy = 0, the two equilibrium states will be equivalent?(I am not sure about this statement.
The internal energy of the system 1 and system 2 remains the same, i.e. n1 T1 + n2 T2 = (n1 + n2 )Tf.
Consider system 1,
the first reversible step will be an isothermal expansion from V1 to V1 + V2,
the entropy change in this reversible process is n1 R ln (V1 + V2 / V1).
the second step will be an isochoric heating to slowly increase the temperature from T1 to Tf,
dQrev = Cv dT
ΔS1=Cv ln (Tf / T1) + n1 R ln (V1 + V2 /V1)
Similarly for system 2,
so the total change in entropy is:
ΔS1 + ΔS2
= Cv ln (Tf / T1) + n1 R ln ((V1 + V2) /V1) + Cv ln (Tf / T2) + n2 R ln ((V1 + V2) /V2)
Then I don't know how to preceed with the volume term...
- #15
- 23,281
- 5,682
Yes. It is a physical property of the material.Clara Chung said:Sorry I have another question... Is entropy an unique property?
Sure. You already showed that for an ideal gas, where the entropy is a function of temperature and specific volume (or, equivalently, temperature and pressure)Is there two different equilibrium states with different values of entropy? For example... Energy is a state function... but it is ok for two different equilibrium states to have the same temperature (but with different volume/ pressure) etc...
- #16
Clara Chung
- 304
- 14
Chestermiller said:Very nice job, but the problem is a little over-specified and there should be n's in front of the Cv's. You need to use the ideal gas law for the initial conditions to express V1 and V2 in terms of P1, T1, n1 and P2, T2, n2. Also, if there is a sliding barrier present between the two gases, the final volume of each gas is not equal to the total volume of the container. For one of the gases, its volume increases, and, for the other gas, its volume decreases by an equal amount. The change in volume of each is determined by requiring that the final pressure is the same for each. So, with these comments, please go back and determine the final pressure.
P1 V1 = n1 R T1
P2 V2 = n2 R T2
Pf (V1+V2) = (n1 + n2) R Tf
so Pf = (n1 + n2) R Tf / (V1+V2)
The new volume of system 1 will be
V1f= n1 R Tf / [(n1 + n2) R Tf / (V1+V2)]
= (V1+V2) n1 / (n1+n2)
Similarly for system 2 the change in volume will be
V2f= n2 R Tf / [(n1 + n2) R Tf / (V1+V2)]
= (V1+V2) n2 / (n1+n2)
It is quite surprising that the volume each gas occupied is not the same as the total volume...
Should I proceed in the calculation of change in entropy with these results? However, the calculation will be so complicated because n1 and n2 will contribute as power in the logarithm...?
- #17
Clara Chung
- 304
- 14
So, if I have 2 systems, each with the same value of entropy, however the temperature of system A is higher than the temperature in system B, will there be a heat transfer from system A to system B?Chestermiller said:Yes. It is a physical property of the material.
Sure. You already showed that for an ideal gas, where the entropy is a function of temperature and specific volume (or, equivalently, temperature and pressure)
Mathematically, entropy is a function of T and V, i.e. S(T,V), is it possible that
S(T1 , V1) = S(T2, V2) for different temperature and volume? (This would imply two system are not in equilibrium even if ΔS=0 between the two systems)
or
S depends only on temperature?
- #18
- 23,281
- 5,682
Eliminate V1 and V2 using the initial conditions to express Pf exclusively in terms of T1, T2, P1, P2, n1, and n2. Then calculate entropy change of each gas from $$\Delta S=nC_p\Delta (\ln{T})-nR\Delta (\ln{P})$$
- #19
- 23,281
- 5,682
Sure.Clara Chung said:So, if I have 2 systems, each with the same value of entropy, however the temperature of system A is higher than the temperature in system B, will there be a heat transfer from system A to system B?
Let's see how it plays out when we do what I just said in my previous post.Mathematically, entropy is a function of T and V, i.e. S(T,V), is it possible that
S(T1 , V1) = S(T2, V2) for different temperature and volume? (This would imply two system are not in equilibrium even if ΔS=0 between the two systems)
or
S depends only on temperature?
- #20
- 23,281
- 5,682
To simplify things (temporarily), let's just, for now, focus on the case where n1=n2=1
- #21
- #22
- 23,281
- 5,682
What happened to the n2 chamber?Clara Chung said:
- #23
Clara Chung
- 304
- 14
If my equation is correct, the total change in entropy for n1=n2=1 will beChestermiller said:What happened to the n2 chamber?
??
Attachments
- #24
- 23,281
- 5,682
If I multiply and divide the expression in brackets of the 2nd term in your relationship by ##T_1T_2##, I obtain:Clara Chung said:If my equation is correct, the total change in entropy for n1=n2=1 will be
View attachment 236705
??
$$\Delta S=C_p\ln{\left(\frac{(T_1+T_2)^2}{T_1T_2}\right)}-R\ln{\left(\frac{(T_1+T_2)^2}{T_1T_2}\frac{\left(\frac{T_1}{P_1}\right)\left(\frac{T_2}{P_2}\right)}{\left(\frac{T_1}{P_1}+\frac{T_2}{P_2}\right)^2}\right)}$$This can be rewritten as:
$$\Delta S=C_v\ln{\left(\frac{(T_1+T_2)^2}{T_1T_2}\right)}+R\ln{\left(\frac{\left(\frac{T_1}{P_1}+\frac{T_2}{P_2}\right)^2}{\left(\frac{T_1}{P_1}\right)\left(\frac{T_2}{P_2}\right)}\right)}$$
OK so far? Note the similarity in mathematical form for the two logarithmic terms. I will continue after you indicate you are comfortable with these results.
- #25
Clara Chung
- 304
- 14
OK. I am comfortable with the resultChestermiller said:If I multiply and divide the expression in brackets of the 2nd term in your relationship by ##T_1T_2##, I obtain:
$$\Delta S=C_p\ln{\left(\frac{(T_1+T_2)^2}{T_1T_2}\right)}-R\ln{\left(\frac{(T_1+T_2)^2}{T_1T_2}\frac{\left(\frac{T_1}{P_1}\right)\left(\frac{T_2}{P_2}\right)}{\left(\frac{T_1}{P_1}+\frac{T_2}{P_2}\right)^2}\right)}$$This can be rewritten as:
$$\Delta S=C_v\ln{\left(\frac{(T_1+T_2)^2}{T_1T_2}\right)}+R\ln{\left(\frac{\left(\frac{T_1}{P_1}+\frac{T_2}{P_2}\right)^2}{\left(\frac{T_1}{P_1}\right)\left(\frac{T_2}{P_2}\right)}\right)}$$
OK so far? Note the similarity in mathematical form for the two logarithmic terms. I will continue after you indicate you are comfortable with these results.
- #26
- 23,281
- 5,682
We are missing a factor of 4 in the denominator of each of the logarithmic terms. For example, for the first term, the final temperature is ##(T_1+T_2)/2##
I can write $$T_1T_2=\left(\frac{(T_1+T_2)}{2}\right)^2-\left(\frac{(T_1-T_2)}{2}\right)^2$$ so $$\frac{(T_1+T_2)^2}{4T_1T_2}=\frac{1}{\left[1-\frac{1}{4}\left(\frac{\Delta T}{\bar{T}}\right)^2\right]}$$ where ##\bar{T}=\frac{(T_1+T_2)}{2}##and ##\Delta T=(T_1-T_2)##. This expression is >1 for all values ##\Delta T\neq 0##, which means it always makes a positive contribution to the entropy change.
What does the same procedure tell you for the second term in the entropy change equation.
I can write $$T_1T_2=\left(\frac{(T_1+T_2)}{2}\right)^2-\left(\frac{(T_1-T_2)}{2}\right)^2$$ so $$\frac{(T_1+T_2)^2}{4T_1T_2}=\frac{1}{\left[1-\frac{1}{4}\left(\frac{\Delta T}{\bar{T}}\right)^2\right]}$$ where ##\bar{T}=\frac{(T_1+T_2)}{2}##and ##\Delta T=(T_1-T_2)##. This expression is >1 for all values ##\Delta T\neq 0##, which means it always makes a positive contribution to the entropy change.
What does the same procedure tell you for the second term in the entropy change equation.
- #27
Clara Chung
- 304
- 14
Chestermiller said:We are missing a factor of 4 in the denominator of each of the logarithmic terms. For example, for the first term, the final temperature is ##(T_1+T_2)/2##
I can write $$T_1T_2=\left(\frac{(T_1+T_2)}{2}\right)^2-\left(\frac{(T_1-T_2)}{2}\right)^2$$ so $$\frac{(T_1+T_2)^2}{4T_1T_2}=\frac{1}{\left[1-\frac{1}{4}\left(\frac{\Delta T}{\bar{T}}\right)^2\right]}$$ where ##\bar{T}=\frac{(T_1+T_2)}{2}##and ##\Delta T=(T_1-T_2)##. This expression is >1 for all values ##\Delta T\neq 0##, which means it always makes a positive contribution to the entropy change.
What does the same procedure tell you for the second term in the entropy change equation.
And the same expression with T bar and ΔT replaced by X bar and ΔX. 2 X bar is always larger than ΔX, so the contribution to entropy is always positive. The change in entropy is zero when ΔT=0 and ΔX=0
Attachments
- #28
Clara Chung
- 304
- 14
Therefore the entropy change is zero if and if only the temperature and pressure are equal between two equilibrium states. Does it mean if two states have the same value of entropy, the two states are identical? (States with different temperature but the same entropy don't exist?)
- #29
- 23,281
- 5,682
No. It only means that, at equilibrium, entropy is a minimum with respect to changes in the intensive variables.Clara Chung said:Therefore the entropy change is zero if and if only the temperature and pressure are equal between two equilibrium states. Does it mean if two states have the same value of entropy, the two states are identical? (States with different temperature but the same entropy don't exist?)
1. How do I calculate the change from a differentials problem?
To find the change from a differentials problem, you will need to use the formula: dT = k * dQ / m * c. This formula represents the change in temperature (dT) as a result of a change in heat (dQ), with k being a constant, m being the mass of the substance, and c being its specific heat capacity. You will need to plug in the values for these variables to solve for the change in temperature.
2. What is the difference between a differential problem and a regular problem?
A differential problem is a type of problem that deals with small changes or variations in a system, while a regular problem deals with larger changes. In a differential problem, the goal is to find the change in a specific variable, such as temperature, due to a small change in another variable, such as heat. Regular problems, on the other hand, may involve finding the overall change in a system or solving for a specific value without taking into account small changes.
3. Can I use the same formula for all differentials problems?
No, the formula for finding the change from a differentials problem will vary depending on the specific variables involved. For example, if you are trying to find the change in pressure due to a change in volume, you will need to use a different formula than if you were trying to find the change in temperature due to a change in heat. It is important to understand the variables and their relationships in order to use the correct formula for each problem.
4. How can I determine the values for the variables in a differentials problem?
To determine the values for the variables in a differentials problem, you will need to gather information from the problem itself, such as the initial and final values for the variables, and any given constants or relationships between the variables. You may also need to use additional equations or formulas to solve for the values of the variables.
5. What units should I use for the variables in a differentials problem?
The units for the variables in a differentials problem will depend on the specific units used for each variable in the formula. For example, if the formula involves mass, it may use kilograms (kg) as the unit for mass. It is important to be consistent with units throughout the problem to ensure accurate calculations and results.
Similar threads
Engineering
How do I model a Thorium Reactor thermal system?
-
Engineering and Comp Sci Homework Help
- Replies
- 7
- Views
- 962
-
Engineering and Comp Sci Homework Help
- Replies
- 3
- Views
- 1K
-
Engineering and Comp Sci Homework Help
- Replies
- 1
- Views
- 1K
-
Introductory Physics Homework Help
- Replies
- 10
- Views
- 363
-
Thermodynamics
- Replies
- 3
- Views
- 362
-
Engineering and Comp Sci Homework Help
- Replies
- 16
- Views
- 1K
-
Mechanical Engineering
- Replies
- 16
- Views
- 482
-
Engineering and Comp Sci Homework Help
- Replies
- 9
- Views
- 2K
-
Engineering and Comp Sci Homework Help
- Replies
- 1
- Views
- 624
-
Earth Sciences
- Replies
- 7
- Views
- 1K
Share: