Inverse Trigonometric Function Problems

[Lucretius]

The math book I have does a pretty terrible job explaining this to me, because I am absolutely stumped as to why I get every question wrong in two sections: finding values of each expression in radians (can often be given in terms of ?) and finding approximate/exact values of the expressions.

Here is a problem where I have to find values for each expression in radians:

Sin^-1(1/2) The answer is ?/6, but as to how they got this answer I am completely stumped. I don't think I got one problem right in the section that I had to use no calculator on.

The next ones are worse though, because even though I can do actual calculation I still get them wrong. The problem is tan(Cos^-1(12/13)) and I am supposed to find the value of the expressions:

I did Cos^-1 which is hypotenuse/adjacent:

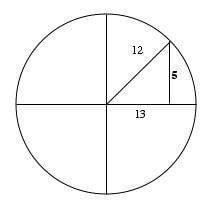
Then took tan, which is x/y, so I would get 13/15, but the answer is 5/12!

Anyways, any help would be appreciated.

 

[Hurkyl]

$$\cos^{-1} x \neq \frac{1}{\cos x}$$

You need to review what it means to be an inverse trig function.

(P.S. that triangle in your image cannot possibly be right)

 

[hypermorphism]

When your book uses the symbol ^-1 after a function, it means the inverse function, not the reciprocal of the function.
Cos^-1(x) is a function whose argument, x, is the cosine of some angle. The inverse cosine function, also called arccosine, returns the principal angle (the angle between -$$\pi$$ and $$\pi$$) whose cosine is x. In other words, Cos^-1(cos(t)) = t. The appropriately similar equations hold for the other inverse or arctrigonometric functions.
In response to Sin^-1(1/2), you are looking for the angle t such that sin(t) = 1/2. Drawing a triangle and letting the opposing leg (from t) have length 1, you see that the hypotenuse then has length 2 and the adjacent side has length $$\sqrt{3}$$. This is a common 30-60-90 triangle, and the angle t is then 30 degrees, or $$\frac{\pi}{6}$$ radians.

 

[dextercioby]

Read the previous post (#3) really carefully and then attempt to solve the equation

$$\sin x=\frac{1}{2}$$

Daniel.

 

[what]

you should have memorized the sine and cos function for 0, pi, pi/3, pi/4, and pi/6, and the corresponding angles in the other quadrents then just work backwards. For the other ones that don't correspond to those radian messures just use your calculator, almost all calculators have an arcsine or inverse sine button. And the posts above are also quite correct.

 

[Data]

Alright. You obviously know about the standard trigonometric function, $\sin \theta, \; \cos \theta$ and $\tan \theta$. The $\theta$ in these expressions is an angle. You are undoubtedly familiar with angles from geometry. On the ortherhand, the value of the functions is not and angle, it is a ratio of lengths.

The inverse trigonometric functions, $\sin^{-1} x, \; \cos^{-1} x,$ and $\tan^{-1} x$ work in a somewhat opposite manner. Here $x$ should be a ratio of lengths, and the value of each of these functions is an angle. They are such that

$$\sin^{-1} (\sin x) = \sin \left(\sin^{-1} x\right) = x$$

and

$$\cos^{-1} (\cos x) = \cos \left(\cos^{-1} x\right) = x$$

and

$$\tan^{-1} (\tan x) = \tan \left(\tan^{-1} x\right) = x.$$

Now, you have also probably learned that, for example,

$$\sin 0 = 0, \; \sin \frac{\pi}{2} = 1, \; \sin \frac{\pi}{6} = \frac{1}{2}, \; . \ . \ .$$

and similar identities for $\cos$ and $\tan$.

Let's consider your first question, to find $\sin^{-1} 1/2$. From what I wrote above, though, you see that

$$\sin \frac{\pi}{6} = \frac{1}{2}$$

so we can rewrite

$$\sin^{-1} \left(\frac{1}{2}\right) = \sin^{-1} \left( \sin \left( \frac{\pi}{6} \right) \right)$$

but from the rule that I mentioned above,

$$\sin^{-1} ( \sin x ) = x,$$

so we get

$$\sin^{-1} \left(\frac{1}{2}\right) = \sin^{-1} \left( \sin \left( \frac{\pi}{6} \right) \right)$$

$$= \frac{\pi}{6}.$$

You should try other questions in a similar way on your own.

Now, let's look at your second question. You want

$$\tan \left( \cos^{-1} \left( \frac{12}{13}\right) \right).$$

Your original intuition, to use the geometry of the triangle, was correct, but you made some mistakes. The way to approach such a question is as follows.

Remember that, as I said above, $\cos^{-1}$ returns a angle. It serves us to consider it to be an angle in a right triangle, for this question.

You should probably draw the triangle at this point. One of its angles is $\pi /2$ and another is $\cos^{-1} (12/13)$. What does this second angle mean, though?

Well, if $\cos \theta = x$, where $\theta$ is the angle, then as I mentioned earlier,

$$\cos^{-1} (\cos \theta) = \theta$$

so, if the angle is $\theta = \cos^{-1} (12/13)$, as it is in our question, then we can immediately say that

$$\cos \theta = \frac{12}{13}$$

! But thinking about this a little more solves our question: remember that $\cos$ is the ratio of the lengths of the adjacent side and the hypotenuse of the triangle, ie.

$$\cos \theta = \frac{\mbox{adjacent}}{\mbox{hypotenuse}} = \frac{12}{13}.$$

Then in our case, we can interpret the hypotenuse length to be $13$, and the adjacent length to be $12$. What is the length of the third side of the triangle? Well, we can use the familiar pythagorean theorem to say that it is

$$\mbox{opposite} = \sqrt{ \mbox{hypotenuse}^2 - \mbox{adjacent}^2} = \sqrt{13^2 - 12^2} = \sqrt{169 - 144} = \sqrt{25} = 5.$$

What we want, then is the tangent of the angle, ie. $\tan \theta$. But I'm sure you remember that

$$\tan \theta = \frac{ \mbox{opposite}}{\mbox{adjacent}}.$$

In our case, we have already found $\mbox{opposite} = 5$ and $\mbox{adjacent} = 12}$, so this is easy:

$$\tan \theta = \frac{5}{12}.$$

Recalling that $\theta$ is just $\cos^{-1} (12/13)$, we are finished, since we have found

$$\tan \left(\cos^{-1}\left(\frac{12}{13}\right)\right) = \tan \theta = \frac{5}{12}.$$

 

[Lucretius]

How do you guys get those math equations to be grouped as one whole item? Do you not type them out?

P.S. thanks for the answers, I'm trying to work it out now.

 

[Data]

Double-click on math notation to see the code to generate it in a popup window.

 

[dextercioby]

Data,though your post is quite big,nowhere does it include the essential fact that the functions $\sin,\cos$ are invertible only on a restricted domain,namely $\left[-\frac{\pi}{2},+\frac{\pi}{2}\right]$ for sine and $[0,\pi]$ for cosine...

Without that having been said,all those $\sin^{-1}\left(\sin \left(\mbox{blah,blah,blah}\right)\right)$ et al.make no sense...

Daniel.

P.S.We use Latex code.

 

[Data]

Indeed. But I get the impression that he doesn't know about the periodic natures of the functions yet, so I don't want to confuse him. It doesn't even seem like he's really figured out what invertibility is yet. If I tell him that now, it'll probably just be random babbling for him to ignore

 

[hypermorphism]

How do you guys get those math equations to be grouped as one whole item? Do you not type them out?

They are using this forum's native TeX interpreter. You can see exactly what they typed to get the graphics they did by clicking on the graphic you're interested in. Note the important "tex" tags surrounding each one.

 

[SpaceTiger]

Alright. You obviously know about the standard trigonometric function...

Wow, that's very nice, Data. Kudos for writing all that out.

 

[Data]

Better to explain it once clearly than several times unclearly

 

[Lucretius]

I did another problem to make sure I understood what you guys said:

$$\tan \left(\cos^{-1} \left(\frac{-12}{-13}\right)\right)$$

(how does the coding work so that I can just say it's a negative fraction?)

Anyways, I had left$$\cos^{-1} \left(\frac{-12}{-13}\right)$$

I got $$-13^2$$ - $$-12^2$$, and I got $$5$$

So $$\tan$$ of that would be $$\frac{-5}{-12}.$$

Meaning the answer would be $$\tan \left(\cos^{-1} \left(\frac{-12}{-13}\right)\right) = \frac{-5}{-12}$$


Is this correct?

 

[Lucretius]

Oh, another question, if I may be so bold!

Is there anyway to figure out $$\sin^{-1}\frac{1}{2}$$ without memorizing tables?

I know now the answer is $$\frac{\pi}{6}$$, but what exactly makes it that number? Is it just something I have to know?

Pre-calc is not my best subject, but I want to get better at it!

Thanks to everyone for their help; and thanks to Data for his über explanation!

 

[Data]

Do you actually mean

$$\cos^{-1} \left(-\frac{12}{13}\right)$$

?

in that case, the opposite side length is again $5$ and the answer is

$$\frac{-5}{12}$$

 

[Lucretius]

Do you actually mean

$$\cos^{-1} \left(-\frac{12}{13}\right)$$

?

in that case, the opposite side length is again $5$ and the answer is

$$\frac{-5}{12}$$

Yes, that is what I meant, I just couldn't figure out how to get that negative symbol where I wanted it to be; but now I know. Hurray I did it right!

My main problem was I really didn't know what an inverse function was. I had it all backwards, as Hurkyl explained to me.

 

[pack_rat2]

Oh, another question, if I may be so bold!

Is there anyway to figure out $$\sin^{-1}\frac{1}{2}$$...

Your just have to "think in reverse." An inverse trig function is always an angle. For Sin^-1(1/2), figure out what angle is there such that its sine is 1/2.

 

[Data]

As to your question about memorizing things, you do not actually have to memorize too much. The only trigonometric ratios that you will likely be expected to know are the "nice" ones (like the ones I mentioned, and a few more, such as $\cos \pi / 4 = \sin \pi / 4 = 1 / \sqrt{2}$).

 

[Lucretius]

Your just have to "think in reverse." An inverse trig function is always an angle. For Sin^-1(1/2), figure out what angle is there such that its sine is 1/2.

Yes, but wouldn't I have to memorize a chart in order to know at what angle sine is $$\frac{1}{2}$$?

 

[hypermorphism]

Yes, but wouldn't I have to memorize a chart in order to know at what angle sine is $$\frac{1}{2}$$?

Just two triangles, the 30-60-90 and the 45-45-90. Just remember the lengths of the sides of these triangles (the simplest representation of the relative lengths) in whatever way feels best to you and you're good to go.

 

[Data]

Like I said, there aren't many to memorize. Just a few nice fractions of $\pi$. If you get really bored and are worried you'll forget your calculator somewhere, you can buy a copy of the lovely Handbook of Mathematical Functions (my copy is published by Dover books) and have at some tables

 

[Lucretius]

As to your question about memorizing things, you do not actually have to memorize too much. The only trigonometric ratios that you will likely be expected to know are the "nice" ones (like the ones I mentioned, and a few more, such as $\cos \pi / 4 = \sin \pi / 4 = 1 / \sqrt{2}$).

hmm, most likely the nice ones yes.

Why is $$\cos^{-1}\frac{1}{2} = \frac{\pi}{3}$$, but $$\cos^{-1}-\frac{1}{2} = \frac{2\pi}{3}$$?

Why is it not just $$-\frac{\pi}{3}$$?

Hope I'm not becoming annoying with my incessant questions?

 

[Naeem]

$$\mbox{opposite} = \sqrt{ \mbox{hypotenuse}^2 - \mbox{adjacent}^2} = \sqrt{13^2 - 12^2} = \sqrt{169 - 144} = \sqrt{25} = 5.$$

 

[Data]

Not at all.

Have you seen trigonometric functions defined using the unit circle, or just triangles?

In general, $\cos (-\theta) = \cos \theta$, though, so $\cos^{-1} (-x) \neq \cos^{-1} x$.

 

[Lucretius]

Not at all.

Have you seen trigonometric functions defined using the unit circle, or just triangles?

In general, $\cos (-\theta) = \cos \theta$, though, so $\cos^{-1} (-x) \neq \cos^{-1} x$.

I guess just triangles. I was just wondering because $\cos$ seems to be the only one that will change if it's $\cos^{-1}$ to a different number.

I was just wondering what made it change like that, while $sin$ and $tan$ don't.

 

[Data]

Take a look at this webpage. Read carefully:

http://encyclopedia.laborlawtalk.com/Unit_circle

Here's my explanantion:

Draw the unit circle in the cartesian plane(circle of radius 1, centered at the origin). Now, rotate an angle $\theta$ counter-clockwise from the positive x-axis, and draw a line in this direction. This line intersects the circle at exactly one point. The $x$-coordinate of the intersection is defined to be $\cos \theta$ and the $y$-coordinate is defined to be $\sin \theta$. $\tan \theta$ is defined as $\sin \theta / \cos \theta$.

If you instead measure the angle clockwise from the positive $x$-axis, you put a negative sign in front of it.

Look at it carefully and see if you can figure out in which quadrants $\sin, \ \cos,$ and $\tan$ are positive and negative. Be sure to post with any questions!

 

[Lucretius]

Take a look at this webpage. Read carefully:

http://encyclopedia.laborlawtalk.com/Unit_circle

Here's my explanantion:

Draw the unit circle in the cartesian plane(circle of radius 1, centered at the origin). Now, rotate an angle $\theta$ counter-clockwise from the positive x-axis, and draw a line in this direction. This line intersects the circle at exactly one point. The $x$-coordinate of the intersection is defined to be $\cos \theta$ and the $y$-coordinate is defined to be $\sin \theta$. $\tan \theta$ is defined as $\sin \theta / \cos \theta$.

If you instead measure the angle clockwise from the positive $x$-axis, you put a negative sign in front of it.

Look at it carefully and see if you can figure out in which quadrants $\sin, \ \cos,$ and $\tan$ are positive and negative. Be sure to post with any questions!

So $\sin \theta$ is positive in Quadrants: 1,2 and negative in 3,4
$\cos \theta$ is positive in Quadrants: 1,4 and negative in 2,3
and $\tan \theta$ is positive in Quadrant 1 and negative in 2,3, and 4?

So $$\cos^{-1}-\frac{1}{2} = \frac{2\pi}{3}$$ because $\cos$ is $$-\frac{1}{2}$$, which would put it in quadrant 2 which should make it negative? I'm confused. I thought the adjacent was between the right angle and $\theta$, which would place it on the $x$ axis? Since $x$ is negative in quadrant 2, why is the answer positive?

I'm sorry I'm not understanding, I hope you aren't getting frustrated :(

 

[Data]

Not at all!

Alright, first let's standardize terminology:

I assume you mean

quadrant 1 is where x and y are both positive

quadrant 2 is where x is negative and y is positive

quadrant 3 is where x is negative and y is negative

quadrant 4 is where x is positive and y is negative.

Assuming that that is right, your analysis of where each function is + and - is perfect, except for one quadrant with the $\tan$ function: it is positive in quadrant 3. See if you can tell why.

Now, let's look at your question. First, I want you to forget your old definitions for the functions! At least for now (when you're dealing with normal triangles again, you should remember it again of course. For the purposes of these questions, you want to use the one I've shown you).

You are perfectly right: $\cos \theta = -1/2$ implies that $\theta$ is in one of the quadrants where cos is negative, of course . Now, you might ask, how do we know which quadrant it is in (since cos is negative in two of the four!)?

Well, the answer is something that dextercioby hinted to earlier: the inverse trig functions only give you values in certain ranges. $\sin^{-1}$ and $\tan^{-1}$ only give you values between $-\pi /2$ and $\pi / 2$, and $\cos^{-1}$ only gives you values between $0$ and $\pi$.

Anyways, going back to your question. Based on what I just said, the angle that we're looking for, given by

$$\cos^{-1} \left(-\frac{1}{2}\right),$$

must be between $0$ and $\pi$. Also, it must be in the second quadrant. The $x$-coordinate is $-1/2$, so the angle is indeed $2\pi / 3$. The sign of $\cos \theta$ does not correspond to the sign on $\theta$.

 

[Lucretius]

Assuming that that is right, your analysis of where each function is + and - is perfect, except for one quadrant with the $\tan$ function: it is positive in quadrant 3. See if you can tell why.

Oh yeah… $\frac{-x}{-y}=positive$

Anyways, going back to your question. Based on what I just said, the angle that we're looking for, given by

$$\cos^{-1} \left(-\frac{1}{2}\right),$$

must be between $0$ and $\pi$. Also, it must be in the second quadrant. The $x$-coordinate is $-1/2$, so the angle is indeed $2\pi / 3$. The sign of $\cos \theta$ does not correspond to the sign on $\theta$.

You lost me here. I now get that $tan^{-1}$ ranges between $0$ and $\pi$. But why is the $x$-coordinate is $-1/2$? Shouldn't it just be $-1$ because $\cos$ is $\frac{adjacent}{hypotenuse}$? And from there I still don't understand how we realize it is $\frac{2\pi}{3}$.

I feel dumber as we go along lol. I bet the answer is real simple and I'm just not clicking for some reason.

 

[Data]

The $x$-coordinate is $-1/2$ because we're considering whatever is the argument to $\cos^{-1}$ to be the cosine of some angle, so that the value of the expression is the angle itself.

Remember, the cosine is just the x-coordinate of a point on the circle! So, in this case, the cosine is $-1/2$, and that means that the corresponding point on the circle has an $x$-coordinate of $-1/2$. We then know that it's in the second quadrant, too, because like I said, $\cos^{-1}$ only gives us values in quadrants 1 and 2, and no points in quadrant 1 have negative x-coordinates.

So, we have a point in quadrant 2 with an x-coordinate of $-1/2$. There is exactly one point on the circle satisfying these conditions. We now need to know what the angle is, though.

Notice that this point is the reflection over the $y$-axis of the point on the circle with x-coordinate $1/2$ in quadrant 1 (ie. if we flip the point over the $y$-axis, we get the point with x-coordinate $1/2$ in quadrant 1, also on the circle). But you know what angle corresponds to that point, it's just $\pi / 3$.

The angle for our real point, with x-coordinate $-1/2$, is then just $\pi - \pi / 3 = 2\pi / 3$ (which you should be able to see geometrically from your picture)

 

[Lucretius]

Hmm…interesting. I think I understand now. So $cos^({-1}$ is different because of the range of values it can be in?

I guess I'll go memorize that chart, or better yet, I'll just write it down in my journal of notes. I can use those on tests…

Data, can't tell you how helpful you have been. Thanks for sticking it through with me !

 

[Data]

Let me state this a little bit more formally so that it might be a little clearer.

Okay. Let's say we want to find

$$\cos^{-1} x$$

for some given $x$. We know that

$$\cos^{-1} (\cos \gamma) = \gamma$$

(at least for $\pi \geq \gamma \geq 0$)

so we'll try to construct an expression something like that. Remember that $\cos^{-1}$ always returns an angle, so we can let it be equal to some angle $\theta$:

$$\theta = \cos^{-1} x$$

but then, this means that we can interpret this to mean

$$x = \cos \theta$$

thus, if we can find an angle $\theta$ such that

$$x = \cos \theta$$

then our question is solved. We also have to keep in mind that

$$0 \leq \theta \leq \pi$$

so we're only looking for angles in quadrants 1 and 2.

The hard part of the question, then, is just how to find $\theta$? You should do this by considering the situation geometrically, like I did in my last post to find the angle $2\pi / 3$ (or by just taking $\cos^{-1}(x)$ if you have your calculator handy ).

 

[Data]

good

if you have any more questions, just ask. I'm going to sleep for now, though! :zzz: