- #1
wirefree
- 108
- 21
I am appreciative of the opportunity afforded by this forum to submit a query.
Problem: See
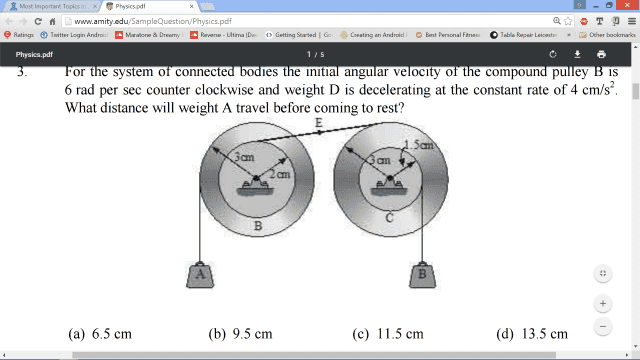
NOTE: The reference to weight "D" in the second line is incorrect.
My Attempt:
Step 1: Since this is a constant acceleration situation, I calculated the angular acceleration = 3 cm * -4 cm/s/s
Step 2: Employed the constant acceleration equation [tex] \omega^2 = \omega_0^2 + 2*\alpha*\theta [/tex] to obtain [tex]\theta = 3/2 [/tex]
Step 3: Lastly, I converted angle to length for the disc of radius 3 cm to obtain the required answer as 14.13 cm.Question: This approach does not yield. What should I relook?
Look forward to your suggestion.wirefree
Problem: See
NOTE: The reference to weight "D" in the second line is incorrect.
My Attempt:
Step 1: Since this is a constant acceleration situation, I calculated the angular acceleration = 3 cm * -4 cm/s/s
Step 2: Employed the constant acceleration equation [tex] \omega^2 = \omega_0^2 + 2*\alpha*\theta [/tex] to obtain [tex]\theta = 3/2 [/tex]
Step 3: Lastly, I converted angle to length for the disc of radius 3 cm to obtain the required answer as 14.13 cm.Question: This approach does not yield. What should I relook?
Look forward to your suggestion.wirefree