- #1
fletch-j
- 19
- 0
I am aware that for a function of two variables [itex]f(x,y)[/itex] a linear approximation of a point [itex]f(x,y)[/itex] close to [itex]f(x_0,y_0)[/itex] can be approximated by the tangent plane approximation [itex]f(x_0+\Delta x,y_0+\Delta y)\approx f(x_0,y_0)+f_x(x_0,y_0)\Delta x+f_y(x_0,y_0)\Delta y[/itex] where [itex]\Delta x=x-x_0[/itex] and [itex]\Delta y=y-y_0[/itex]
So is the process the same if you have a function [itex]f(x,y)[/itex] where [itex]x[/itex] and [itex]y[/itex] are themselves functions of more variables?
For example approximating [itex]f(x(s,t),y(s,t))[/itex] near [itex]f(x(s_0,t_0),y(s_0,t_0))[/itex]
Does it scale to an arbitrary number of nested functions?
Would it be something like:
[itex]f(x(s,t),y(s,t)) \approx f(x(s_0,t_0)+x_s(s_0,t_0)\Delta s+x_t(s_0,t_0)\Delta t , y(s_0,t_0)+y_s(s_0,t_0)\Delta s+y_t(s_0,t_0)\Delta t)[/itex]
or
[itex]f(x(s,t),y(s,t)) \approx f(x(s_0,t_0),y(s_0,t_0))+f_s(x(s_0,t_0),y(s_0,t_0))\Delta s + f_t(x(s_0,t_0),y(s_0,t_0))\Delta t[/itex]
??Also I have this problem:
Use a triple integral to find the volume of a solid cut from [itex]x=y^2[/itex] by the planes [itex]z=0[/itex] and [itex]x+z=1[/itex]
I just need help setting it out, I can do the calculation myself.
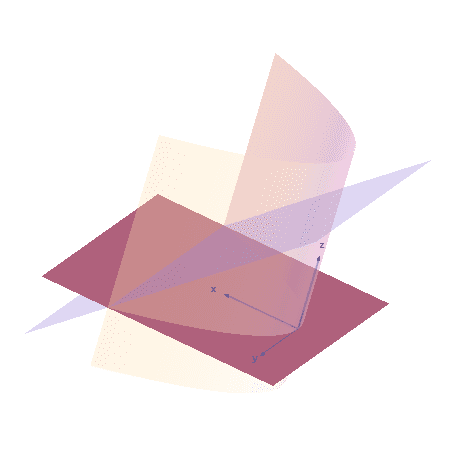
I had it set out as follows but I am unsure if it is correct.
[itex]\int^1_{-1} \int^1_0 \int^{1-z}_{y^2} dx dz dy[/itex]
Can someone please let me know if that is incorrect and if so, what I have done wrong.
Also I was wondering, (how) could this be written as a double integral?
So is the process the same if you have a function [itex]f(x,y)[/itex] where [itex]x[/itex] and [itex]y[/itex] are themselves functions of more variables?
For example approximating [itex]f(x(s,t),y(s,t))[/itex] near [itex]f(x(s_0,t_0),y(s_0,t_0))[/itex]
Does it scale to an arbitrary number of nested functions?
Would it be something like:
[itex]f(x(s,t),y(s,t)) \approx f(x(s_0,t_0)+x_s(s_0,t_0)\Delta s+x_t(s_0,t_0)\Delta t , y(s_0,t_0)+y_s(s_0,t_0)\Delta s+y_t(s_0,t_0)\Delta t)[/itex]
or
[itex]f(x(s,t),y(s,t)) \approx f(x(s_0,t_0),y(s_0,t_0))+f_s(x(s_0,t_0),y(s_0,t_0))\Delta s + f_t(x(s_0,t_0),y(s_0,t_0))\Delta t[/itex]
??Also I have this problem:
Homework Statement
Use a triple integral to find the volume of a solid cut from [itex]x=y^2[/itex] by the planes [itex]z=0[/itex] and [itex]x+z=1[/itex]
I just need help setting it out, I can do the calculation myself.
The Attempt at a Solution
I had it set out as follows but I am unsure if it is correct.
[itex]\int^1_{-1} \int^1_0 \int^{1-z}_{y^2} dx dz dy[/itex]
Can someone please let me know if that is incorrect and if so, what I have done wrong.
Also I was wondering, (how) could this be written as a double integral?
Last edited: