- #1
reigner617
- 28
- 0
Homework Statement
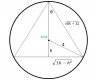
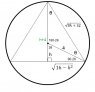
Find the area of the largest isosceles triangle that can be inscribed in a circle of radius 4. Solve by writing the area as a function of θ
Homework Equations
A=1/2 (bh)
The Attempt at a Solution
Given the side h and the hypotenuse 4, we can find the base of the smallest triangle to be sqrt(16-h2) which means that the base of the largest triangle is 2(sqrt(16-h2)). Its height can be considered as h+4, which would give a side length of sqrt(8h+32). So the area would be:
sqrt(16-h2)(h+4). However, they want it to be solved in terms of theta, which is the part that I am not sure of.