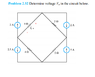
- #1
uasaki
- 16
- 0
Homework Statement
The problem is attached below.
Homework Equations
The Attempt at a Solution
I tried reducing the circuit by applying a source transformation to each pair of current sources and resistors. I reduced the CS and R at the upper left to an 8V source and 4-ohm resistor in series, the CS and R at the upper right to a -4V source and 2 ohm-resistor in series, the CS and R in the bottom left to a -10V source and 4-ohm resistor in series, and the CS and R in the bottom right to a 10V source and 2-ohm resistor in series. After all these source transformations, I'm left with a circuit with 4 voltage sources and four resistors all in series, which I know how to solve. I think I might be applying these source transformations incorrectly, because circuitlab (online circuit simulator) is giving me a different answer than the one I have.