Solving Special Relativity Equation: Rail Car Thought Experiment

[Alex Pavel]

I know that c is the same in all reference frames. I am trying to understand the rail car thought experiment.
I've got it down pretty well except for one part - the difference between an observer moving toward a light source versus an observer moving away from a light source at the same relative velocity.

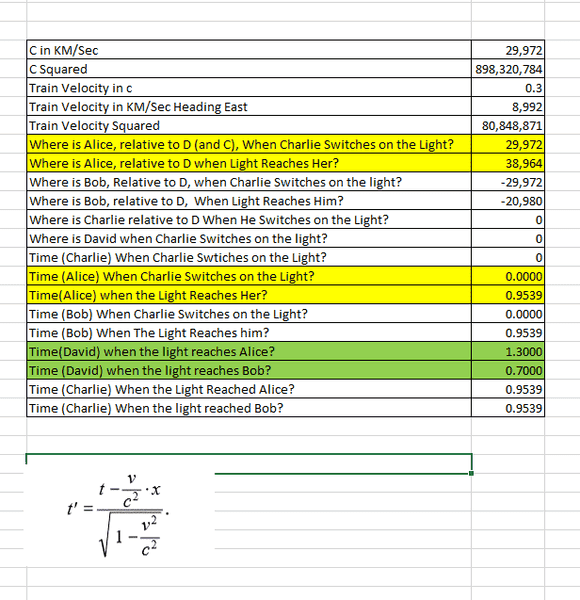
- We have two observers in a train moving east at, say, 0.3c relative to the track. It is a very long train, two light seconds long.
- We have two synchronized clocks at the two targets, A (Alice) and B (Bob)
- Observer (C) Charlie is on the train in the middle and he controls the light source
- Alice stands 1 light second east, and Bob is 1 light second west (29,972KM) of Charlie and the light source on the train
- An observer is on the track (David) with his own clock.
- When the center point of the train (Charlie) reaches David (on the track) the light source is switched on. At this even, all clocks are set to zero.

David, located on the track, measures the light reaching Alice at 1.3 seconds on his clock at a distance of 38,964KM east of David. He measures the light reaching Bob at 0.7 seconds, 20,980KM west of David.

- Alice, on the east end of the train, will record the time of light reaching her as 0.95 seconds.
- Bob, on the west end of the train is moving toward the light source at .3c, will also record the time of the light reaching him as: 0.95 on his clock.
Therefore, with Alice and Bob, using synchronized clocks, agree that the light beam has hit them simultaneously.

Whereas David (on the track) sees the light beam hit Bob 0.6 seconds earlier than Alice.
Thus, Alice and Bob at opposite ends of the train show identical time elapsed, while a 3rd party can perceive me meeting the light earlier or later.

This does not 'feel' correct yet I think I have the calculations right. Is this right?

Thank you!

 

[Doc Al]

- We have two observers in a train moving west at, say, 0.3c. It is a very long train, two light seconds long.
- We have two synchronized clocks at the two targets, A (Alice) and B (Bob)
- Observer (C) Charlie is on the train in the middle and he controls the light source

So A, B, C, and the light source are all on the train?

- Therefore Alice is moving away from the light source.
- And Bob is moving toward the light source.

I thought A and B were on the train? And the train is moving west.

- When the center point of the train (Charlie) reaches David (on the track) the light source is switched on. At this even, all clocks are set to zero.

According to what frame? When you say the clocks are set to zero, I assume you mean that according to observers on the train, clocks at A, B, and C all read zero at that moment. (Observers in the ground frame would disagree.)

Whenever you state where something is at a given time (such as when the light reaches them) you must also state according to what frame.

A diagram might be nice.

 

[PeroK]

@Alex Pavel You have a couple of conceptual errors buried in that post. A, B, C and the light source are all on the train. As far as they are concerned they are all at rest and only D is moving. Assuming the rest length of the train is 2 light seconds, they will measure 1s for the light to travel from C to A and from C to B. In their reference frame they are not moving relative to tge source.

If A, B and C synchronize their clocks, then D will not measure them as synchronised in his frame. This is as aspect of the relativity of simultaneity.

 

[Alex Pavel]

Okay I see what I misunderstood, problem solved. Thank you.