- #1
Kaushik
- 282
- 17
- Homework Statement
- Three springs are given. Their spring constants are k, 2k and 3k respectively. Their compressions are ## x_1 , x_2 , x_3 ## respectively. (Find the figure attached)
- Relevant Equations
- attached below.
I got the compression for the first one.
Second one, i am a bit confused. But i got ## x_2 = \frac{F}{2k} ##
In the third one, what will the compression be?
I have to get the ratio ## x_2 : x_3 ## . Then i will be able to get my answer.
Thanks.
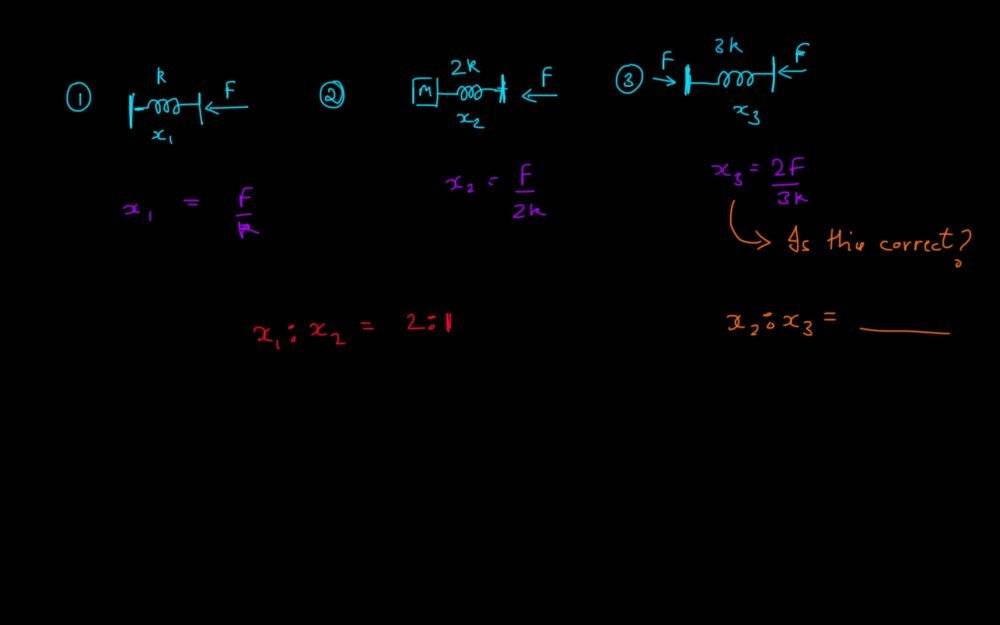
Second one, i am a bit confused. But i got ## x_2 = \frac{F}{2k} ##
In the third one, what will the compression be?
I have to get the ratio ## x_2 : x_3 ## . Then i will be able to get my answer.
Thanks.
 This is analogous to drawing 2 in which only one force ##F## is acting on the spring and the mass accelerates with constant acceleration. If we know the acceleration, we can find the compression.
This is analogous to drawing 2 in which only one force ##F## is acting on the spring and the mass accelerates with constant acceleration. If we know the acceleration, we can find the compression.