To derive an equation of black hole thermodynamics

[etotheipi]

This is the problem statement:

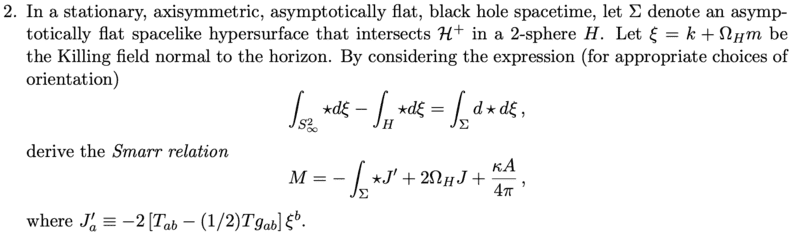
We can start by writing $(\star d \star d \xi)_a = - \nabla^b (d\xi)_{ab} = - \nabla^b \nabla_a \xi_b + \nabla^b \nabla_b \xi_a = 2\nabla^b \nabla_b \xi_a$. Then with $\nabla_a \nabla_b \xi_c = R_{cbad} \xi^d = -R_{bcad} \xi^d$ we can contract over $a$ and $b$ to find $\nabla^b \nabla_b \xi_c = -{R^b}_{cbd} \xi^d = -R_{cd} \xi^d$ so that \begin{align*}

(\star d \star d \xi)_a = -2R_{ab} \xi^b = -2 \left( 8 \pi \left[ T_{ab} - \frac{1}{2} T g_{ab} \right] \right) \xi^b := 8 \pi J_a'

\end{align*}Because the metric has Lorentzian signature the Hodge star for $p=1$ satisfies $\star \star d \star d \xi = -(-1)^p d \star d \xi = d \star d \xi$. Then we can use the expression in the question to write down\begin{align*}

\frac{1}{8\pi} \int_{\Sigma} d \star d \xi = \int_{\Sigma} \star J_a' = \frac{1}{8\pi} \int_{S_{\infty}^2} \star d \xi - \frac{1}{8\pi} \int_H \star d \xi

\end{align*}The second term $\frac{1}{8\pi} \int_{S_{\infty}^2} \star d \xi = \frac{1}{8\pi} \int_{S_{\infty}^2} \star d k + \frac{\Omega_H}{8\pi} \int_{S_{\infty}^2} \star dm= -M + 2\Omega_H J$ is negative the Komar mass plus two lots of $\Omega_H J$ so we are left to write\begin{align*}

M = -\int_{\Sigma} \star J_a' + 2\Omega_H J - \frac{1}{8\pi} \int_H \star d\xi

\end{align*}Now I am stuck because I don't know how to integrate $\star d\xi = \star d k + \Omega_H \star dm$ over $H$. I know that if $\xi^a \big{|}_{\mathcal{H}^+} = f n^a$ for some function $f$ and normal $n^a = \tilde{f} \partial^a S$ to the horizon then the surface gravity satisfies $\kappa = \xi \cdot \partial \log{|f|}$, but I don't see how it arises from this integral nor how $A$ is defined. Thanks in advance for any hints!

 

[etotheipi]

Just for the record, I noticed and fixed one mistake; the integral $\frac{1}{8\pi} \int_{S_{\infty}^2} \star d \xi$ should instead be$$\frac{1}{8\pi} \int_{S_{\infty}^2} \star d \xi = \frac{1}{8\pi} \int_{S_{\infty}^2} \star d k + \frac{\Omega_H}{8\pi} \int_{S_{\infty}^2} \star dm= -M + 2\Omega_H J$$given that $M := \frac{-1}{8\pi} \int_{S_{\infty}^2} \star d k$ and $J := \frac{1}{16\pi} \int_{S_{\infty}^2} \star d m$. The only thing left to show is that$$- \frac{1}{8\pi} \int_H \star d\xi = \frac{\kappa A}{4\pi}$$Does anyone know how to do this?

 

[etotheipi]

Tried to look at this question again with fresh eyes, but can't say it's any clearer to me. $\mathcal{H}^+$ is a Killing horizon (of $\xi^a$) and thus null, so we can put Gaussian null coordinates $(r,\lambda, y^i)$ on it by letting $y^i$ be coordinates on the 2-sphere $H$ and carrying these to the rest of $\mathcal{H}^+$ in the usual way. Thus on $H$ we have $\xi^a = fU^a$ with $U^a = (\partial / \partial \lambda)^a$ and so $\kappa = \xi^a \partial_a \mathrm{log}|f| = \partial f / \partial \lambda \implies f = \kappa \lambda + g(y^i)$. Then $\xi^a = \kappa \lambda U^a + g(y^i) U^a$. How do you determine the function $g$?

 

[PeterDonis]

How do you determine the function $g$?

Doesn't it have to be zero? Heuristically, $\xi^a$ is a KVF and is orthogonal to the spherical symmetry KVFs, but any variation with $y^i$ would have to be "in the direction" of at least one of those KVFs.

 

[etotheipi]

Thanks, that seems reasonable! We would have $d\xi_{ab} = \kappa \lambda dU_{ab}$ and thus\begin{align*}
\star d\xi_{ab} = \frac{\sqrt{-g}}{2!} g^{ce}g^{df}\epsilon_{abef} d\xi_{cd} = \frac{\kappa \lambda}{2} \sqrt{\gamma} g^{ce}g^{df}\epsilon_{abef} dU_{cd}

\end{align*}where $\gamma_{ab}$ (with $\sqrt{-g} = \sqrt{\gamma}$) is the induced metric on $H$. Then write the integral,\begin{align*}

\int_H \star d\xi = \int_{\phi(H)} d^2 y (\star d\xi)_{12} &= \frac{\kappa}{2} \int_{\phi(H)} d^2 y \sqrt{\gamma} \lambda g^{ce}g^{df}\epsilon_{12ef} dU_{cd} \\

&= \kappa \int_{\phi(H)} d^2 y \sqrt{\gamma} \lambda g^{ce} g^{df} \epsilon_{12ef}\partial_{[c} U_{d]}
\end{align*}with $d^2 y = dy_1 \wedge dy_2$. I'm not sure how to handle that; I believe that$$A = \int_{\phi(H)} d^2 y \sqrt{\gamma}$$but I need to think more about how to evaluate $\lambda g^{ce} g^{df} \epsilon_{12ef}\partial_{[c} U_{d]}$. The $\lambda$ seems a little bit suspect - I've just realized that on $H$ we should have $\lambda = 0$, so I think I've messed up somehow in defining the coordinates.

 

[etotheipi]

Doesn't it have to be zero? Heuristically, $\xi^a$ is a KVF and is orthogonal to the spherical symmetry KVFs, but any variation with $y^i$ would have to be "in the direction" of at least one of those KVFs.

Actually, I don't know if this will hold true. On $H$ we will have $\lambda = 0$ and thus $f = g(y^i)$. And since $\xi$ is non-zero on $H$ we must also have $g \neq 0$. I think setting $g=0$ might be where post #5 went wrong.

 

[PeterDonis]

I've just realized that on $H$ we should have $\lambda = 0$

I had thought you were using $\lambda$ to denote the affine parameter along a null geodesic generator of the horizon. If so, it would not be zero.

 

[PeterDonis]

Thus on $H$ we have $\xi^a = fU^a$

I think $f$ should be a constant, which should in fact be the surface gravity $\kappa$ with an appropriate choice of normalization. Since $\xi^a$ is a KVF, any geometric quantity must be constant along its integral curves, and that includes the norm of $\xi^a$, which is basically what $f$ is capturing.

 

[etotheipi]

Thanks for the replies!

I had thought you were using $\lambda$ to denote the affine parameter along a null geodesic generator of the horizon. If so, it would not be zero.

But by construction I defined the $y^i$ on $H$ and then assigned the coordinates $(\lambda, y^i)$ to the point at affine parameter distance $\lambda$ from $H$ along the geodesic with tangent $U^a$, so by definition $\lambda = 0$ on $H$ right?

I think $f$ should be a constant, which should in fact be the surface gravity $\kappa$ with an appropriate choice of normalization. Since $\xi^a$ is a KVF, any geometric quantity must be constant along its integral curves, and that includes the norm of $\xi^a$, which is basically what $f$ is capturing.

I'm not sure I completely understand because $\kappa = \xi^a \partial_a \mathrm{log}|f| = \partial f / \partial \lambda \implies f = \kappa \lambda + g(y^i)$ would suggest that it varies with $\lambda$ and $y^i$

Also, $U = \partial / \partial \lambda$ being a holonomic basis vector derived from a coordinate function is not necessarily normalised, so I thought $\xi_a \xi^a = f^2 U_a U^a \neq f^2$ and $f$ isn't necessarily identified with the norm of $\xi$

 

[PeterDonis]

by construction I defined the $y^i$ on $H$ and then assigned the coordinates $(\lambda, y^i)$ to the point at affine parameter distance $\lambda$ from $H$ along the geodesic with tangent $U^a$, so by definition $\lambda = 0$ on $H$ right?

No. You are confusing metric "distance" with affine parameter. They can be the same along timelike or spacelike curves, but not along null curves. The whole point of an affine parameter is that every point on the curve has to have a different value of it. So obviously you can't use metric "distance" as an affine parameter along a null curve.

 

[etotheipi]

No. You are confusing metric "distance" with affine parameter. They can be the same along timelike or spacelike curves, but not along null curves. The whole point of an affine parameter is that every point on the curve has to have a different value of it. So obviously you can't use metric "distance" as an affine parameter along a null curve.

I don't understand, the generators are lines of constant $y^i$ and to assign null coordinates $(\lambda, y^i)$ to some point $p \in \mathcal{N}$ in the null hypersurface you simply label it with the affine parameter distance $\lambda$ from $\mathcal{N}$ along the generator passing through the point in $H$ with coordinates $y^i$. All the points on the same generator are different affine parameter distances from $H$

By affine parameter distance I of course don't mean the metric distance c.f. $ds^2 = g_{\mu \nu} dx^{\mu} dx^{\nu}$ (which is clearly zero between everywhere in a null hypersurface)

 

[PeterDonis]

$U = \partial / \partial \lambda$ being a holonomic basis vector derived from a coordinate function is not necessarily normalised, so I thought $\xi_a \xi^a = f^2 U_a U^a \neq f^2$ and $f$ isn't necessarily identified with the norm of $\xi$

"Norm" was a bad choice of words on my part since null vectors can't be normalized anyway; $\xi_a \xi^a = 0$ on the horizon, and so is $U_a U^a$. So you can't actually deduce anything about $f$ by considering norms.

As for what you can deduce about $f$, see below.

I'm not sure I completely understand because $\kappa = \xi^a \partial_a \mathrm{log}|f| = \partial f / \partial \lambda \implies f = \kappa \lambda + g(y^i)$ would suggest that it varies with $\lambda$ and $y^i$

John Wheeler used to say that you should never do a calculation until you've already figured out what the answer should be. I think his advice is good for this problem.

Geometrically speaking, the vector field $\xi^a$ on the horizon has three important geometric properties:

(1) It is a Killing vector field.

(2) It is null.

(3) Its integral curves generate the horizon (i.e., every point on the horizon $\mathcal{H}^+$ lies on one and only one such curve). (You might think about how we know this.)

Also, we have one important property of the spacetime geometry as a whole:

(4) It is axisymmetric.

(Note that I was confused earlier about what scenario we were discussing, I had thought we were discussing the spherically symmetric case. For the axisymmetric case, I think it turns out, for reasons given below, that $\xi^a$ can vary with $\theta$, but not $\phi$, to use the standard notation for the angular coordinates in axisymmetric spacetimes instead of your generic $y^i$ notation. We can always choose those standard coordinates without loss of generality, so I don't see any harm in adopting them.)

What do these properties imply, geometrically?

(1) implies, as I've already said, that any geometric property must be constant along integral curves of $\xi^a$. That includes the inner product of $\xi^a$ with any other vector fields that we can define in a coordinate-independent manner. There are at least two of those that we know of, namely $k$ and $m$, the ones that appear in the definition of $\xi$. In standard Boyer-Lindquist coordinates, $k = \partial / \partial t$ and $m = \partial / \partial \phi$. Note that both of these are also KVFs (though neither of them are null on the horizon--in fact they are both spacelike, except at the two points where the horizon intersects the axis of symmetry of the spacetime, where $k$ is null).

(2) implies, as I said in my previous post, that we cannot use metric "distance" along the integral curves of $\xi^a$ as an affine parameter (or a coordinate, for that matter) on the horizon. So if your $\lambda$ coordinate is to be an affine parameter along those curves (which is indeed a good choice, for reasons that will appear in a moment), then every point on a given curve must have a different value of $\lambda$. However, we can still pick out points on different integral curves of $\xi^a$ that have the same value of $\lambda$; and an obvious choice for how to do that is to assign the value $\lambda = 0$ to all the points on the 2-surface $H$.

(3) actually is needed to justify the statement I just made, since it is what tells us that every point on $H$ lies on one and only one integral curve of $\xi^a$.

(4) implies that $m = \partial / \partial \phi$ is a KVF. That means that, just as with $\xi$, any geometric property must be constant along integral curves of $m$. One such integral curve is the "equator" of the 2-surface $H$. The inner product of $\xi$ and $m$ is a geometric quantity, and must therefore be constant along this "equator". Combining this with (1) above, we find that this inner product must be constant along the "equator" of every 2-surface on the horizon.

Now consider the coordinates we've defined on $\mathcal{H}^+$. We leave out $r$ since it is constant everywhere on $\mathcal{H}^+$, so it can't be a coordinate; but $\mathcal{H}^+$ is only a 3-surface, so we only need three coordinates. We already have two: $\phi$ and $\theta$. The obvious third coordinate, as I said above, is $\lambda$, the affine parameter along the integral curves of $\xi$. But note what that means: it means the vector field $\partial / \partial \lambda$ "points in the same direction" as $\xi$. In fact, we can simply choose $\lambda$ so that it is $\xi$. And that choice makes your $f$ equal to $1$ and your $g$ equal to zero--because $\xi$ is orthogonal to the 2-surface spanned by the other two coordinates $\theta$ and $\phi$, so it obviously can't have any components in either of those coordinate directions.

Now, finally, consider the surface gravity $\kappa$. It is also a geometric quantity, which means that it must be constant along integral curves of $\xi$ and $m$. That means it cannot be a function of either $\lambda$ or $\phi$; it can only be a function of $\theta$. And, except for the edge case of Schwarzschild spacetime, where the spacetime is actually spherically symmetric instead of just axisymmetric, we would expect $\kappa$ to vary with $\theta$.

One more item: in all your equations for $\kappa$, you have been writing partial derivatives. But they should be covariant derivatives, since we are working in a curved spacetime. The partial derivative of $f$ along integral curves of $\xi$, given the above, is obviously zero; but the covariant derivative is not, because the terms in the connection coefficients have to be considered. You should find that at least one of those terms does not vanish when contracted with $\xi^a$. (Note that this calculation should look similar to the one that tells you that integral curves of $\xi^a$ in a spacetime like Kerr or Schwarzschild, not on the horizon but in the exterior region, have nonzero proper acceleration. The surface gravity on the horizon is in fact a sort of "redshifted proper acceleration".)

 

[etotheipi]

Thanks, that was interesting! I only had one thing to ask about;

One more item: in all your equations for κ, you have been writing partial derivatives. But they should be covariant derivatives, since we are working in a curved spacetime. The partial derivative of f along integral curves of ξ, given the above, is obviously zero; but the covariant derivative is not, because the terms in the connection coefficients have to be considered.

I think that $\nabla_a f = \partial_a f$ because the covariant derivative of a function is nothing but its partial derivative, so they should both be zero

 

[PeterDonis]

I think that $\nabla_a f = \partial_a f$ because the covariant derivative of a function is nothing but its partial derivative, so they should both be zero

You're not taking the covariant derivative of $f$, you're taking the covariant derivative of $\xi^a$ (since, as I have said, your vector field $U^a$ is $\xi^a$). That's not the same thing. Again, I strongly suggest considering how we calculate the proper acceleration of an observer following an integral curve of the timelike KVF outside the horizon; the same issue arises.

 

[etotheipi]

You're not taking the covariant derivative of $f$, you're taking the covariant derivative of $\xi^a$ (since, as I have said, your vector field $U^a$ is $\xi^a$). That's not the same thing.

Which equation are you referring to? As far as I can tell I don't think I need to introduce covariant derivatives into any of the equations that I wrote...

 

[PeterDonis]

Which equation are you referring to?

The equation for $\kappa$. You wrote it as $\kappa = \xi^a \partial_a | \log f |$ but I don't think that's correct. The general equation, I believe, is $\kappa = \xi^a \nabla_a \xi^b$, evaluated on the horizon.

 

[etotheipi]

The general equation, I believe, is $\kappa = \xi^a \nabla_a \xi^b$, evaluated on the horizon.

This cannot be true because the LHS is a scalar and the RHS a vector!

If the Killing horizon of $\xi^a$ is $\mathcal{N}$, then $\mathcal{N}$ is a surface of constant $\xi^a \xi_a = 0$ hence $\nabla_a (\xi^b \xi_b) |_{\mathcal{N}}$ is orthogonal to $\mathcal{N}$ which can be expressed as $\nabla_a (\xi^b \xi_b) |_{\mathcal{N}} := -2\kappa \xi_a$ for some function $\kappa$. Then we write\begin{align*}
\nabla_a (\xi^b \xi_b) |_{\mathcal{N}} &= \xi^b \nabla_a \xi_b |_{\mathcal{N}} +\xi_b \nabla_a \xi^b |_{\mathcal{N}} \\

&= 2\xi^b \nabla_a \xi_b |_{\mathcal{N}} \\

&= -2 \xi^b \nabla_b \xi_a |_{\mathcal{N}} = -2 \kappa \xi_a\end{align*}by Killing's equation which gives$$\xi^b \nabla_b \xi^a |_{\mathcal{N}} = \kappa \xi^a$$Similarly let $U^a$ be the tangent to the affinely parameterised generator on $\mathcal{N}$, then $\xi^a = f U^a$ for some $f$ and on $\mathcal{N}$ we have\begin{align*}
\xi^b \nabla_b \xi^a = f U^a U^b \partial_b f = f^{-1} \xi^a \xi^b \partial_b f = \xi^a \xi^b \partial_b \mathrm{log}|f|
\end{align*}thus $\kappa = \xi^a \partial_a \mathrm{log}|f|$. Also the modulus signs should be around the $f$ and not the $\mathrm{log} f$

 

[PeterDonis]

This cannot be true because the LHS is a scalar and the RHS a vector!

Yes, $\kappa$ would actually be the norm of the vector on the RHS. (At least, I think that's correct.)

$$\xi^b \nabla_b \xi^a |_{\mathcal{N}} = \kappa \xi^a$$

Ok so far.

Similarly let $U^a$ be the tangent to the affinely parameterised generator on $\mathcal{N}$

No. That tangent is, as I have already said, just $\xi^a$. There is no need to introduce a separate vector field at all.

on $\mathcal{N}$ we have\begin{align*}
\xi^b \nabla_b \xi^a = f U^a U^b \partial_b f = f^{-1} \xi^a \xi^b \partial_b f = \xi^a \partial_a \mathrm{log}|f|
\end{align*}thus $\kappa = \xi^a \partial_a \mathrm{log}|f|$.

No.

First, as above, you don't need to introduce $U^a$ at all.

Second, even if you do introduce $f$, $\xi^b \nabla_b \xi^a = f U^a U^b \partial_b f$ is wrong. You are assuming that $\nabla_b U^a$ is zero, and it isn't. You are ignoring the connection coefficient terms. Try writing it out explicitly.

 

[etotheipi]

@PeterDonis I don't see your objection; I'm fairly certain this equation is correct, it's even stated in the Townsend notes in section 2.3.6. (Townsend also introduces the vector I call $U$ but denotes it by the letter $l$).

First, as above, you don't need to introduce $U^a$ at all.

It is very helpful to introduce $U^a$ because $U^a \nabla_a U^b = 0$, i.e. its geodesics are affinely parameterised, which is not the case for $\xi$.

Second, even if you do introduce $f$, $\xi^b \nabla_b \xi^a = f U^a U^b \partial_b f$ is wrong. You are assuming that $\nabla_b U^a$ is zero, and it isn't. You are ignoring the connection coefficient terms. Try writing it out explicitly.

I am not assuming that $\nabla_b U^a$ is zero - it's not - I am using that $U^b \nabla_b U^a = 0$ on $\mathcal{N}$

Viz. on $\mathcal{N}$ with $U^a \nabla_a U^b = 0$ we write\begin{align*}
\xi^b \nabla_b \xi^a = fU^b \nabla_b (fU^a) = fU^b U^a \nabla_b f = fU^a U^b \partial_b f = f^{-1} \xi^a \xi^b \partial_b f = \xi^a \xi^b \partial_b \mathrm{log}|f|

\end{align*}from which Townsend's result $\kappa = \xi^a \partial_a \mathrm{log}|f| = \xi \cdot \partial \mathrm{log}|f|$ arises

 

[etotheipi]

Yes, κ would actually be the norm of the vector on the RHS. (At least, I think that's correct.)

I don't think this is right either, because on $\mathcal{N}$ and denoting $\xi^b \nabla_b \xi^a = v^a$ we have$$v^a = \kappa \xi^a \implies v_a v^a = \kappa^2 \xi_a \xi^a = 0$$whilst $\kappa$ is definitely non-zero

 

[PeterDonis]

It is very helpful to introduce $U^a$ because $U^a \nabla_a U^b = 0$, i.e. its geodesics are affinely parameterised, which is not the case for $\xi$.

Yes, agreed.

I am not assuming that $\nabla_b U^a$ is zero - it's not - I am using that $U^b \nabla_b U^a = 0$ on $\mathcal{N}$

Ah, yes, I see. I think I'm used to doing this computation a different way, which I'll try to reconstruct in a future post if I have time. I'll take a look at the Townsend notes.

 

[PeterDonis]

we have$$v^a = \kappa \xi^a \implies v_a v^a = \kappa^2 \xi_a \xi^a = 0$$whilst $\kappa$ is definitely non-zero

Yes, but $\xi^a$ is null on the horizon so $\xi_a \xi^a = 0$, so we don't have to have $\kappa = 0$ to have $v_a v^a = 0$.