Transformation of coordinate basis

[Antarres]

TL;DR Summary

Confusion about coordinate transformation on a tangent bundle

So while reading T. Frankel's "The Geometry of Physics", I was going through the part on cotangent bundles which ended with the definition of Poincare 1-form. The author argued that cotangent bundles are better suited than tangent bundles for some problems in physics and that there is no natural isomorphism between them, that is, one has to include a metric as an additional structure to relate vectors to covectors. He said that some objects naturally live in the cotangent bundle(for example canonical momenta), etc.

So at the end of that discussion there is an exercise that is(I presume) supposed to show that an equivalent of Poincare 1-form doesn't exist on a tangent bundle. The precise statement of the exercise is given in the screenshot below.

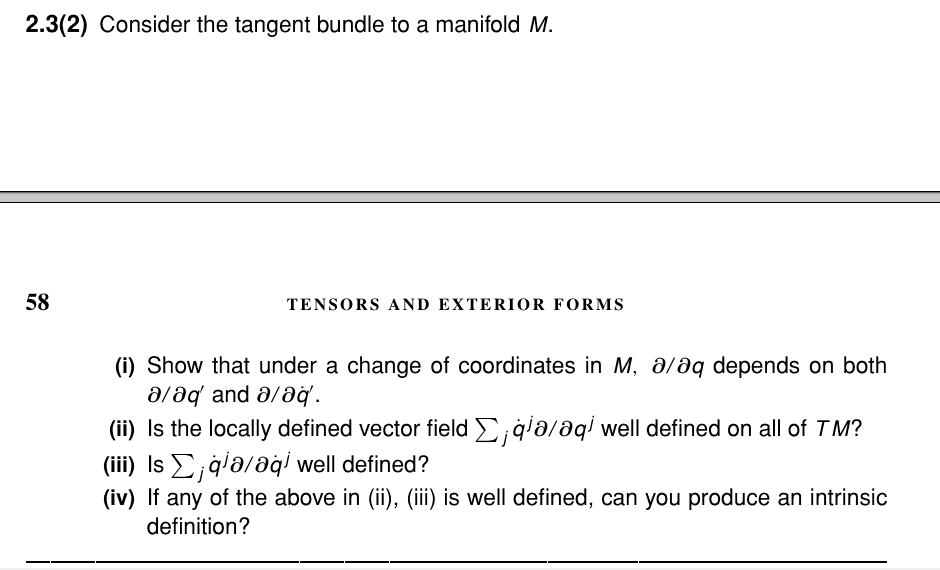
The exercise doesn't look hard, but still, when I look at the (i) I don't see why $\bf{\partial/\partial q}$ would depend on both $\bf{\partial/\partial q}$ and $\bf{\partial/\partial \dot{q}}$. $q$ is a letter reserved for the coordinate basis. $\dot{q}$ is a generalized velocity(that is, the on tangent bundle the coordinates are of form $(q,\dot{q})$).
Doesn't the coordinate change $q'^i = q'^i(q^1,\dots , q^n)$ imply the transformation:
$$\frac{\partial}{\partial q^i} = \frac{\partial q'^j}{\partial q^i}\frac{\partial}{\partial q'^j}$$

P.S. This is not a homework, just something I'm doing in my free time.

 

[martinbn]

Well, you said that the coorinates are $(q_1,\dots,q_n,\dot{q}_1,\dots,\dot{q}_n)$, a change of coordinates will take $(q_1,\dots,q_n,\dot{q}_1,\dots,\dot{q}_n)$ to $(q'_1,\dots,q'_n,\dot{q}'_1,\dots,\dot{q'}_n)$. Then it is clear that $\frac{\partial}{\partial q_1}$ in general will change to something involving $\frac{\partial}{\partial q'_i}$ and the $\frac{\partial}{\partial \dot{q}'_i}$ .

 

[Antarres]

Upon thinking a bit more about this, I arrived at this sort of conclusion. When the author defined the tangent bundle as the space of all tangent vectors to a manifold at all points, he proved that it is a $2n$-dimensional manifold as well(the original manifold being $n$-dimensional).

In that proof he considered that an arbitrary coordinate change $q'^i = q'^i(q^1,\dots , q^n)$ induces a change in velocities(which is just a normal change of vector components):
$$\dot{q}'^i = \frac{\partial q'^i}{\partial q^j}\dot{q}^j$$
with summation implied.
Under this coordinate change we have that transition maps between patches are smooth.
So when he considered coordinate change on the tangent bundle, he considered this type of coordinate change(which isn't exactly completely arbitrary).

Now velocities and positions are generally independent, so that confused me, but like you said, it looks like this vector $\frac{\partial}{\partial q}$ is tangent to the tangent bundle $TM$, not the manifold $M$ itself(because $\frac{\partial}{\partial\dot{q}}$ don't exist in the tangent space to $M$).

Now in the case when we have manifold $M$ with coordinates $q$, this Jacobian matrix that is the transformation between vector components should be computed at the exact point where we're calculating the tangent vector, so it should be a constant matrix(right?). But if I got to the tangent bundle $TM$, then I guess I can make that Jacobian dependent on $q$(not calculating it at a point, but just calculating it as a function of $q$), in which case I'd have that $\frac{\partial}{\partial q}$ does depend on both $\frac{\partial}{\partial\dot{q}'}$ and $\frac{\partial}{\partial q'}$, like it is claimed.

Is this reasoning correct?

 

[wrobel]

Summary:: Confusion about coordinate transformation on a tangent bundle

So at the end of that discussion there is an exercise that is(I presume) supposed to show that an equivalent of Poincare 1-form doesn't exist on a tangent bundle.

In tangent bundle there is a canonical vector field:
$$\dot q^i\frac{\partial }{\partial \dot q^i}$$
it is invariant under mappings that preserve tangent bundle structure

(i) is wrong

 

[Antarres]

Okay, so, this canonical vector field is what's defined in (iii). However, can you please elaborate why (i) is wrong? Let's say that (i) is wrong, then we supposedly have, under coordinate change:
$$q'^i = q'^i(q^1, \dots , q^n)$$
implies on the tangent bundle:
$$\dot{q}'^i = \sum_{j} \left(\frac{\partial q'^i}{\partial q^j}\right)\dot{q}^j$$
if (i) is wrong, I suppose that means:
$$\frac{\partial}{\partial q^k} = \sum_{j} \left(\frac{\partial q'^j}{\partial q^k}\frac{\partial}{\partial q'^j} + \frac{\partial \dot{q}'^j}{\partial q^k}\frac{\partial}{\partial \dot{q}'^j}\right) = \sum_j \frac{\partial q'^j}{\partial q^k}\frac{\partial}{\partial q'^j}$$

i.e. the second term vanishes(in doesn't make sense that the first one vanishes). Then let's look at the field defined in (ii).

$$\sum_i \dot{q}^i\frac{\partial}{\partial q^i} = \sum_{i} \left(\sum_j \left(\frac{\partial q^i}{\partial q'^j}\right)\dot{q}'^j \sum_k \left(\frac{\partial q'^k}{\partial q^i}\right)\frac{\partial}{\partial q'^k}\right) = \sum_k \dot{q}'^k\frac{\partial}{\partial q'^k}$$

So then it looks like the field in (ii) is well defined on $TM$, so it would be a second canonical vector field, right? That shouldn't be the case, I think.

 

[Antarres]

Excuse me for double post, but I think I resolved it.

Under coordinate change in $M$: $q'^i = q'^i(q^1, \dots q^n)$, the inverse change would be $q^i = q^i(q'^1, \dots q'^n)$. These changes imply on $TM$:
$$\dot{q}'^i = \sum_j \left(\frac{\partial q'^i}{\partial q^j}\right)(q)\dot{q}^j \quad \Leftrightarrow \quad \dot{q}^i = \sum_j \left(\frac{\partial q^i}{\partial q'^j}\right)(q')\dot{q}'^j$$

From here we have in (i):
$$\frac{\partial}{\partial q^k} = \sum_j \frac{\partial q'^j}{\partial q^k}\frac{\partial}{\partial q'^j} + \frac{\partial \dot{q}'^j}{\partial q^k}\frac{\partial}{\partial\dot{q}'^j}$$
No terms vanish since $\dot{q}'$ has explicit dependence(from the Jacobian) on $q$.

Then we have in (ii):
$$\begin{align}
&\sum_k \dot{q}^k\frac{\partial}{\partial q^k} = \sum_k \sum_j \left(\frac{\partial q^k}{\partial q'^j}\right)(q')\dot{q}'^j \left(\sum_i \frac{\partial q'^i}{\partial q^k}\frac{\partial}{\partial q'^i} + \frac{\partial \dot{q}'^i}{\partial q^k}\frac{\partial}{\partial\dot{q}'^i}\right) \\ &= \sum_j \dot{q}'^j\frac{\partial}{\partial q'^j} + \sum_{ijk}\left(\frac{\partial q^k}{\partial q'^j}\right)\left(\frac{\partial \dot{q}'^i}{\partial q^k}\right)\dot{q}'^j\frac{\partial}{\partial \dot{q}'^i}
\end{align}$$

Here the second term won't vanish for same reasons the second term in (i) didn't vanish.
Meanwhile we have for $\frac{\partial}{\partial \dot{q}}$:
$$\frac{\partial}{\partial \dot{q}^k} = \sum_j \frac{\partial q'^j}{\partial \dot{q}^k}\frac{\partial}{\partial q'^j} + \frac{\partial \dot{q}'^j}{\partial \dot{q}^k}\frac{\partial}{\partial\dot{q}'^j}$$
Here the first term vanishes because $q'^j$ is explicitly independent of $\dot{q}$, while the second term simplifies to:
$$ \frac{\partial \dot{q}'^j}{\partial \dot{q}^k} = \frac{\partial q'^j}{\partial q^k}$$
Hence in (iii) we have:
$$\sum_k \dot{q}^k\frac{\partial}{\partial \dot{q}^k} = \sum_k\sum_j \left(\frac{\partial q^k}{\partial q'^j}\right)\dot{q}'^j \sum_i \left(\frac{\partial q'^i}{\partial q^k}\right)\frac{\partial}{\partial \dot{q}'^i} =\sum_j \dot{q}'^j\frac{\partial}{\partial \dot{q}'^j}$$
So this is well defined, as @wrobel said, it's called canonical vector field. When I said the exercise was supposed to show such a thing doesn't exist, that was my wrong assumption, since I didn't really know what to expect as a solution. Turns out the exercise is supposed to show its existence.

I think my confusion mostly came from losing the count of explicit/implicit dependencies, I didn't see how the second term in (ii) wouldn't vanish, but the first term in (iii) would. I think it's good now, but it would be nice if someone more knowledgeable checked it.

 

[wrobel]

Let's say that (i) is wrong, then we supposedly have, under coordinate change:

implies on the tangent bundle:

I interpret that in (i) the tangent bundle is not considered just the manifold M

 

[wrobel]

By the way, it would be a useful task : show that
$$a_i=\frac{d}{dt}\frac{\partial L}{\partial \dot x^i}- \frac{\partial L}{\partial x^i},\quad L=L(x,\dot x)$$ is transformed as a covector under the change $x^i=x^i(x')$

 

[Antarres]

I meant that the smooth structure on $TM$ is dictated by $M$, so we need to check for coordinate transitions on $TM$ of form:
$$q'^i = q'^i(q^1,\dots , q^n) \quad \dot{q}'^i = \sum_i \frac{\partial q'^i}{\partial q^j}\dot{q}^j$$
Am I wrong? This book is not exactly completely formal, but I guess I could try to formalize that.

Suppose we have an atlas on $M$, consisting of charts $(U_\alpha,\phi_\alpha)$. Taking natural projection from $TM$ to $M$, $\pi(q,\dot{q}) = (q)$, we create the atlas on TM using charts:
$$\phi'_\alpha : \pi^{-1}(U_\alpha) \rightarrow \mathbb{R}^{2n}$$
Here we used that the tangent space at every point is in bijective correspondence to $\mathbb{R}^n$, as well as $M$ itself through the charts.
Then the smoothness condition on chart overlaps $\pi^{-1}(U_\alpha \cap U_\beta)$ is induced from $M$ as well. Correct me where I'm wrong. The transformation of $\dot{q}$ is just the usual transformation of vector component in each tangent space, while the coordinate transformation for $q$ is general.

By the way, it would be a useful task : show that
$$a_i=\frac{d}{dt}\frac{\partial L}{\partial \dot x^i}- \frac{\partial L}{\partial x^i},\quad L=L(x,\dot x)$$ is transformed as a covector under the change $x^i=x^i(x')$

I can do this, it looks analogous to finding out that canonical momenta transform as covectors. I don't see how it's related the issue above though.

 

[wrobel]

For example one can say that a tangent bundle TM={(x,v)∣x∈M,v∈TxM} is a manifold such that
if xi′=xi′(x) are the "gluing" maps in M then
xi′=xi′(x),vi′=∂xi′∂xivi are the "gluing" maps in TM

I don't see how it's related the issue above though.

just another elementary (but important) exercise in the same field