Typo in an example (Probability)

[TheMathNoob]

Homework Statement

RollingDice.
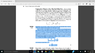
Supposethat 12dice are to be rolled.We shall determine the probability p that each of the six different numbers will appear twice. Each outcome in the sample space S can be regarded as an ordered sequence of 12 numbers, where the ith number in the sequence is the outcome of the ith roll. Hence, there will be 6^12 possible outcomes in S, and each of these outcomes can be regarded as equally probable. The number of these outcomes that would contain each of the six numbers 1, 2,...,6 exactly twice will be equal to the number of differentpossiblearrangementsofthese12elements.Thisnumbercanbedetermined byevaluatingthemultinomialcoefficientforwhichn=12,k=6,andn1=n2=...= n6=2. Hence, the number of such outcomes is
12 choose 2, 2, 2, 2, 2, 2= 12!/ (2!)6 , and the required probability p is
p=12!/ (2!(6)(6^12)) =0.0034

Homework Equations

Multinomial coefficient.

The Attempt at a Solution

Here, the book uses multinomial coefficients to compute the number of outcomes for the given event. The thing is that I really think by heart that the solution is wrong. They are counting the number of outcomes of the sample space using permutation and the number of outcomes of the given event using combination. I really think that the right answer for the number of outcomes for the event is just 12! or another approach to solve this problem using multinomial coefficients is to change the counting method for the sample space, so it would be 72 choose 12. 72 would the total number of faces that will be distributed randomly in a set of 12 units.

 

[andrewkirk]

I really think that the right answer for the number of outcomes for the event is just 12!

I agree with the book.
12! is the number of eligible outcomes if the members of each pair of values are distinguishable, ie if
* allocating the 'first' 6 to roll 8 and the 'second' 6 to roll 5
is distinguishable from
* allocating the 'first' 6 to roll 5 and the 'second' 6 to roll 8
But they are not distinct events in this sample space.

Using 12! implies that they are distinct events. To remove this implication, we need to divide the number of eligible sample space points by the number of ways the two 6s can be distributed between rolls 8 and 5 (or between any other two distinct rolls), which is 2. We then also do that for all the other pairs 1 - 5, hence we end up dividing by $2^6$.

 

[TheMathNoob]

I agree with the book.
12! is the number of eligible outcomes if the members of each pair of values are distinguishable, ie if
* allocating the 'first' 6 to roll 8 and the 'second' 6 to roll 5
is distinguishable from
* allocating the 'first' 6 to roll 5 and the 'second' 6 to roll 8
But they are not distinct events in this sample space.

Using 12! implies that they are distinct events. To remove this implication, we need to divide the number of eligible sample space points by the number of ways the two 6s can be distributed between rolls 8 and 5 (or between any other two distinct rolls), which is 2. We then also do that for all the other pairs 1 - 5, hence we end up dividing by $2^6$.

in the sample space, they are distinguishable. 6^12 implies permutation.

 

[andrewkirk]

No they are not distinguishable, and $6^{12}$ is not a permutation formula. I think this is where you are getting confused.

The sample space is built up from the point of view of the roll. For each roll there can be a number in 1-6, so the sample space has $6^{12}$ elements. Each element is a sequence of 12 numbers, each of which is in {1,2,...,6}

The allocation of pairs comes at it from the other direction, from the point of view of the pair. It starts with six pairs 1-1, 2-2, ..., 6-6 and assumes that the members of each pair are distinguishable as a 'first' and 'second' element, so that there are 12 distingushable elements. Those elements are then allocated to the 12 rolls. Now imagine that we have labelled our two sixes as 6a and 6b and that the pair are allocated to rolls 11 and 12.

Consider the following two allocations to the 12 rolls

(1) 1a 1b 2a 2b 3a 3b 4a 4b 5a 5b 6a 6b
(2) 1a 1b 2a 2b 3a 3b 4a 4b 5a 5b 6b 6a

In the sample space these are both just the element 1 1 2 2 3 3 4 4 5 5 6 6
So we have to adjust our number of different allocations of pairs to take account of the fact that allcoating 6a and 6b to rolls m and n is part of the same sample space element as allocating them to n and m. THat's why we divide by $2^6$.

Edit: It may help if I add that permutation formulas like 12! are used for sampling without replacement whereas power formulas like $6^{12}$ are used for sampling with replacement.

 

[TheMathNoob]

No they are not distinguishable, and $6^{12}$ is not a permutation formula. I think this is where you are getting confused.

The sample space is built up from the point of view of the roll. For each roll there can be a number in 1-6, so the sample space has $6^{12}$ elements. Each element is a sequence of 12 numbers, each of which is in {1,2,...,6}

The allocation of pairs comes at it from the other direction, from the point of view of the pair. It starts with six pairs 1-1, 2-2, ..., 6-6 and assumes that the members of each pair are distinguishable as a 'first' and 'second' element, so that there are 12 distingushable elements. Those elements are then allocated to the 12 rolls. Now imagine that we have labelled our two sixes as 6a and 6b and that the pair are allocated to rolls 11 and 12.

Consider the following two allocations to the 12 rolls

(1) 1a 1b 2a 2b 3a 3b 4a 4b 5a 5b 6a 6b
(2) 1a 1b 2a 2b 3a 3b 4a 4b 5a 5b 6b 6a

In the sample space these are both just the element 1 1 2 2 3 3 4 4 5 5 6 6
So we have to adjust our number of different allocations of pairs to take account of the fact that allcoating 6a and 6b to rolls m and n is part of the same sample space element as allocating them to n and m. THat's why we divide by $2^6$.

Edit: It may help if I add that permutation formulas like 12! are used for sampling without replacement whereas power formulas like $6^{12}$ are used for sampling with replacement.

Yeah, maybe permutation is not the right word, but I think that when you do sampling with replacement, order is taken into account. I imagine 1-1,2-2,3-3,4-4,5-5,6-6 as 12 distinguishable elements. What I am going to do next is how I see the problem.

- - - - - - - - - - - -
I assume that those slots are dices, so to find the number of outcomes you put in each slot the number of possibilities that this dice can have, so it would be

6 6 6 6 6 6 6 6 6 6 6 6

Then the total number of outcomes would 6 ^12 and there order matters.

 

[TheMathNoob]

No they are not distinguishable, and $6^{12}$ is not a permutation formula. I think this is where you are getting confused.

The sample space is built up from the point of view of the roll. For each roll there can be a number in 1-6, so the sample space has $6^{12}$ elements. Each element is a sequence of 12 numbers, each of which is in {1,2,...,6}

The allocation of pairs comes at it from the other direction, from the point of view of the pair. It starts with six pairs 1-1, 2-2, ..., 6-6 and assumes that the members of each pair are distinguishable as a 'first' and 'second' element, so that there are 12 distingushable elements. Those elements are then allocated to the 12 rolls. Now imagine that we have labelled our two sixes as 6a and 6b and that the pair are allocated to rolls 11 and 12.

Consider the following two allocations to the 12 rolls

(1) 1a 1b 2a 2b 3a 3b 4a 4b 5a 5b 6a 6b
(2) 1a 1b 2a 2b 3a 3b 4a 4b 5a 5b 6b 6a

In the sample space these are both just the element 1 1 2 2 3 3 4 4 5 5 6 6
So we have to adjust our number of different allocations of pairs to take account of the fact that allcoating 6a and 6b to rolls m and n is part of the same sample space element as allocating them to n and m. THat's why we divide by $2^6$.

Edit: It may help if I add that permutation formulas like 12! are used for sampling without replacement whereas power formulas like $6^{12}$ are used for sampling with replacement.

1 more thing, I think that if you want to find the total number of outcomes in the sample space not taking into account order then what you would do is to sum the total number of faces of the dices which is 72 because 6 * 12 is 72 then you apply the combinatorial equation which is 72 choose 12

 

[andrewkirk]

You are confusing
-order of dice rolls
with
- order of allocation of pair members to rolls.
The first order matters. The second does not.

 

[TheMathNoob]

You are confusing
-order of dice rolls
with
- order of allocation of pair members to rolls.
The first order matters. The second does not.

Thank you so much, I finally got it.

 

[Ray Vickson]

Yeah, maybe permutation is not the right word, but I think that when you do sampling with replacement, order is taken into account. I imagine 1-1,2-2,3-3,4-4,5-5,6-6 as 12 distinguishable elements. What I am going to do next is how I see the problem.

- - - - - - - - - - - -
I assume that those slots are dices, so to find the number of outcomes you put in each slot the number of possibilities that this dice can have, so it would be

6 6 6 6 6 6 6 6 6 6 6 6

Then the total number of outcomes would 6 ^12 and there order matters.

If it helps, think of rolling a single die 12 (independent) times, and asking for the probability that we have two of each face value in the outcome string. One die, 12 times, or 12 dice once---they are, basically, the same.

Now in the one die 12 times scenario, the multinomial distribution is clearly the one to use---there are no "replacements" occurring, just 12 independent tosses.