Understanding the concept of Probability distribution

[chwala]

Homework Statement

see attached

Relevant Equations

statistics

Consider the attachment below;

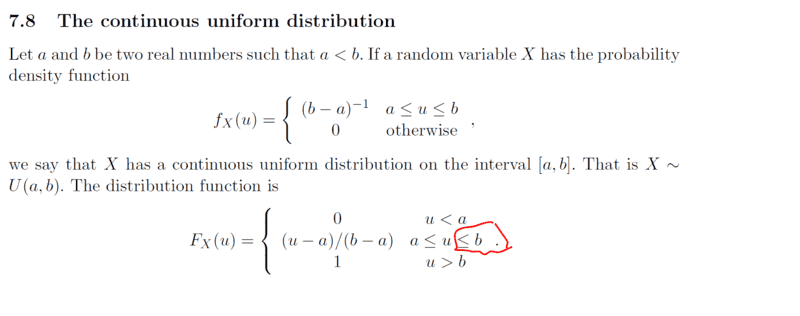
How did they arrive at

$F_X (u) = \dfrac{u-a}{b-a}$ ?

I think there is a mistake on the inequality, probably its supposed to be $a≤u<b$ and that will mean;

$$F_X (u) =\dfrac{1}{b-a} \int_a^u du= \dfrac{1}{b-a} ⋅(u-a)$$ as required. Your thoughts...then i have the second part of the question that i will post here after the analysis.

 

[Office_Shredder]

What do you think goes wrong if you include b? What is $F(b)$ supposed to be, and what does it become?

 

[chwala]

What do you think goes wrong if you include b?

It will be equal to $1$.

 

[Orodruin]

It will be equal to $1$.

And why would that be a problem?

Do note that the definition of $F_X$ would result in exactly the same function if you instead defined the middle case on the interval $[a,b)$ and the third on $[b, \infty)$ because both expressions are equal to one at $u = b$.

 

[chwala]

And why would that be a problem?

Do note that the definition of $F_X$ would result in exactly the same function if you instead defined the middle case on the interval $[a,b)$ and the third on $[b, \infty)$ because both expressions are equal to one at $u = b$.

Ok, i hear you...then are my steps in post $1$ correct with the given limits? if not then how did they arrive at the solution.

 

[chwala]

And why would that be a problem?

Do note that the definition of $F_X$ would result in exactly the same function if you instead defined the middle case on the interval $[a,b)$ and the third on $[b, \infty)$ because both expressions are equal to one at $u = b$.

My intention is to get the shown $F_X(u)$ from the given probability density function $f_x(u)=(b-a)^{-1}$. This is where my problem is.

 

[chwala]

This is the continuation of the same problem...clearly textbook mistake on $E(x)$ value i.e the highlighted in red...Anyway, my approach on this problem;

$$E(x)=\dfrac{1}{b-a} \int_a^b u du= \left[\dfrac{b^2-a^2}{2} ⋅\dfrac{1}{b-a}\right]=\left[\dfrac{(b+a)(b-a)}{2(b-a)}\right]=\dfrac{b+a}{2}$$$$Var(x)=\dfrac{1}{b-a} \int_a^b u^2 du=\left[\dfrac{b^3-a^3}{3} ⋅\dfrac{1}{b-a}\right]=\left[\dfrac{b^2+a^2+ab}{3}-\dfrac{(b+a)^2}{4}\right]$$

$$=\left[\dfrac{(4b^2+4a^2+4ab)-(3b^2+6ab+3a^2)}{12}\right]$$

$$=\left[\dfrac{b^2+a^2-2ab}{12}\right]= \dfrac{(b-a)^2}{12}$$

if there is a better approach will appreciate. Cheers guys!