What is the best way to determine the distributed load for a cargo shelf?

[kleptomaniac]

Hello forum,
I'm hoping i can get some help figuring something out. I'm working on a project which requires a 4 wheel trolley frame to be designed to transport cargo. The basic dimensions of the trolley I've already determined based on the required need and ergonomic guidelines etc. Now i need to design the members and select a suitable material for the various components.

I've been completing some of my frame analysis calculations based on some assumptions about the cargo's load distribution, which on further thought, i think may be wrong.

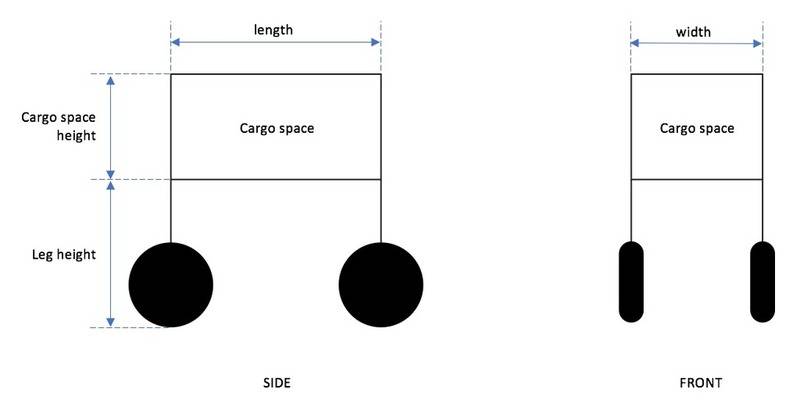
Essentially, I've determined that the trolley will need to transport a maximum of 300kg of cargo, which when fully loaded, will take up the full surface area of the cargo shelf (and most of the provided cargo space height). A basic representation of the trolley is shown below, which is basically a (top) open box on wheels (not including the horizontal push handle). For reference, length = 0.54m, width = 0.36m, cargo space height = 0.25m, and leg height = 0.65m.

Currently, I've simply assumed that the weight of the cargo (mg = 300*9.81 = 2943N) on the cargo shelf "beam" is applied at the half length where its Center of Gravity (CG) would be. However the more i think about this the less sense it makes, as the cargo (let's assume a uniform large rectangular mass that fits snugly within the cargo space) has a significantly large contact surface area with the cargo shelf that it should really be evenly distributed across the length.

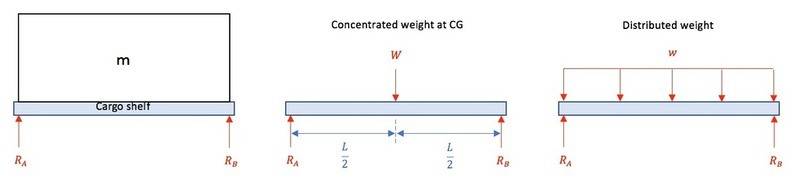
The cargo shelf is essentially a supported beam as shown in the image above.
My question now is, what would the distributed load (little w) be? Would it:

1. simply be the weight (big W) of the cargo (W = 2943N) spread across the length?
2. or would the distributed load need to be calculated based on the weight of the mass and the length?

At first i thought the distributed w load would just be the weight divided by the length of the beam. So:

Distributed load w = W/l = 2943N/0.54m = 5451N/m​

This would make the most sense considering the castor/wheel load capacity will be at least 1/4 the cargo mass (300/4 = 75kg per castor). Which matches the calculated reaction forces at the supports based on this distributed load assumption.
However I'm still not certain if there's a better way of determining the distributed load of a mass with sufficiently large contact surface area (something tells me the full contact surface area should come in at some point). My google search hasn't turned up anything useful thus far other than the usual "distributed load to concentrated load" conversion equations.

The load type matters when determining the shear and moment diagram for the beam, which is why I've gone back to think it through again.

 

[PhanthomJay]

the 5451N/m is correct, it accounts for the shelf width which must be used in the stress calculations for determining the beam's x-section area and Section Modulus. Or you can look at it as 2943 N spread over a .36 X .54 sq meter area, or 15,138 N/m^2 loading , which is 15,138(.36) = 5,451 N/m over length of beam.

 

[kleptomaniac]

the 5451N/m is correct, it accounts for the shelf width which must be used in the stress calculations for determining the beam's x-section area and Section Modulus. Or you can look at it as 2943 N spread over a .36 X .54 sq meter area, or 15,138 N/m^2 loading , which is 15,138(.36) = 5,451 N/m over length of beam.

Sweet, thanks. That makes sense.
I started another thread for another problem for the same trolley. Hopefully i can get some feedback on that one too.

 

[JBA]

How you distribute the loading depends on the configuration of the beams you use to support the bottom plate. If you use only peripheral beams then the above area method is best and then the loading is distributed to the beams based upon the adjoining area segments created by drawing cross lines from corner to corner across the base; however, under this arrangement a flat diaphragm plate analysis is also required to determine stresses in the unsupported region of the bottom plate that will cause transverse bending in the perimeter beams due to the plate tension under load.

On the other hand, if you support the bottom plate by a series of of parallel longitudinal beams connected to the two end beams then the distributed loading can be divided evenly between the longitudinal beams; but their connections to the end transverse beams is not a distributed loading, it is a series of point loadings along the the end beam with each point load equal to one half of the connected longitudinal beam's total load; and, visa versa for a series of cross beams connected to the longitudinal beams.