- #1
ChiralSuperfields
- 1,206
- 132
- Homework Statement
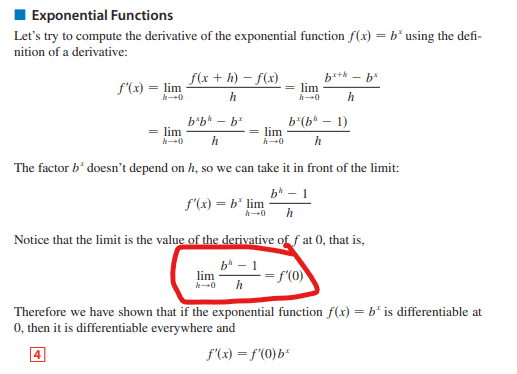
- I am trying to understand why as h approach's zero ##\frac{b^h - 1}{h} = f'(0)##. Dose anybody please know of a good way to explain this? Many thanks!
- Relevant Equations
- Pls see below
Thank you for your reply @FactChecker !FactChecker said:That is the definition of the derivative of ##f(x) = b^x## at ##x=0##. Notice that they do not say there that the limit and the derivative exist. In fact, the last sentence says "if the exponential function ##f(x) = b^x## is differentiable" (emphasis mine). So there is still something to prove.
You have formula wrong. I think that ##\lim_{h \rightarrow 0} {\frac {f( x+h) - f(x)}{h}} = f'(x)## is a common definition of the derivative. Is that your definition of the derivative? Now plug in ##x=0##.Callumnc1 said:How dose ##\lim_{x \rightarrow h} {\frac {b^h - 1} {h}} = f'(0)##?
Callumnc1 said:Homework Statement:: I am trying to understand why as h approach's zero ##\frac{b^h - 1}{h} = f'(0)##. Dose anybody please know of a good way to explain this? Many thanks!
Relevant Equations:: Pls see below
Thank you for your reply @FactChecker!FactChecker said:You have formula wrong. I think that ##\lim_{h \rightarrow 0} {\frac {f( x+h) - f(x)}{h}} = f'(x)## is a common definition of the derivative. Is that your definition of the derivative? Now plug in ##x=0##.
Thank you for your reply @SammyS!SammyS said:First: The word is "Please", not Pls .
Also, you've been misspelling the word "Does". It is not "Dose".
So, you're back to posting a very detailed explanation of some math or physics topic, then asking some question regarding a detail which has been very well explained.
The best answer I can give as to why ##\displaystyle f'(0)=\lim_{h\to 0} \dfrac{b^h - 1}{h} ## is because ##\displaystyle b^0 = 1## .
Actually, you went too far. You asked this:Callumnc1 said:Thank you for your reply @FactChecker!
If I plug in x = 0, to the definition of derivative (the expression that you mentioned, I get
##\frac{0}{0} = undefined ##. I am not sure where to go from here?
Many thanks!
Plug in ##x=0## to get the definition ##f'(0) =\lim_{h \rightarrow 0} \frac{b^{0+h} - b^0}{h} = \lim_{h \rightarrow 0}\frac {b^h - 1}{h}##.Callumnc1 said:Homework Statement:: I am trying to understand why as h approach's zero ##\frac{b^h - 1}{h} = f'(0)##.
That's not how to evaluate this limit.Callumnc1 said:Thank you for your reply @SammyS!
But plugging in h = 0 gives ##\frac{0}{0} = undefined##. How can ##f'(0)## be undefined since it is not a number?
Many thanks!
The general formula for computing the derivative of an exponential function is f'(x) = ax * ln(a), where a is the base of the exponential function.
Yes, the derivative of an exponential function can be negative. This occurs when the base of the exponential function is between 0 and 1, and the value of x is negative.
The derivative of an exponential function can be used to model growth and decay in various fields such as finance, biology, and physics. It can also be used to calculate rates of change in exponential processes.
Yes, there are special rules for computing the derivative of an exponential function. These include the power rule, product rule, and chain rule. It is important to understand these rules in order to accurately compute the derivative.
Yes, there are certain properties of exponential functions that can make the process of computing the derivative simpler. These include the fact that the derivative of an exponential function with base e is equal to the function itself, and the fact that the derivative of ax is equal to ax * ln(a).