- #1
tomdodd4598
- 138
- 13
Hi there,
Over the last couple of weeks, I have been learning about the relativistic description of electromagnetism through Leonard Susskind's Theoretical Minimum lectures, and although I have managed to follow it, there are some parts which I am becoming increasingly confused by, not helped by the fact Susskind uses the (-+++) convention rather than the seemingly more popular (+---).
Is the definition of the electromagnetic tensor different depending on the convention? I am aware that the matrix changes (the contravariant and covariant forms are multiplied by -1 when moving between conventions), but does the definition of each component in terms of the vector potential change?
The reason I ask this is because when deriving the relativistic Lorentz force from the Lagrangian:
L=-m√(-dXϑ/dt dXϑ/dt)-qAϑ dXϑ/dt
I always seem to get the following answer (using the (-+++) convention):
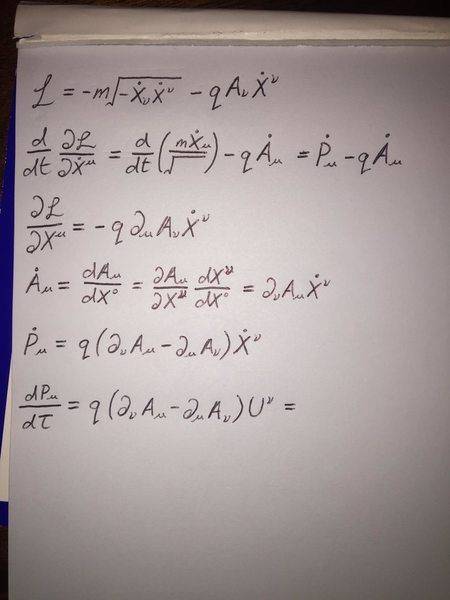
Which is the negative of what I would expect from the definition of the Electromagnetic Tensor.
Any help would be appreciated, and thanks in advance.
Over the last couple of weeks, I have been learning about the relativistic description of electromagnetism through Leonard Susskind's Theoretical Minimum lectures, and although I have managed to follow it, there are some parts which I am becoming increasingly confused by, not helped by the fact Susskind uses the (-+++) convention rather than the seemingly more popular (+---).
Is the definition of the electromagnetic tensor different depending on the convention? I am aware that the matrix changes (the contravariant and covariant forms are multiplied by -1 when moving between conventions), but does the definition of each component in terms of the vector potential change?
The reason I ask this is because when deriving the relativistic Lorentz force from the Lagrangian:
L=-m√(-dXϑ/dt dXϑ/dt)-qAϑ dXϑ/dt
I always seem to get the following answer (using the (-+++) convention):
Which is the negative of what I would expect from the definition of the Electromagnetic Tensor.
Any help would be appreciated, and thanks in advance.