- #1
gionole
- 281
- 24
- TL;DR Summary
- How is surface charge accumulated with battery connected, but circuit still open
let's discuss copper wire all along so we only focus on the specific wire. Let's discuss 2 cases(case 1 is hopefully correct, so if it is, would be good to mention it).
I'm sorry that this text got so bigger(didn't expect it), but wanted to mention my thought process. Somehow, none of the videos/articles explain the below. They all explain why surface charges must exist because if they don't, E won't be constant. Maybe they do, but then I'm not understanding it. My questions and theorem are about how they're produced in the first place. Hope you read it and let me know where I'm making mistakes.
Case 1: We only have copper wire which is not connected to battery or anything else. It's just wire and thats it. In this case, we know there's no surface charges presented on it and the situation is it has got copper atoms inside it in which 1 valence electron from each atom kind of jumps around back and forth within some small area due to electric field of its neighbouring atoms' protons' electric fields. Due to this, electrons do actually move, but within small area and overally, their drift velocity is 0.
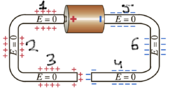
Case 2: We connect the wire to battery. Note that we don't close the circuit. Well, in this case, what we know is atoms have been arranged to become surface charges and in this case, E inside wire is 0 everywhere. I wonder about this case and will mention what I'm curious.
So in the wire, there were copper atoms that for a sec, become positive ions, then another sec, become neutral atoms due to electron "seas" jumping into and from it all the time. but when we connected it to the battery(circuit is still open), it's said that on the positive terminal end, wire has positive charges on the surface accumulated, while on the negative terminal end, wire has negative charges on the surface. Could you explain how this happened ? what exactly caused this and why are more surface charges near the terminals of the battery and why they decrease over distance of the wire ?
My Theorem: Maybe what happens is battery's positive terminal's positive charges attract free electrons that are very close to them which causes the wire part that's very close to the terminal to have more positive ions(before battery, it didn't, but now it has). Since positive ions appeared(in the wire, there're more positive ions now than free electrons while before - their count was the same) in the wire near the part of terminal, 2 things take effect now:
The good questions now(if ofc, my theorem is right) would be the following:
Please note that I'm only discussing the case during my theorem and in questions(before wire is connected to the battery and when wire is connected to battery but here circuit is still open - the sides of the wires are not connected to each other).
Question 1: In my explanation, what would you add so that it explains why surface charges are much less the more you go through the wire from positive terminal ?
Question 2: I mentioned that positive terminal of the battery since it had positive charges, when it got connected to wire, it caused some of the nearby free electrons of the wire to be attracted to it, so I guess these free electrons move to battery and stay there ? Which in the end means some of the free electrons completely disappeared from the wire. This seems more logical than to say that free electron and battery's positive charge both go to each other to meet (I think this is wrong as in the positive terminal, there's Cu2+ ion so definitely it's attracting free electrons). So these free electrons move to battery and Cu2+ become Cu. (hopefully I'm explaining this well enough - all these happens before circuit is closed). Thoughts whether this is correct ?
Question 3: Well, I was thinking now how the negative charges end up on the surface on the negative terminal side. Well, on the battery's negative end, we got negative charges or maybe Zn metal that's so eager to lose its electrons. Nearby atoms of the wire definitely can cause these electrons of the battery to get into the wire which logically seems that in the negative terminal's side, the wire has more negative charge(before connecting it to battery, there was the same number of free electrons and positive ions in the wire, but after connecting the wire to battery, as I said, some electrons from battery hopped into the wire, and there're more negative charges overally. I believe this causes the nearby wire's charges to start repelling each other and they end up on the surface. But I have the same question here as in the Question 1: why exactly negative surface charges are less the more you go through the wire ?
I'm sorry that this text got so bigger(didn't expect it), but wanted to mention my thought process. Somehow, none of the videos/articles explain the below. They all explain why surface charges must exist because if they don't, E won't be constant. Maybe they do, but then I'm not understanding it. My questions and theorem are about how they're produced in the first place. Hope you read it and let me know where I'm making mistakes.
Case 1: We only have copper wire which is not connected to battery or anything else. It's just wire and thats it. In this case, we know there's no surface charges presented on it and the situation is it has got copper atoms inside it in which 1 valence electron from each atom kind of jumps around back and forth within some small area due to electric field of its neighbouring atoms' protons' electric fields. Due to this, electrons do actually move, but within small area and overally, their drift velocity is 0.
Case 2: We connect the wire to battery. Note that we don't close the circuit. Well, in this case, what we know is atoms have been arranged to become surface charges and in this case, E inside wire is 0 everywhere. I wonder about this case and will mention what I'm curious.
So in the wire, there were copper atoms that for a sec, become positive ions, then another sec, become neutral atoms due to electron "seas" jumping into and from it all the time. but when we connected it to the battery(circuit is still open), it's said that on the positive terminal end, wire has positive charges on the surface accumulated, while on the negative terminal end, wire has negative charges on the surface. Could you explain how this happened ? what exactly caused this and why are more surface charges near the terminals of the battery and why they decrease over distance of the wire ?
My Theorem: Maybe what happens is battery's positive terminal's positive charges attract free electrons that are very close to them which causes the wire part that's very close to the terminal to have more positive ions(before battery, it didn't, but now it has). Since positive ions appeared(in the wire, there're more positive ions now than free electrons while before - their count was the same) in the wire near the part of terminal, 2 things take effect now:
- a. these positive ions try to repel from each other.
- b. these positive ions try to attract free electrons that are in the later part of the wire.
The good questions now(if ofc, my theorem is right) would be the following:
Please note that I'm only discussing the case during my theorem and in questions(before wire is connected to the battery and when wire is connected to battery but here circuit is still open - the sides of the wires are not connected to each other).
Question 1: In my explanation, what would you add so that it explains why surface charges are much less the more you go through the wire from positive terminal ?
Question 2: I mentioned that positive terminal of the battery since it had positive charges, when it got connected to wire, it caused some of the nearby free electrons of the wire to be attracted to it, so I guess these free electrons move to battery and stay there ? Which in the end means some of the free electrons completely disappeared from the wire. This seems more logical than to say that free electron and battery's positive charge both go to each other to meet (I think this is wrong as in the positive terminal, there's Cu2+ ion so definitely it's attracting free electrons). So these free electrons move to battery and Cu2+ become Cu. (hopefully I'm explaining this well enough - all these happens before circuit is closed). Thoughts whether this is correct ?
Question 3: Well, I was thinking now how the negative charges end up on the surface on the negative terminal side. Well, on the battery's negative end, we got negative charges or maybe Zn metal that's so eager to lose its electrons. Nearby atoms of the wire definitely can cause these electrons of the battery to get into the wire which logically seems that in the negative terminal's side, the wire has more negative charge(before connecting it to battery, there was the same number of free electrons and positive ions in the wire, but after connecting the wire to battery, as I said, some electrons from battery hopped into the wire, and there're more negative charges overally. I believe this causes the nearby wire's charges to start repelling each other and they end up on the surface. But I have the same question here as in the Question 1: why exactly negative surface charges are less the more you go through the wire ?
Last edited: