Self lock: conditions to make it work

[Karol]

Homework Statement

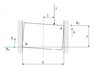
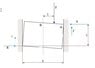
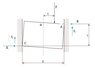
The flat piece is between 2 walls and is pushed eccentrically by force F. what should be the relation between the width x and the height y in order to achieve self locking (that the piece won't go down)?

Homework Equations

Friction Force: f=μN
Balance of moments: F₁L₁=F₂L₂

The Attempt at a Solution

I make 2 equations of moments around the contact points with the walls. the friction coefficient is μ. round point A:
$$F\left(\frac{x}{2}-l\right)+yR_2=\mu R_1\rightarrow R_2=\frac{F\left(\frac{x}{2}-l\right)}{\mu x-y}$$
round point B:
$$F\left(\frac{x}{2}+l\right)=yR_1+\mu xR_1\rightarrow R_1=\frac{F\left(\frac{x}{2}+l\right)}{y+\mu x}$$
First i see that R₁≠R₂, is it true?
I now insert R₁ and R₂ in the forces equation with the inequality $F\geq\mu(R_1+R_2)$ and get:
$$\frac{2}{\mu}\geq (x-2l)(\mu x+y)+(x+2l)(\mu x-y)$$
I can't get a conclusion from that. where, in which book, is this subject covered? i feel i complicated it

 

[mfb]

R1 and R2 should be the same, otherwise your horizontal forces are not balanced.
It is possible to simplify the result (didn't check it) a bit.
You can solve this inequality for x or (easier) y.

 

[Karol]

O.K. I take moments around point A (new drawing):
$$F\left(\frac{x}{2}-l\right)+yR=\mu R x\rightarrow R=\frac{F\left(\frac{x}{2}-l\right)}{\mu x-y}$$
While moments around B give: $Fl=Ry\rightarrow R=\frac{Fl}{y}$
The first equation involves μ. i think she's wrong, but i don't know why. also in that equation if l=0 still R exists and it's wrong

 

[mfb]

Ah, I think I see the problem: The force from friction does not have to be µ times the horizontal force - this is just the maximum value.

 

[Karol]

The force from friction does not have to be µ times the horizontal force - this is just the maximum value.

Right so i cannot take moments around A? but i am interested in the limit just before the sliding, then it is the maximum force, i am interested to find geometrical relations between x, y and l so it will just lock.
And it is strange that around point B the R forces balance the moment from F and around A it is the frictional force which balances even a greater moment added by R

 

[mfb]

You can, and the self-locking requirement probably means the frictional force becomes maximal at one side (not at both), but you don't know which one in advance.

 

[Karol]

How come the frictional force becomes maximal at one side only if R is the same on both sides? and even if it does it won't prevent from sliding since i made F=f₁+f₂=2μR. you need both sides to be the maximum.

 

[haruspex]

You can, and the self-locking requirement probably means the frictional force becomes maximal at one side (not at both), but you don't know which one in advance.

I think it's easy to determine which. Imagine there were no friction at the lower point and consider torque about the other. Could the system remain static? Now consider no friction at the higher point, etc.

 

[mfb]

I didn't say it is hard, and considering torque around the center of the object will lead to the right answer.

In general friction should appear on both sides, I'm not sure if analyzing the setup with zero friction leads to the same result.

 

[haruspex]

I didn't say it is hard, and considering torque around the center of the object will lead to the right answer.

In general friction should appear on both sides, I'm not sure if analyzing the setup with zero friction leads to the same result.

Thinking what would happen without friction at some point can be a useful way of figuring out which way friction acts there.

 

[Karol]

But why no friction at all, at any side? the friction maximizes on one side earlier, as you say, so there is no relevance to no friction, it doesn't happen.
And my initial question was what are relations between x and y to achieve self locking. and also on post #3 i showed moments around different points that lead to two different answers.

 

[haruspex]

But why no friction at all, at any side? the friction maximizes on one side earlier, as you say, so there is no relevance to no friction, it doesn't happen.
And my initial question was what are relations between x and y to achieve self locking. and also on post #3 i showed moments around different points that lead to two different answers.

The zero friction (one side at a time, not both sides at once) was a thought experiment to establish which of the two contacts would necessarily be at limiting friction. There may in general be friction at the other point also.
In your post #3 there are a couple of problems with your equation for moments about B. The left hand side should be F(x/2+l). There is an unknown frictional force at A.
It's a tricky problem because you are looking for a condition such that it won't slip no matter how large F is.

 

[Karol]

In your post #3 there are a couple of problems with your equation for moments about B. The left hand side should be F(x/2+l). There is an unknown frictional force at A.

I don't think there should be F(x/2+l) on the left side. i wrote $Fl=Ry\rightarrow R=\frac{Fl}{y}$ and i think it's true. and the two equal frictional forces make moments around B that cancel each other. in post #3 i attached a new drawing in which i moved point B, you probably still looked at the original drawing from post #1

 

[haruspex]

I don't think there should be F(x/2+l) on the left side. i wrote $Fl=Ry\rightarrow R=\frac{Fl}{y}$ and i think it's true. and the two equal frictional forces make moments around B that cancel each other. in post #3 i attached a new drawing in which i moved point B, you probably still looked at the original drawing from post #1

You're right, I didn't notice you had moved B. I assume it is meant to be at the same height as A.
As I keep saying, you cannot assume the two frictional forces are the same.

I added an edit to my previous post that got lost somehow. There are only three equations to be had in a statics problem with one moveable object. If you write two equations for linear statics and two for moments about different points then the four equations will be linearly dependent.

 

[mfb]

It's a tricky problem because you are looking for a condition such that it won't slip no matter how large F is.

All forces scale with F as we neglect the mass of the object, fixing it would not change the problem.

 

[haruspex]

All forces scale with F as we neglect the mass of the object, fixing it would not change the problem.

Yes, but I feel it makes it hard to think about.

 

[Karol]

The zero friction (one side at a time, not both sides at once) was a thought experiment to establish which of the two contacts would necessarily be at limiting friction. There may in general be friction at the other point also.

Why does this condition of zero friction determine on which side is the limiting friction and what is a limiting friction, is it the maximum friction f_max=μR ?
And i cancel the friction one side at a time and take moments on the other, opposite, contact point and find that both friction forces aren't needed, the moment from F could be balanced with R only.

 

[mfb]

F and R only do not satisfy the vertical force balance.

 

[Karol]

Yes, so the thought experiment of the 0 friction doesn't help since at both points the other friction is needed, they are both needed

 

[haruspex]

Yes, so the thought experiment of the 0 friction doesn't help since at both points the other friction is needed, they are both needed

They may both be needed, but only one needs to be at maximum value. The point of the thought experiment is to tell you which one. Then you can work out the frictional force at the other (if that's of interest).

 

[Karol]

I uploaded a picture. i marked the lower left contact point with C and i take moments around it, assuming the frictional force f₁ on the opposite corner is the maximum f₁=μR:
$$F\left(\frac{x}{2}+l\right)=yR+\mu Rx\rightarrow R=\frac{F\left(\frac{x}{2}+l\right)}{y+\mu x}$$
If y gets bigger R diminishes and it's true, but if x grows R diminishes also and it's wrong.
If i take moments around A and assume the other frictional force is at the maximum f₂=μR:
$$F\left(\frac{x}{2}-l\right)+Ry=\mu Rx\rightarrow R=\frac{F\left(\frac{x}{2}-l\right)}{\mu x-y}$$
That one isn't logical because of more reasons:
1. as y grows R grows. false
2. as x grows R lessens. false
3. if F acts outside, meaning $\frac{x}{2}<l$ R changes direction

 

[haruspex]

If i take moments around A and assume the other frictional force is at the maximum f₂=μR:
$$F\left(\frac{x}{2}-l\right)+Ry=\mu Rx\rightarrow R=\frac{F\left(\frac{x}{2}-l\right)}{\mu x-y}$$
That one isn't logical because of more reasons:
1. as y grows R grows. false
2. as x grows R lessens. false
3. if F acts outside, meaning $\frac{x}{2}<l$ R changes direction

I'm not following your logic.
I've changed my mind about what makes this problem tricky - it's the role of R. The trouble is, you've no way to find R, yet it is critical that it is sufficiently large. In a sense, the problem is insufficiently specified. The only way to solve it is to suppose that R can be arbitrarily large, increasing as necessary as F increases. (The same problem arises in analysing an object pushed into a narrow angle between two fixed frictional surfaces. The normal force can be arbitrarily large, it just depends on how tightly the object was pushed into the angle initially.)
So, you have to look for the relationship between x, y and mu which allows a sufficiently large R to hold everything in place. The two equations in your last post give you that.

Edit: I have doubts about what I wrote above. Will post more thoughts.

 

[Karol]

In order to maintain the inward direction of R, in calculating moments around A:
$$R=\frac{F\left(\frac{x}{2}-l\right)}{\mu x-y}\rightarrow \left\{ \begin {array}{ccc}\mbox{if }\frac{x}{2}<l, & \mu x<y & \surd \\ \mbox{if }\frac{x}{2}>l, & \mu x >y & \otimes \end{array}\right.$$
I don't understand what i got. The first condition says that if F is outside, the piece must be high (big y) and narrow, and if F is inside, the piece must be wide (large x). are there really 2 conditions for that? why does the application point of F make a difference?
I calculated moments when F is outside, indeed the equations differ a bit but the final result is the same.
In what i am really interested is a condition for the piece to move, not to lock, so how do i interpret the results?
This formula was from moments only, so the results, if i didn't made a logical mistake, enable F to be balanced, so what? what happens if i don't stand in the conditions, what does it mean that R's direction changes? such thing, i think, cannot happen in any case

 

[haruspex]

In order to maintain the inward direction of R, in calculating moments around A:
$$R=\frac{F\left(\frac{x}{2}-l\right)}{\mu x-y}\rightarrow \left\{ \begin {array}{ccc}\mbox{if }\frac{x}{2}<l, & \mu x<y & \surd \\ \mbox{if }\frac{x}{2}>l, & \mu x >y & \otimes \end{array}\right.$$
I don't understand what i got. The first condition says that if F is outside, the piece must be high (big y) and narrow, and if F is inside, the piece must be wide (large x). are there really 2 conditions for that? why does the application point of F make a difference?
I calculated moments when F is outside, indeed the equations differ a bit but the final result is the same.
In what i am really interested is a condition for the piece to move, not to lock, so how do i interpret the results?
This formula was from moments only, so the results, if i didn't made a logical mistake, enable F to be balanced, so what? what happens if i don't stand in the conditions, what does it mean that R's direction changes? such thing, i think, cannot happen in any case

I don't know why you mark the second result, $\mu x > y$ as wrong.

Let's go back to the two equations we're sure of,
1 $F(x/2+L) = Ry+F_1x$
2 $F(x/2-L) = F_2x-Ry$
From the geometry, it cannot slip at the top right corner unless it also slips at the bottom left. So we can assume $F_2=\mu R$.
You can then obtain an equation which involves neither F₂ nor R. Let F₁ = c F. You can then get mu in terms of c, x, y and L.
What is the range of possible values for c?

 

[Karol]

From the geometry, it cannot slip at the top right corner unless it also slips at the bottom left. So we can assume $F_2=\mu R$.

Why? i can assume with the same reasoning that $F_1=\mu R$

You can then obtain an equation which involves neither F2 nor R

I use my equations with the assumption $F_2=\mu R$:
$$\left\{\begin {array}{l}R=\frac{F\left(\frac{x}{2}-l\right)}{\mu x-y}\\F\left(\frac{x}{2}+l\right)=yR+f_1x\end{array}\right.$$
$$\rightarrow f_1=F\left(\frac{y-\mu\left(\frac{x}{2}+l\right)}{y-\mu x}\right)$$
$$f_1<F\rightarrow l+\frac{x}{2}<x$$
First, that is how i drew the sketch with F inside, it doesn't say something new, and secondly it's a restraining condition, what if F is outside?

 

[haruspex]

Why? i can assume with the same reasoning that F1=μRF_1=\mu R

No. From the geometry, it could slip down at the lower left corner while pivoting at the top right. The converse is not true.

$\rightarrow f_1=F\left(\frac{y-\mu\left(\frac{x}{2}+l\right)}{y-\mu x}\right)$

Good, but provably $\mu x > y$, so I would swap the signs in numerator and denominator.
For the range of $f_1$, $\mu$ provides a basis for an upper limit, but what is the lower limit?

 

[Karol]

$$f_1=F\left(\frac{y-\mu\left(\frac{x}{2}+l\right)}{y-\mu x}\right)$$
The nominator and the denominator must have equal signs in order to maintain R's inward direction. f₁ must also be bigger than 0 so it will have a value and that's it's minimum. for F inside:
$$\mbox{and}\left\{\begin{array}{ll}y-\mu\left(\frac{x}{2}+l\right)>0\\y-\mu x>0\end{array}\right.\rightarrow \mbox{and}\left\{\begin{array}{ll}y>\mu\left(\frac{x}{2}+l\right)\\y>\mu x\end{array}\right. \rightarrow y>\mu x$$
That isn't logical since it makes the part high (big y) which is good for sliding, not locking, and the equations here are for locking.
The nominator and denominator can be both negative, and i get a different result:
$$\mbox{and}\left\{\begin{array}{ll}y-\mu\left(\frac{x}{2}+l\right)<0\\y-\mu x<0\end{array}\right.\rightarrow \mbox{and}\left\{\begin{array}{ll}y<\mu\left(\frac{x}{2}+l\right)\\y<\mu x\end{array}\right. \rightarrow y<\mu\left(\frac{x}{2}+l\right)$$
That is logical since it implies a wide part.

 

[haruspex]

Look at your second equation in post #21. F inside means x/2 > l, so $\mu x > y$. Why do you keep asserting the opposite?

 

[Karol]

Look at your second equation in post #21. F inside means x/2 > l, so $\mu x > y$. Why do you keep asserting the opposite?

But if F is outside and x/2 < l then it's inverse: $\mu x < y$

 

[haruspex]

But if F is outside and x/2 < l then it's inverse: $\mu x < y$

No. If x/2 < l then F₂ acts downwards, so is negative.

Edit: I withdraw that. Need to think some more...

Yes, you're right, if F acts outside the wall then the the critical value of $\mu$ is less than y/x. But it would be wrong to interpret that as meaning y needs to be large for it to stay in place. All it says is that $\mu$ does not need to be as much as y/x.
In fact, the "F outside" case is easier. In that set-up, it cannot slip unless it slips at both contacts, so you can take $F_1=F_2=\frac 12 F=\mu R$. You should get a very simple expression for $\mu$.

 

[Karol]

For F outside moments around A:
$$\mbox{and}\left\{\begin{array}{ll}F\left(\frac{x}{2}+l\right)=Ry+\mu Rx\\2\mu R<F\end{array}\right.\rightarrow F\left(\frac{x}{2}+l\right)<\frac{F}{2\mu}(y-\mu x)\rightarrow y>2\mu l$$
And for F inside its $\mu x < y$

 

[haruspex]

For F outside moments around A:
$$\mbox{and}\left\{\begin{array}{ll}F\left(\frac{x}{2}+l\right)=Ry+\mu Rx\\2\mu R<F\end{array}\right.\rightarrow F\left(\frac{x}{2}+l\right)<\frac{F}{2\mu}(y-\mu x)\rightarrow y>2\mu l$$
And for F inside its $\mu x < y$

I agree with your algebra for the F outside case, but the condition seems to be inverted. I thought we wanted the non-slip condition, so it would be $2\mu R > F$, leading to $\mu > y/(2l)$.
For F inside, perhaps you're doing the same, since you keep asserting $\mu x < y$ when I have shown the opposite must be true. Anyway, the inside case is more complicated because we cannot assume the top right corner is on the point of slipping. The frictional force there is anything from zero to $\mu R$. It will take a bit of thought to decide which of those extremes is the right one for the purpose of answering the question.