- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Barrett O'Neil's book: Elementary Differential Geometry ...
I need help with fully understanding the example on wedge products of differential forms in O'Neill's text on page 31 in Section 1.6 ..The example reads as follows:
 I do not follow the computations in this example ... can someone please explain (in very simple terms) how the example "works" ... essentially how do we justify each step in the reasoning ...Particularly puzzling for me is the following ... ...... in the first equation (equation *) we find
I do not follow the computations in this example ... can someone please explain (in very simple terms) how the example "works" ... essentially how do we justify each step in the reasoning ...Particularly puzzling for me is the following ... ...... in the first equation (equation *) we find
[itex]d(fg \ dx \ dy ) = \partial (fg) / \partial z \ dz \ dx \ dy [/itex]
How is this step justified?... then I find all the steps in the second and third equations puzzling as well ...
Can someone explain and justify the steps in the example in terms of O'Neill's Definitions, Theorems and Lemma's ... ...To give readers of this post the context, notation and necessary Definitions, Theorems and Lemma's, I am providing the text of Section 1.6: Differential Forms ... ... as follows ... ...
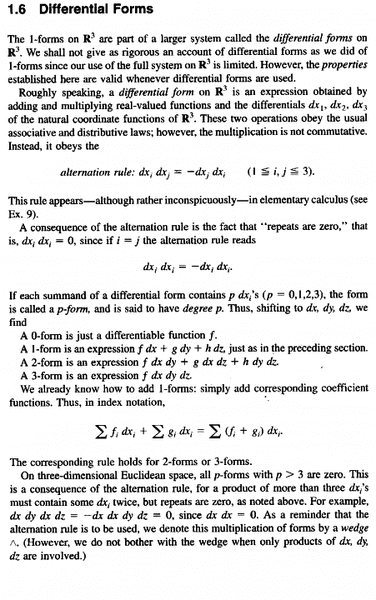

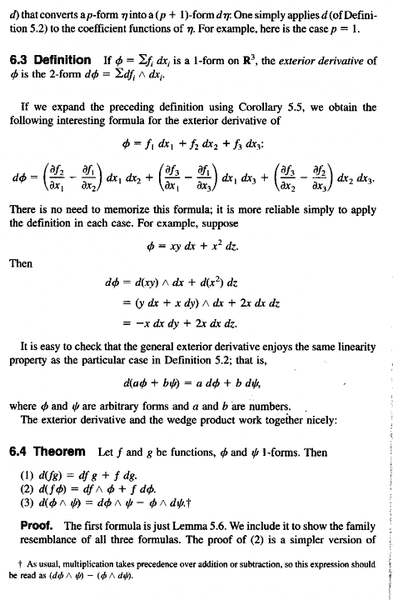
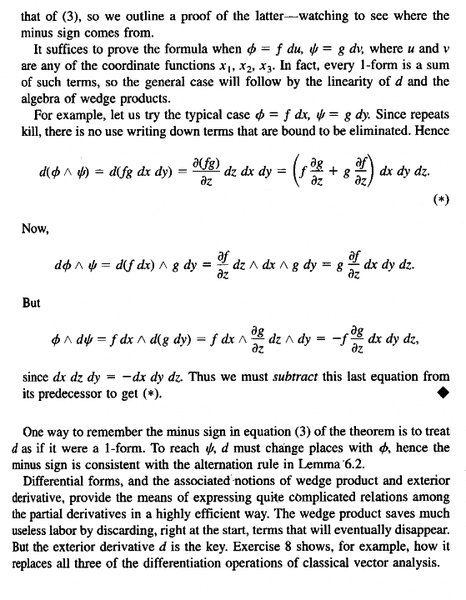
I need help with fully understanding the example on wedge products of differential forms in O'Neill's text on page 31 in Section 1.6 ..The example reads as follows:
[itex]d(fg \ dx \ dy ) = \partial (fg) / \partial z \ dz \ dx \ dy [/itex]
How is this step justified?... then I find all the steps in the second and third equations puzzling as well ...
Can someone explain and justify the steps in the example in terms of O'Neill's Definitions, Theorems and Lemma's ... ...To give readers of this post the context, notation and necessary Definitions, Theorems and Lemma's, I am providing the text of Section 1.6: Differential Forms ... ... as follows ... ...
Attachments
-
 O'Neill - EXAMPLE on page 31 - Wedge Products of Differential Forms.png28.7 KB · Views: 966
O'Neill - EXAMPLE on page 31 - Wedge Products of Differential Forms.png28.7 KB · Views: 966 -
 O'Neill - 1 - Section 1.6 - page 28 - Differential Forms - PART 1 .png41.9 KB · Views: 897
O'Neill - 1 - Section 1.6 - page 28 - Differential Forms - PART 1 .png41.9 KB · Views: 897 -
 O'Neill - 2 - Section 1.6 - page 29 - Differential Forms - PART 2 .png49.1 KB · Views: 872
O'Neill - 2 - Section 1.6 - page 29 - Differential Forms - PART 2 .png49.1 KB · Views: 872 -
 O'Neill - 3 - Section 1.6 - page 30 - Differential Forms - PART 3 .png33 KB · Views: 786
O'Neill - 3 - Section 1.6 - page 30 - Differential Forms - PART 3 .png33 KB · Views: 786 -
 O'Neill - 4 - Section 1.6 - page 31 - Differential Forms - PART 4 .png42.7 KB · Views: 918
O'Neill - 4 - Section 1.6 - page 31 - Differential Forms - PART 4 .png42.7 KB · Views: 918