- #1
Kolika28
- 146
- 28
- Homework Statement
- I'm a bit confussed when it comes to kinetic rotational energy.
- Relevant Equations
- ##K=\frac{1}{2}I\omega^2##
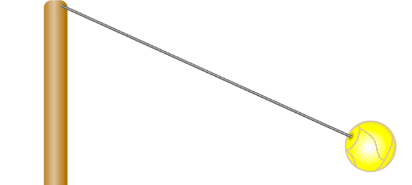
When does an object have rotational energy? Is it only if it rotates around an axis within the object? Does for example a ball attached to a string with a uniform circular movement have rotational kinetic energy?