Gas Expansion in insulated cylinder (piston & Diaphragm)

In summary, the conversation discusses two expansion cases involving an insulated cylinder divided by a separating element - diaphragm for case A and piston for case B. Case A involves a thin diaphragm that is suddenly removed, resulting in a free expansion process. Case B involves a piston that is allowed to move without friction, resulting in an irreversible free expansion process. The final pressure for case A can be determined using the simple equation P1*V1=P2*V2, while the final pressure for case B is more complex and depends on the work done by the gas on the surroundings. It is noted that the final pressure in case B will be lower than in case A due to the work done on the surroundings. The conversation also discusses
  • #1
pyroknife
613
3
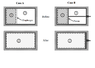
I attached an image of two expansion cases I am analyzing. Both cases involve an insulated cylinder that is divided by a separating element (diaphragm for case A and piston for B). The portion on the right is evacuated. The left contains a calorically perfect gas with an initial pressure and temperature.

Case A is a thin diaphragm and at a certain point in time, it is suddenly removed (removed without friction&flow disturbance) and gas expands to fill the whole cylinder. Case B is a piston which is allowed to move (without friction) while gas expands to fill the whole cylinder.


Would both of these cases be considered free expansion?
 

Attachments

  • Untitled.png
    Untitled.png
    21.7 KB · Views: 533
Physics news on Phys.org
  • #2
Is the piston massive or massless? What difference does that make?
 
  • #3
I believe it is massless. Being massless would imply it is free expansion?
 
  • #4
A massless piston not backed by anything would not impede the expansion in any way.
 
  • #5
voko said:
A massless piston not backed by anything would not impede the expansion in any way.

Got it. I was speaking to a professor about case B. He mentioned that the piston is connected to the outside. Does this change anything in the physics of the problem?
 
  • #6
Most likely it does. What are your thoughts?
 
  • #7
I am thinking that if it's connected to the outside it does work on the surroundings.
 
  • #8
That's what I think, too.
 
  • #9
Hmmm, I am trying to analyze which one of the cases would result in a higher final pressure.

For case A, I believe this is irreversible free expansion process, with no heat transfer and work, thus change in internal energy is zero. Since the system involves a perfect gas where internal energy is only a function of temperature, that would mean change in temperature is zero, making the process isothermal. Thus we can use the simple P1*V1=P2V2, where state 1 is the initial state and state 2 is the final state after the gas expands to cover up the whole cylinder.

For case B, I am a bit lost on how I can go about figuring out the final pressure. Would it be at constant process expansion since work is done on the surroundings?
For case B to expand, wouldn't heat need to be applied to the gas?
 
  • #10
I believe you need to assume that the gas can expand in case B without any external heat. I agree with your method for case A.
 
  • #11
Hmmm, I see. So the gas has to create a expansion force large enough to push the cylinder and expand?
 
  • #12
Yes, it will have to do work on the surroundings.
 
  • #13
I made the assumption that case B was an adiabatic expansion process. So this means the pressure inside has to be larger than outside for it to be expanding. Case B is not free expansion.
If I were to obtain the final pressure for case B, would I have to apply the work equation?
I feel there is a much simpler way.
 
  • #14
pyroknife said:
I made the assumption that case B was an adiabatic expansion process. So this means the pressure inside has to be larger than outside for it to be expanding. Case B is not free expansion.
If I were to obtain the final pressure for case B, would I have to apply the work equation?

You won't be able to unless you know exactly what the "surroundings" are. But that is not required if you just need to state whether the final pressure is lower or greater than in case A.

You know that in case A ##p_1 V_1 = p_2^A V_2 ##. You know that in case B you will have some ##p_2^B##. What do you know about ##p_2^B## without going into details of the process, and knowing solely that some work is done?
 
  • #15
Does your subscript 2 represent the properties associated with the initially evacuated volume or the final state after the gas expansion?
 
  • #16
Since the initial volume has to have a smaller pressure in order for the gas filled volume to expand, that means in the final state, the pressure of the gas should be lowered because work is done on the surroundings.
 
  • #17
Subscript 2 means "final state" (same as in #9 I believe).

Your logic in #16 is not flawless. Take again you reasoning from #9. We know that ##V_2 > V_1##, so ##p_1 V_1 = p_2 V_2 ## implies that ## p_2 < p_ 1 ## even though no work has been done (this ## p_2 ## is what I denoted as ##p_2^A##).
 
  • #18
Is there a formatting issue in your last post? If not, your post appears to be displaying wrong for me.
 
  • #19
I do not see any problem there. What seems wrong?
 
  • #20
voko said:
I do not see any problem there. What seems wrong?

I refreshed it and it looks fine now. It looked weird earlier (see attached).

I can no longer use the relationship P1V1=P2V2 because this is no longer an isothermal process and thus, there is a change in temperature.
 

Attachments

  • Untitled.png
    Untitled.png
    11.4 KB · Views: 418
  • #21
pyroknife said:
I can no longer use the relationship P1V1=P2V2 because this is no longer an isothermal process and thus, there is a change in temperature.

What change? Does it increase or decrease? Why?
 
  • #22
voko said:
What change? Does it increase or decrease? Why?

I think I can answer your question by looking at the ideal gas equation, but I would like to understand it from a more conceptual point of view.

From the ideal gas equation we have P1V1/T1=P2V2/T2. For the gas to expand, P1 has to be greater than the surroundings. So pressure must decrease. Then that means temperature must decrease.
 
  • #23
That could also be said in case A. Yet in case A temperature is constant.
 
  • #24
voko said:
That could also be said in case A. Yet in case A temperature is constant.

Sorry. I was having a dumb moment. I forgot all about volume. Let me think.
 
  • #25
Another question I had (which may help with answering the pressure problem) is if this scenario can be assumed as reversible. I think this cannot be determined because we do not know the rate of expansion? The rate of expansion should be quite low for it to be considered reversible.
 
  • #26
You are overthinking the problem. Answer the questions from #21. Compare with case A. Use the ideal gas law.
 
  • #27
Hmmm, in case A, the pressure and volume change. In case B, the pressure, temperature, and volume change.
We know volume increases for both cases, but I am not sure how to think about the physical process associated with the pressure and temperature change?
For the gas to expand, the pressure must be greater than the surroundings. For some reason, I am thinking that the work done on the surroundings is solely associated with the increase in volume at a constant pressure.
 
  • #28
Once again. You have established that in case A, temperature is constant. Why?

What does that mean with regard to temperature in case B?
 
  • #29
voko said:
Once again. You have established that in case A, temperature is constant. Why?

What does that mean with regard to temperature in case B?


Temperature is constant because there is no change in temperature for the free expansion of a perfect gas.

Case B is not free expansion, therefore the same principle does not hold true. Should I be thinking of this in a different way? I'm not sure how the case A scenario would help in understanding case B's temperature change.
 
  • #30
pyroknife said:
Temperature is constant because there is no change in temperature for the free expansion of a perfect gas.

Why is there no change in temperature in this case?

Case B is not free expansion, therefore the same principle does not hold true.

Consequently, what happens with temperature?
 
  • #31
voko said:
Why is there no change in temperature in this case?



Consequently, what happens with temperature?


I am having problems with looking at this conceptually. I will try to take another crack at justifying case A. Since there's no heat transfer and work in case A, that means there's no change in internal temperature. For a perfect gas, internal temperature is only a function of temperature. Since there's no change in internal energy, there's no change in temperatre. Temperature and internal energy are directly correlated
 
  • #32
pyroknife said:
Since there's no change in internal energy, there's no change in temperatre. Temperature and internal energy are directly correlated

And what does that imply for case B?
 
  • #33
voko said:
And what does that imply for case B?
Oh I see. Internal energy and temp would also be directly correlated for case B because the gas is still an ideal gas. There's no heat but there's a work output thus change in internal energy is negative, which implies change in temperature is negative (a decrease in temp from initial to final)!
 
  • #34
Correct. And what does lower final temperature imply for final pressure?
 
  • #35
voko said:
Correct. And what does lower final temperature imply for final pressure?
Sweet. Hmmm, wouldn't we have to know the change in temperature to determine whether the final pressure increase or decrease?

I forgot to mention the final volume is 6 times the original volume.

Let me work this out mathematically.

The left portion (1) has a volume 5x the volume of the right. I have use "f" index to denote final state rather than (2).
For case A:
P1V1=P_f*V_f, V_f=1/5V1+V1=6/5*V1
=>P_f=5/6*P1 (Decrease in pressure for case A)For case B:
P1V1/T1=P_f*V_f/T_f => P_f=5/6*P1*T_f/T1. The ratio T_f/T1 is less <1, thus P_f for case B is less than P_f for case A.
Is there any way to obtain the T_f/T1 ratio though?
 
Last edited:
<h2>1. What is gas expansion in an insulated cylinder?</h2><p>Gas expansion in an insulated cylinder refers to the process in which the volume of a gas increases due to an increase in temperature or a decrease in pressure. This expansion occurs within a closed system, such as a cylinder with a piston or diaphragm, and is isolated from the surrounding environment by insulation.</p><h2>2. How does gas expansion in an insulated cylinder occur?</h2><p>Gas expansion in an insulated cylinder occurs when the gas particles gain kinetic energy, causing them to move faster and collide with the walls of the cylinder with more force. This results in an increase in pressure and an expansion of the gas volume.</p><h2>3. What is the purpose of using an insulated cylinder for gas expansion?</h2><p>The purpose of using an insulated cylinder for gas expansion is to prevent heat exchange between the gas and its surroundings. This allows for more accurate measurements of the gas expansion and helps maintain a constant temperature and pressure within the system.</p><h2>4. How does the piston or diaphragm affect gas expansion in an insulated cylinder?</h2><p>The piston or diaphragm in an insulated cylinder acts as a barrier that separates the gas from its surroundings and allows for the measurement of the gas expansion. As the gas expands, the piston or diaphragm moves, indicating the change in volume of the gas.</p><h2>5. What factors can affect gas expansion in an insulated cylinder?</h2><p>The main factors that can affect gas expansion in an insulated cylinder are temperature, pressure, and volume. Changes in any of these factors can result in a change in the volume of the gas and the overall behavior of the system.</p>

1. What is gas expansion in an insulated cylinder?

Gas expansion in an insulated cylinder refers to the process in which the volume of a gas increases due to an increase in temperature or a decrease in pressure. This expansion occurs within a closed system, such as a cylinder with a piston or diaphragm, and is isolated from the surrounding environment by insulation.

2. How does gas expansion in an insulated cylinder occur?

Gas expansion in an insulated cylinder occurs when the gas particles gain kinetic energy, causing them to move faster and collide with the walls of the cylinder with more force. This results in an increase in pressure and an expansion of the gas volume.

3. What is the purpose of using an insulated cylinder for gas expansion?

The purpose of using an insulated cylinder for gas expansion is to prevent heat exchange between the gas and its surroundings. This allows for more accurate measurements of the gas expansion and helps maintain a constant temperature and pressure within the system.

4. How does the piston or diaphragm affect gas expansion in an insulated cylinder?

The piston or diaphragm in an insulated cylinder acts as a barrier that separates the gas from its surroundings and allows for the measurement of the gas expansion. As the gas expands, the piston or diaphragm moves, indicating the change in volume of the gas.

5. What factors can affect gas expansion in an insulated cylinder?

The main factors that can affect gas expansion in an insulated cylinder are temperature, pressure, and volume. Changes in any of these factors can result in a change in the volume of the gas and the overall behavior of the system.

Similar threads

I Work in hydrostatic system in cylinder with piston: P_int or P_ext?
    • Classical Physics
Replies
5
Views
576
A Work done during adiabatic expansion on piston
    • Thermodynamics
Replies
8
Views
545
I The relationship between reversible and irreversible processes
    • Classical Physics
Replies
5
Views
1K
B Gas cylinder at constant pressure being exposed to atmosphere, how?
    • Thermodynamics
Replies
8
Views
1K
Can a turbo expander convert more heat to work than a piston expander?
    • Mechanical Engineering
Replies
2
Views
497
Why is temperature constant after gas has expanded?
    • Introductory Physics Homework Help
Replies
33
Views
1K
I Irreversible adiabatic processes & entropy change (clarification needed)
    • Thermodynamics
Replies
22
Views
2K
Adiabatic Expansion of Pressurized Air in a Piston-Cylinder Setup
    • Introductory Physics Homework Help
Replies
8
Views
744
B Adiabatic or Isobaric process?
    • Classical Physics
2
Replies
61
Views
5K
Total force from air spring model (double acting piston)
    • Mechanical Engineering
Replies
8
Views
1K

Hot Threads

Recent Insights

Back
Top