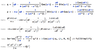- #1
itssilva
- 55
- 0
Some time ago I was playing with the oscillator when I noticed a few funny things. Consider first the 1D oscillator with Hamiltonian $$ \displaystyle H(q,p) = \frac{p^2}{2m} + \frac{m\omega^2}{2}q^2$$ whose solutions are $$ q(t) = q_0cos(\omega t) + \frac{p_0}{m\omega}sin(\omega t), p(t) = m \dot q(t); $$ Also, since the Hamiltonian is time-independent, the energy is a constant of motion given by $$ \displaystyle E(q_0,p_0) = \frac{p_0^2}{2m} + \frac{m\omega^2}{2}q_0^2$$ Now, perhaps unsurprisingly, I notice that taking ## \omega \rightarrow 0 ## as ## O(\omega^2) \sim 0 ## will reduce this problem to that of the free 1D particle. Okay. But now consider the driven oscillator with Hamiltonian $$ \displaystyle H(q,p,t) = \frac{p^2}{2m} + \frac{m\omega^2}{2}q^2 + \lambda cos(\omega_d t)q $$ with solutions $$ q(t) = q_0cos(\omega t) + \frac{p_0}{m\omega}sin(\omega t) + \frac{\lambda}{m(\omega_d^2 - \omega^2)}cos(\omega_d t), p(t) = m \dot q(t); $$ Now the energy is given by $$ \displaystyle E(q_0,p_0,t) = \frac{p_0^2}{2m} + \frac{m\omega^2}{2}q_0^2 + \omega q_0p_0sin(2\omega t) + O(\lambda) $$ (done by hand+Wolfram Alpha - but you may want to double-check it); so, it appears that the previous "trick" doesn't quite work here as well (note that ## O(\lambda) ## is linear in ## q_0,p_0) ##. So 1) I wonder if this is a true "anomaly", and 2) whether there are other known examples (classical or quantum, as may be appropriate here; I was studying the quantum problem when I noticed this detail).
(P.S.: Finally I get myself to post here in LaTex; I'm beaming )
)
(P.S.: Finally I get myself to post here in LaTex; I'm beaming