Calculating the Static Frictional Force of Nylon Bushings

[Apple&Orange]

Hi guys

I'm looking to calculate the static frictional force for a hinge
Below is a CAD image of the component I am testing.

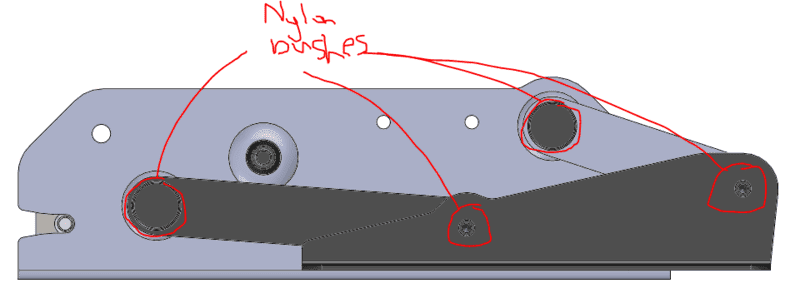
I am thinking of attaching a hook onto the plate as shown below, and continuously add weights at the end of the hook until the hinge begins to move.

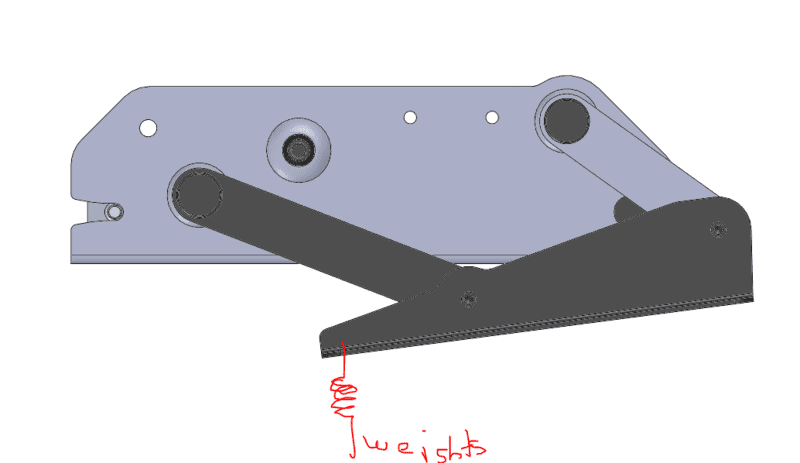
By multiplying the weight with the acceleration of gravity, will this give me the Static Frictional Force?

 

[Dr.D]

The product of weight with the acceleration of gravity is a meaningless concept. I think you are intending the product of mass with the acceleration of gravity, and that would give you the amount of force required to move the linkage.

Now, is that what you want? I don't know; only you can answer that question.

There are four pin joints in this linkage, and there is some amount of friction in each of them. You may well encounter difficulty in running your experiment because friction experiments are often difficult to repeat. Each time you cycle the mechanism, you wear away a bit of material and thus change the system. If you start with a new mechanism for each trial, then you will see the effect of manufacturing variations. You need to do quite a few tests before you can truly characterize the friction in this system.

 

[Apple&Orange]

Thanks, that was what I was looking for. I have a few samples of these hinges to do the test, so I hope this will help with the accuracy.

Just for final confirmation, would I still use the formula F_s = μ_sN, in this situation?
From what I found out, the above formula is used in a lot of linear applications, but not so much in circular.

If it is, I assume the normal force is the clamping force on the nylon washers in between the steel arms?

 

[Dr.D]

It does not appear to me that your test will give the friction force at any particular location, so the expression Fs = mu*N makes little sense. What your experiment shows is the effect of friction on an entire system (the linkage), not the effect of friction at any particular location.

As I said before, we don't really know what it is you want. If you want to understand the friction at each joint, then you will need a different approach. If you only want the effect of friction on the overall system, then your experiment shows that.

 

[CWatters]

What do you plan to do with the answer to your question?

I'm looking to calculate the static frictional force for a hinge

Presumably you mean frictional torque rather than force?

Might be best to test each pivot/hinge on it's own?

 

[Apple&Orange]

Thanks for your responses guys, and apologies; I should've been a bit more clearly on what I'm trying to achieve.

This test was to investigate how much variation there is in terms of the force that is needed to overcome the total friction in the hinges that we stock. The method of investigating this is what was stated in the first post, and I was wanting confirmation if this was correct. Thankfully, you've confirmed this for me Dr.D!

However, as part of another investigation, I would also like to know the frictional force needed to overcome each individual pin joint, because I am pretty sure that they are all different. I am thinking of carrying this out in a method similar to the above, but for curiosity's sake, I would also like to know the friction coefficient in each pin joint.
I was thinking of using the formula F_s = μ_sN to calculate it, but I am uncertain of what the Normal Force, N, would be. I was thinking it will be the force that is holding the nylon washer and pin together?

 

[CWatters]

I was thinking of using the formula Fs = μsN to calculate it

Here is the/an equation for friction moment/torque in a bearing...
http://www.skf.com/us/products/bear...ction/estimating-frictional-moment/index.html

M = 0,5 μ P d

where
μ = constant coefficient of friction for the bearing
P = equivalent dynamic bearing load [N]
d = bearing bore diameter [mm]

There is also a table of typical u values for different types of bearing.

Google suggests Petroff's equation for plain bearings.

https://en.wikipedia.org/wiki/Sommerfeld_number#Petroff.27s_Law