Diffraction on periodic Structures

[Gamdschiee]

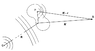
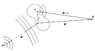
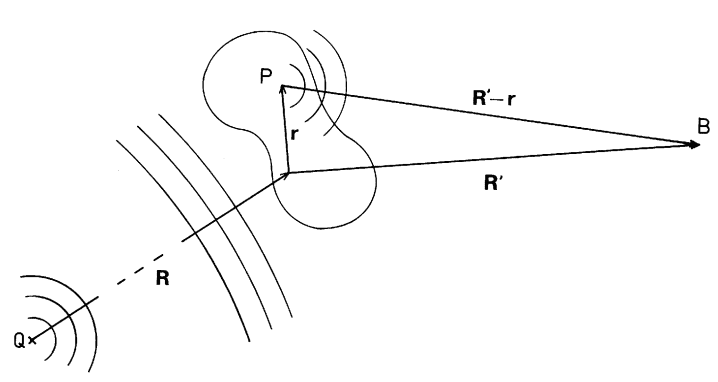
I am trying to understand diffraction on periodic structured in solid state physics.Q is the source of the spherical wave. R the vector to the object and R+r the vector to the scattering centre P, which gives us a another spherical wave.

All spherical waves are considered as plane waves due to the big distance between P,B and Q.

The magnitude at P can be described as: $\Psi_P(t) = \Psi_0e^{i(\vec k (\vec R + \vec r)-\omega_0 t)}$

My questions:

1. The magnitude on point Q, which means $\vec R + \vec r = 0$, is only depending on the time $t$ in $e^{-i\omega_0 t}$. My book(Ibach, Lueth Festkörperphysik) says that it has a fixed phase anytime. According to wikipedia(https://en.wikipedia.org/wiki/Plane_wave#Complex_exponential_form) the phase $\varphi$ is included in $\Psi_0$. **What does it mean to have a "fixed phase" especially at location Q?**

2. "You can only use this plane wave approach $\Psi_B$ for *a* emission process." my book says, but why is it so?

3. "In real emitters do atoms send many photons with uncorrelated phases. (Exception: lasers)" my book also says. Does that mean the phase of the different photos are independent to each other?

 

[Charles Link]

For a simplified case, the source Q can be from a pinhole, and it can be helpful if the scattering region is extended, e.g. a large crystal, that the source has the necessary coherence length, across the extent of the crystal, that the incident wave can be considered to have a constant/fixed phase. Having a monochromatic source, such as laser can be helpful in ensuring that this is the case. $\\$ The Bragg scattering is one of the simpler examples of scattering of crystals that makes use of diffraction theory. To get a Bragg maximum, two conditions are met: 1) The angle of incidence =angle of reflection off of a single plane 2) The path distance between the plane waves reflected off of parallel planes is an integer number of wavelengths. The first condition is the case of a zeroth order diffraction maximum for the individual scatterers from a single plane. The second condition results in interference maxima of integer order $m$. $\\$ There are treatments of Bragg scattering by a crystal that involve writing out the wavefunction and solving for the scattered intensity, sometimes by even writing out the Schrodinger equation. These can get quite complex, and the simplified analysis gets the main features of the scattering, without much mathematical complexity.

 

[Gamdschiee]

Thank you for the answer!

For a simplified case, the source Q can be from a pinhole, and it can be helpful if the scattering region is extended, e.g. a large crystal, that the source has the necessary coherence length, across the extent of the crystal, that the incident wave can be considered to have a constant/fixed phase. Having a monochromatic source, such as laser can be helpful in ensuring that this is the case.

Coherent light are basically electric magnetic waves who have a constant phase, e.g. lasers. So the quote above basically means, when my source Q is a coherence source, the incident light on the crystal has constant phase.

But when the incident wave is a plane wave then there must be a path difference when you compare the "waves" on different point P which are in one line. Is that right? (see figure in starting post.)

 

[Charles Link]

Thank you for the answer!
Coherent light are basically electric magnetic waves who have a constant phase, e.g. lasers. So the quote above basically means, when my source Q is a coherence source, the incident light on the crystal has constant phase.

But when the incident wave is a plane wave then there must be a path difference when you compare the "waves" on different point P which are in one line. Is that right? (see figure in starting post.)

The plane wave can be created by putting the pinhole in the focal plane of a collimating (focussing) lens or mirror. (Parallel rays are brought to a focus. In reverse, light originating from the focused point comes out as parallel rays). $\\$ (This is the general geometry of a diffraction grating spectrometer, where the entrance slit is placed in the focal plane of a collimating mirror to have a plane wave incident on the diffraction grating that may be 2" across or larger. To get the far field pattern from the grating, a second focussing mirror is then used to focus parallel rays emerging from the grating in the plane of the exit slit. The far field diffraction pattern then appears in focus in the focal plane of the second spherical or parabolic mirror which is also the plane of the exit slit. The light emerging from the grating from a monochromatic source is found to be located at angle $\theta$ where $m \lambda=d \sin(\theta)$ (locations of primary maxima). The result is the spectral line (in the shape of the entrance slit) from the monochromatic source is then at position $x=f \, \theta$ in the focal plane of the second focussing mirror. (And yes, the spectral line does appear at multiple locations because of the different orders $m$ of the diffraction maxima)).$\\$ Even with an imperfect lens and/or mirror, (e.g. a spherical mirror instead of a perfectly parabolic one), I believe the degree of spatial coherence across the resulting plane wave is quite good. Perhaps someone else can quantify this, but I think the statement that "the degree of spatial coherence across the resulting plane wave is quite good" is accurate.

 

[Charles Link]

@Gamdschiee Please see my complete post above. I edited it considerably after you last looked at it.

 

[Gamdschiee]

Ah, thanks you for this practical point of view.

So the properties from an incident monochromatic light, which is presented as plane wave, are time coherence, which means constant phase, and spatial coherence, which means no partial difference between my Points P all over the crystal.

But according to simple bragg diffraction there has to be a partial difference between each point P of the reflected plane wave. I.e. either constructive interference or destructive interference. Can you say that?

edit: elastic scattering or inelastic scattering means smth different. I thought that it would be the same as partial difference, but the wave vector k itself can change or not change after the scattering. I.e. energy conservation or no energy conversation.

 

[Charles Link]

Ah, thanks you for this practical point of view.

So the properties from an incident monochromatic light, which is presented as plane wave, are time coherence, which means constant phase, and spatial coherence, which means no partial difference between my Points P all over the crystal.

But according to simple bragg diffraction there has to be a partial difference between each point P of the reflected plane wave. I.e. either constructive interference or destructive interference. This basically means elastic scattering or inelastic scattering. Can you say that?

Yes, that is correct. The plane waves are specularly ( i.e. angle of incidence=angle of reflection) reflected off of many layers of parallel crystal planes each spaced a distance $d$ apart. The condition for constructive interference between reflected waves from two adjacent layers is that $m \lambda=2 d \cos(\theta)$ where $\theta$ is measured from the normal to the crystal planes. Any layer that is equally spaced will also constructively interfere. For the simplest case, the atoms/scattering sites are considered fixed, but if there is thermal motion, there can be an additional Debeye-Waller factor that affects the scattering intensity and results in line width broadening. $\\$ If you are a somewhat advanced student, you may be interested in the book "Thermal Neutron Scattering" by G.L. Squires. It covers the scattering of the neutron wave off of crystals in detail, and even discusses a dynamical theory of scattering in detail in chapter 6, where the derivation goes beyond the simplifying assumption that the incident wavefront is left unchanged by the scatterers. It also discusses the difference between elastic and inelastic scattering (which you asked about) in detail in the early chapters. Even though the immediate application of this book is for neutron scattering, it really applies to scattering of electromagnetic waves as well. The book is not a lengthy one=it is short and to the point, and I highly recommend you take a look at if if you are interested in learning the scattering phenomena in detail. Bragg scattering is also treated in detail in the early chapters. Again, the same equations will apply to an electromagnetic wave that apply to a thermal neutron wave.

 

[Charles Link]

@Gamdschiee I gave this one just a little further thought=in chapter 6 of the G.L. Squires text, he does the case of a dynamical scattering, and he uses the Schrodinger equation for the wave equation that the thermal neutron will obey. Here, there will be a slight difference for the thermal neutron case compared to the E&M case, where Maxwell's equations would apply. For the non-dynamical derivation, in both cases, the scattered wave still has the form $\vec{A}(k) \frac{e^{ik \cdot r}}{r}$. For the dynamical treatment, a periodic potential is used with the Schrodinder equation for the thermal neutron case, and the emerging wavefront is simply the solution of the complete Schrodinger equation. Individual scattering amplitudes are not summed in this dynamical derivation. According to Squires, Darwin and Ewald originally did a dynamical treatment of scattering for the case of x-rays (which would employ Maxwell's equations). $\\$ The overall treatment of the scattering theory in this text, including the Bragg scattering, is quite complete, and I think you would find it of much interest.

 

[Gamdschiee]

Thank you, I will check out that book. Meanwhile I found a book called "solid state physics" by Kittle.

I saw a good picture in it, which also kinda confused me. I want to discuss it please:

E.g. I've never understood why it is $e^{i\vec r \cdot \vec k}$. But why is $\vec r$ exactly the gap between the two atoms, where the incident wave will scatter?

I thought when you write a wave $\vec r$ can be arbitrary. Because $\phi = vec r \cdot \vec k - \omega t$ is the phase of the wave at time t and location $\vec r$. So does that mean we fixed the location at a volume element dV here?

 

[Charles Link]

Usually, at least when you first start out in doing an analysis with waves, the origin is taken to be the place where the wave originates from. Then the phase for a wave with wave vector $\vec{k}$ is $\phi=\vec{k} \cdot \vec{r}$. It turns out that the origin can be put at some other arbitrary location, and it does not affect the phase difference that is calculated between the two sets of rays that get scattered and change direction from $\vec{k}$ to $\vec{k}'$. One gets scatterered at $\vec{X}$ and the other at $\vec{Y}$ in the crystal. If $\vec{r}=\vec{Y}-\vec{X}$, the phase difference in the two sets of rays when viewed in the far field in the direction of $\vec{k}'$ will be $\phi=(\vec{k}-\vec{k}') \cdot \vec{r}$. To compute this in full detail, the two incident paths pick up phase differences $\phi=\vec{k} \cdot \vec{Y} -\vec{k} \cdot \vec{X}=\vec{k} \cdot \vec{r}$, with a similar expression with $\vec{k}'$ for the emerging beam. The correct sign is a minus between $\vec{k}$ and $\vec{k}'$ for the complete phase difference. The reason for the minus sign is not so obvious, but if $\vec{k}=\vec{k}'$, the path and phase difference must necessarily be zero. In the diagram, the incident $\vec{k}$ points along $\vec{r}$ , and the way Kittel shows it in the diagram, $\vec{k}'$ points opposite $\vec{r}$. A similar type of sign problem always becomes an extra detail when treating a diffraction grating problem, with the phase of the transmitted beam, (for a transmission type grating), getting a minus sign for the other quadrant, and similarly for a reflection type diffraction grating for the reflected beam. $\\$ Note that for non-normal angles of incidence ($\theta_i \neq 0$), the complete equation for the position $\theta_r$ of the diffraction primary maxima location is $m \lambda=d(\sin(\theta_i) \pm \sin(\theta_r))$, where the sign of the $\pm$ is determined by what direction is defined to be a positive angle for $\theta_r$.

 

[Gamdschiee]

Usually, at least when you first start out in doing an analysis with waves, the origin is taken to be the place where the wave originates from. Then the phase for a wave with wave vector →k \vec{k} is ϕ=→k⋅→r \phi=\vec{k} \cdot \vec{r} .

Thank you for the quick answer, I am trying to repeat some said things and ask question after it.

So Q can't be the origin where wave really originates from in my posted picture altough it says it in the description? It seems so that the incident beams comes from left bottom. Let's set our Origin T where the plane wave is coming from on bottom left.

From T we can find our scattering location $\vec X$ and $\vec Y$, where the plane wave scatters but with a path difference from $r\sin \phi$ from each other. So we can describe the waves with $e^{i\vec X \cdot \vec k}$ and $e^{i\vec Y \cdot \vec k}$ on the given location. We know that the argument from any sin, cos function is called the phase, which also takes effect for the e-function. As you said above the phase difference from the given waves is $\theta = \vec k \vec r$.

Now I can write $e^{i\theta} = e^{i\vec r \vec k}$ which represents a new wave with given phase. So does that automatically make O as origin? I am not sure how I can imagine this new wave or where it goes.

 

[Charles Link]

Thank you for the quick answer, I am trying to repeat some said things and ask question after it.

So Q can't be the origin where wave really originates from in my posted picture altough it says it in the description? It seems so that the incident beams comes from left bottom. Let's set our Origin T where the plane wave is coming from on bottom left.

From T we can find our scattering location $\vec X$ and $\vec Y$, where the plane wave scatters but with a path difference from $r\sin \phi$ from each other. So we can describe the waves with $e^{i\vec X \cdot \vec k}$ and $e^{i\vec Y \cdot \vec k}$ on the given location. We know that the argument from any sin, cos function is called the phase, which also takes effect for the e-function. As you said above the phase difference from the given waves is $\theta= \vec{k} \vec{r}$.

Now I can write $e^{i\theta} = e^{i\vec r \vec k}$ which represents a new wave with given phase. So does that automatically make O as origin? I am not sure how I can imagine this new wave or where it goes.

The problem is most easily worked backwards for the emerging part. If you go in the far field at the angle where $\vec{k}'$ aims at, let this be the origin of the second part of the problem, and you basically are repeating what you did in the first part of the problem. The result is you get $\phi=(\vec{k} \pm \vec{k}') \cdot \vec{r}$, where I leave it to you to determine the sign of $\pm$. Please see also the last sentence I just added to post 10. $\\$ Edit: One problem emerges in this analysis, and that is that we are using two different origins in working the problem. It is important to see that the origin can be moved to another common location (e.g. somewhere outside of the crystal), and each of the results that we got for the two parts still remain valid. $\\$ And note: These scatterers scatter the light in all directions. We are selecting a specific direction $\vec{k}'$ and seeing how much light gets scattered in that direction. There will be some directions $\vec{k}'$ for which we find that we get constructive interference from the sum of all the scattering amplitudes of the atoms of the crystal, e.g. where the Bragg peaks occur.

 

[Gamdschiee]

Okay thank you, let's set the Origin S on the bottom right. I will have the same result as before only with a wave vector $\vec k'$ we get the wave function $e^{-i\vec r \vec k '}$. The exponent is negative, because my wave vector points to the origin, but I calculated the whole "thing" against it to my Y,X positions.

Both $A=e^{i\vec r \vec k }$ and $B=e^{i\vec r \vec k '}$ are seeming to originate from O, but in opposite directions. So to know the total wave coming from O, I can simply calculate the phase difference of them, which is as you mentioned above. I can describe that new wave originating from P as $C=e^{(\vec k - \vec k ' )\cdot \vec r}$.

Did I derive it correctly?

But what I do not understand how can I imagine this new wave C now? A and B is easy to understand, because one is the incident wave and the other one the scattered wave. C seems to be the difference, but I am not sure how I can imagine that so for making any sense.

 

[Charles Link]

Let's work it a little differently. (You also have an extra prime on the wavevector of A). $\\$ Let's start where the A wave orignates and use that as the origin. The initial wavefunction is $\Phi(\vec{x},t)=A e^{i \vec{k} \cdot \vec{x}-\omega t}$. If we have a scatterer at $\vec{x}'$, it will create an amplitude $aAe^{i \vec{k} \cdot \vec{x}'-\omega t}$ that originates from $\vec{x}'$ and falls off radially (a part that Kittel doesn't show here) as $\frac{1}{r}$ where $r=|\vec{x}-\vec{x}'|$. It's direction is $\vec{k}'$, so that it can be written as $\Phi_{x'}(\vec{x},t)= \frac{aA}{r} e^{-i \omega t} e^{i \vec{k} \cdot \vec{x'}}e^{i \vec{k}' \cdot (\vec{x}-\vec{x}')}=\frac{aA}{r}e^{-i \omega t} e^{i (\vec{k}-\vec{k'}) \cdot \vec{x}'} e^{i \vec{k}' \cdot \vec{x} }$. You then sum over all the scatterers at the various $\vec{x}'$ (where Kittel uses the coordinate $\vec{r}$ in place of $\vec{x}'$ ). The final observation point $\vec{x}$ is at a location in the far-field in the direction of $\vec{k}'$. $\\$ Kittel's derivation is actually somewhat clumsy. You might find what I have written here as an improved approach. This is how Squires and most Optics books treat the problem. $\\$ In the far field the denominator $r=|\vec{x}-\vec{x}'|$ can be treated as a constant.

 

[Gamdschiee]

Thank you. I guess $a$ is a typo, right?

I understand that when a wave is coming form our Origin it will have some certain amplitude at your scatterer $\vec x '$. I know acoustic waves radially decrease their amplitude from their origin, but why is this in our case only at the scatterer and not at the origin the initial wavefunction is coming from?

What I do not understand is why you can simply multiply the radially decreasing amplitude at our scatterer with $e^{i \vec k' \cdot (\vec x - \vec x')}$ to get $\Phi_x'$?

 

[Charles Link]

$a$ is not a typo. It is the scattering amplitude, and it can be a complex number if the scattering produces a phase delay, etc. (It can also be a function of direction, i.e. $a=a(\vec{k}')$ ). $\\$ These equations are much easier to understand when written in one dimension rather than as $\vec{k} \cdot \vec{x}$. The observation point $\vec{x}$ in the far-field is always along $\vec{k}'$. Let's write a wave in one dimension, traveling to the right with velocity $v$, that begins at coordinate $x=b$ and has wavevector $k$. Let's also assume that it has time dependence $g=g(t)=A \cos(\omega t)$ at $x=b$. The wave function $f(x,t)=g(t-\frac{x-b}{v})=A \cos(\omega t-\frac{\omega}{v}(x-b))=A \cos{(\omega t-k(x-b))}=A \cos{(k(x-b)-\omega t)}$. $\\$ By comparing this with the 3-D case, it should be apparent that the wave that originates at $\vec{x}'$, (rather than at $x= b$), that has wavevector $\vec{k}'$, (and remember $\vec{x}$ is in the direction of $\vec{k}'$ ), can be written as $\Phi_{x'}(\vec{x},t)=Ae^{i \vec{k}' \cdot (\vec{x}-\vec{x}')-\omega t }$, where, if you like, we can take just the real part of this wave function. $\\$ The only thing I have left off here is the initial amplitude factor of the scatterer at $\vec{x}'$. It starts with an amplitude factor (reflection/scattering) coefficient $a$ along with a phase factor $e^{i \vec{k} \cdot \vec{x}' }$. Including those factors, along with the $\frac{1}{r}$ factor, (where $r=|\vec{x}-\vec{x}'|$), gives the result $\Phi_{x'}(\vec{x},t)=\frac{aA}{r} e^{i (\vec{k}-\vec{k}') \cdot \vec{x}'}e^{i \vec{k}' \cdot \vec{x}-\omega t }$. $\\$ One additional item: Let's take a closer look at the term $\vec{k}' \cdot ( \vec{x}-\vec{x}')$ in the exponential of the first expression for $\Phi_{x'}(\vec{x},t)$ above, where we converted to the 3-D case. If the location $\vec{x}'$ of the scatterer occurs at a location in the crystal that is a distance that is perpendicular to $\vec{k}'$, this distance/displacement does not cause any extra path distance from the crystal to the observation point $\vec{x}$. That's as it should be, and the dot product $\vec{k}' \cdot \vec{x}'$ ensures this. This term is affected by displacements in $\vec{x}'$ that occur along the direction of the emerging wave that is being observed. Thereby, the replacement of $k(x-b)$ with $\vec{k}' \cdot (\vec{x}-\vec{x}')$ is indeed correct.

 

[Gamdschiee]

Thanks for the accurate answer.

These equations are much easier to understand when written in one dimension rather than as $\vec{k} \cdot \vec{x}$ . The observation point →x \vec{x} in the far-field is always along $\vec{k}'$ . Let's write a wave in one dimension, traveling to the right with velocity $v$ , that begins at coordinate $x=b$ and has wavevector $k$ . Let's also assume that it has time dependence $g=g(t)=A \cos(\omega t)$ at $x=b$ . The wave function $f(x,t)=g(t-\frac{x-b}{v})=A \cos(\omega t-\frac{\omega}{v}(x-b))=A \cos{(\omega t-k(x-b))}=A \cos{(k(x-b)-\omega t)}$ .

So when I have an observation point $\vec x$ in the far-field, I always write it like that, when I want to observe in the far-field and I got a scatterer(or origin that "produces" a wave) at x=b?
Which we can write in 3D as you showed: $D=Ae^{i\vec k (\vec x - \vec x ')-\omega t}$

The only thing I have left off here is the initial amplitude factor of the scatterer at $\vec{x}'$ . It starts with an amplitude factor (reflection/scattering) coefficient a a along with a phase factor $e^{i \vec{k} \cdot \vec{x}' }$ . Including those factors, along with the $\frac{1}{r}$ factor, (where $r=|\vec{x}-\vec{x}'|$), gives the result $\Phi_{x'}(\vec{x},t)=\frac{aA}{r} e^{i (\vec{k}-\vec{k}') \cdot \vec{x}'}e^{i \vec{k}' \cdot \vec{x}-\omega t }$ .

They wave coming from bottom left, which triggers the scattering at $\vec x'$ can be described in general as $\Psi(\vec x, t)=Ae^{i\vec k \vec x - \omega t}$.

So we know our scatterer location and can write his amplitude as $E=\frac{ae^{i\vec k \vec x'}}{|\vec x - \vec x '|}$.
Right?

And now I still do not understand why exactly you can just multiply D and E to get the final amplitude of the scattered wave at the observer $\vec x$ in the far field.

 

[Charles Link]

And now I still do not understand why exactly you can just multiply D and E to get the final amplitude of the scattered wave at the observer →xx→\vec x in the far field.

It's a linear process. These are electric fields that are also propagating sinusoidal waves. You have an incident electric field, (the incident wave function). A fraction of it, given by the scattering amplitude as the linear factor, winds up at the location $\vec{x}$ in the far field. It's similar to the linear response you get in a linear electrical circuit, where for a given frequency ac input $V_{in}(\omega)$, you can write $V_{out}(\omega)=m(\omega) V_{in}(\omega)$, for some complex constant $m(\omega)$ that may be frequency dependent.$\\$ When $m(\omega)$ is a complex constant in the form of $z=z_o e^{i \phi}$, the $\phi$ is the phase (delay) factor for the sinusoidal time dependence $e^{-i \omega t}$. The result then becomes $cos(\phi-\omega t)=\cos(\omega t -\phi)$ when just the real part is computed. $\\$ And the electric fields from each of the scatterers are summed at the location $\vec{x}$. They add linearly by superposition. The incident E-M wave is sinusoidal in time. As it travels over a distance $s$, the result, because it is a sinusoidal wave, is a phase factor $\phi=ks$, along with a $\frac{1}{s}$ factor ($r=|\vec{x}-\vec{x}'|$ in the denominator), which, when the energy (intensity) is computed, (which is the square of the E-field amplitude), results in an inverse square law. The incident plane wave is often a collimated plane wave beam that doesn't contain this inverse square law factor. Note that the scattered waves are assumed to be small enough that they don't change the incident beam that each scatterer sees.$\\$ You could do a similar thing with sound waves. If you know the intensity of the sound wave (it's a sinusoidal disturbance in the air pressure $p=p_o+p_1 \cos(\omega t)$), and the frequency and speed of sound, you could calculate what kind of "echo" an obstacle (scatterer) will produce in the far-field at some location $\vec{x}$. Since the incident beam is a disturbance that is sinusoidal in time, the sound that arrives at location $\vec{x}$ will also be sinusoidal in time. Basically you just need to keep track of any phase differences $\phi=\frac{2 \pi}{\lambda} s=ks$ that occur because of the path length along with any inverse square law effects over distance, and you could write the equation for the intensity of sound at location $\vec{x}$ as a function of time. The intensity (energy) of the sound wave is proportional to $p_1^2$. I don't want to get too off track from the original topic, but I think the analogous acoustic problem can be helpful to see what occurs in the case of E-M scattering. See also: http://physics.bu.edu/~duffy/py105/Sound.html $\\$ Additional note: The $\phi$ or $e^{i \phi }$ is called a phase factor. In the expontial notation, it winds up getting multiplied, but the ultimate effect is that it winds up in the term $\cos(\omega t-\phi)$ not as a "multiplying factor", but rather as a time/phase delay.

 

[Gamdschiee]

Thank you for your help, I thought about it and I understand it a bit better now!

I also found another German book "Festkörperphysik" by Gross and Marx. There I found a picture for which explains the so-called Laue Equations:

You described this earlier as $m\lambda = d(sin(\theta_i)\pm sin(\theta_r))$ in #10. But in my book it says: $m\lambda = |\vec r|(cos \varphi ' - cos \varphi)$

1. Why did you use sin()? Did you maybe use different angles?
2. The right term of the equation is apparently the path difference. But why? I don't see how this could be the path difference between the incident plane wave and the reflected plane wave. Because e.g. $r\cdot cos(\varphi)$ is the path difference between the incident wave at 0 and r. And same goes for the reflected wave. $r\cdot cos \varphi '$ is just the path difference between 0 and location r.
So why would the difference of these path differences the path difference in general? Or why is this constructive interference?

 

[Charles Link]

Thank you for your help, I thought about it and I understand it a bit better now!

I also found another German book "Festkörperphysik" by Gross and Marx. There I found a picture for which explains the so-called Laue Equations:
View attachment 228881

You described this earlier as $m\lambda = d(sin(\theta_i)\pm sin(\theta_r))$ in #10. But in my book it says: $m\lambda = |\vec r|(cos \varphi ' - cos \varphi)$

1. Why did you use sin()? Did you maybe use different angles?
2. The right term of the equation is apparently the path difference. But why? I don't see how this could be the path difference between the incident plane wave and the reflected plane wave. Because e.g. $r\cdot cos(\varphi)$ is the path difference between the incident wave at 0 and r. And same goes for the reflected wave. $r\cdot cos \varphi '$ is just the path difference between 0 and location r.
So why would the difference of these path differences the path difference in general? Or why is this constructive interference?

The equation $m \lambda=d \sin(\theta)$ is used frequently enough, that I should get you a "link" with a picture. Here, I found one: https://en.wikipedia.org/wiki/Fraunhofer_diffraction#/media/File:Double_slit.svg Having done a lot of work with diffraction grating spectrometers, I have seen this equation countless times. I haven't done the solid state case of scattering by a crystal nearly as often. Your post was the most in-depth check of those equations to date that I have done. $\\$ The path distance comes in two parts: First the two incident rays a distance $d$ apart have a path distance difference of $d \sin(\theta_i )$. Then the two diffracted parallel rays have a path difference of $d \sin(\theta_r)$, but this second part can come with a minus sign, depending upon which direction is chosen as a positive $\theta_r$. $\\$ This far-field (Fraunhofer) two-slit and multi-slit case $\Delta=d(\sin(\theta_i) \pm \sin(\theta_r))$ is actually much simpler to compute than the solid state vector calculation. You simply assume the two incident parallel rays originate from the same point, and calculate the difference in distance of the two rays to the two slits that are spaced a distance $d$ apart. The scattered rays are observed at the same location in the far field, and it is really the identical calculation as the incident rays, to compute the path distance difference to a faraway point in the far field. The calculation is done in one plane, and you don't need to work with 3 dimensions. And, yes, the angle in this Fraunhofer rwo-slit diffraction case is measured from the perpendicular bisector of the line connecting the two slits, instead of employing a vector $\vec{d}$ that points from one slit to the next. In the case of a diffraction grating, the angle is measured from the normal to the grating.

 

[Gamdschiee]

Ahh, thank you I understand the Fraunhofer example now!

1. So the so-called Bragg Equation comes from $\theta_i = \theta_r = \theta$, which results in $m\lambda = d(sin \theta + sin \theta)=2dsin\theta$. But I also want to understand the picture I posted. So $cos \varphi$ is negative, because the angle $\varphi$ is negative? But it makes no sense to me.

2. I want to discuss the diffraction on a simple optical grate with x slits please, because it is very similar to the atomic grate in a crystal. The intensity looks like this, when we have a certain number of slits and a ray of light with wavelength according to the Bragg equation falls on the grate:

So the different five highest peaks are called m-th order. And when $\theta_i = \theta_r$ we have the strongest constructive interference, which gives us the highest five peaks we see in this picture. Can you actual say that?

 

[Charles Link]

For the diffraction grating, there are two types: A transmission grating and a reflection grating. The equations are the same for both. Let's just consider the transmission grating. ( For the reflection grating, the narrow slits are replaced by "Huygens mirrors" that scatter the light in all directions, effectively doing the same thing as narrow slit Huygens sources that scatter the light in all directions). For these Huygens sources, single slit diffraction applies, so that the light can be assumed to scatter in a fairly wide pattern. If the slits are finite in size, the pattern will narrow somewhat. The result for finite slit width is an intensity pattern that is a product of the interference factor multiplied by a diffraction pattern factor for a single slit. See http://www.physics.louisville.edu/cldavis/phys299/notes/lo_msgratings.html $\\$ In the case of a reflective type grating, (as well as for a transmission type grating), this will mean the pattern is highest for the central $m=0$ maximum, unless a blazed grating is used that makes a specular reflection and makes the peak intensity occur at some other angle, e.g. at $m=1$ or thereabouts. See https://en.wikipedia.org/wiki/Blazed_grating (The central $m=0$ maximum, in the case of the reflection grating is the specular reflection. For the transmission grating, the central $m=0$ maximum is centered in the straight-line path through the grating). $\\$ To comment on your first question about the Bragg scattering=it is always somewhat clumsy in needing to figure out where the angle is measured from: I have done diffraction gratings most often where they use $m \lambda=d \sin(\theta)$. Every time I pick up a solid state physics book such as Kittel or Ashcroft-Mermin to read about Bragg scattering, I always need to reconstruct their coordinate system to get the $m \lambda=2 d \cos(\theta)$ result.

 

[Gamdschiee]

I see so the x-ray diffraction pattern must be the same for 3D crystals:

Let's consider the peak (110) at the bcc crystal above. (110) also called miller indices and describes a simple plane in the crystal. (110) has many planes parallel to each other. So that parallel planes are like a diffraction grate.

But why does this only represent one peak at 110? Is this the "main peak" or how can you interpret this diffraction pattern? For multiple-slits it's easier somehow.

 

[Charles Link]

In the case of x-ray diffraction Bragg peaks off of a crystal, two things occur: We have $\theta_i=\theta_r$ for a single plane, and $m \lambda=2 d \cos(\theta)$ between parallel planes. In general, this means that there could be peaks for multiple $m$, at different locations. The largest value of $\cos(\theta)$ is $1$, so that if the length of the x-rays is just slightly less than $2d$, there will only be an $m=1$. $\\$ A careful study of the way the light reflects off of parallel planes shows that the scattered light off of an individual plane is taking on the geometry of the transmission type grating rather than a reflection grating. For the transmission grating, the $m=0$ is the case of light going in the forward direction. The peak that occurs in this direction is ignored in these scattering experiments=in general there is always the main beam that is disregarded. Thereby, $m=0$ is ignored, and if $\lambda$ is sufficiently large, the only peak may be $m=1$. For shorter wavelength x-rays, there should also be $m=2$ and $m=3$, etc. $\\$ Alternatively, instead of viewing the parallel planes like the slits of a diffraction grating, it can be viewed as parallel planes of a Fabry-Perot type geometry. (Fabry-Perot effect is the interference effect(s) that occurs between parallel planes). In that case $\Delta=2 d \cos(\theta)$. Once again, the coordinate system always needs a careful review to see where $\theta$ is being measured from. $\\$ Just an additional item on the angle: There are textbooks and "links" that reference the angle of incidence from the planes of the crystal rather than from the normal to these planes. They then write Bragg's law as $m \lambda=2 d \sin(\theta)$. It always requires carefully drawing the diagram to get the angles right.

 

[Gamdschiee]

We have θo=θr \theta_o=\theta_r for a single plane, and mλ=2dcos(θ) m \lambda=2 d \cos(\theta) between parallel planes. In general, this means that there could be peaks for multiple m m , at different locations. The largest value of cos(θ) \cos(\theta) is 1 1 , so that if the length of the x-rays is just slightly less than 2d 2d , there will only be an m=1 m=1 .

But what is when the incident angle is not equal the reflection angle, when we see the crystal as parallel planes? How does that change any diffraction pattern?

Because the light is scatterecd in all directions $\vec k'$ we said, which means the angle of the reflected light isn't always the same.

 

[Charles Link]

But what is when the incident angle is not equal the reflection angle, when we see the crystal as parallel planes? How does that change any diffraction pattern?

Because the light is scatterecd in all directions $\vec k'$ we said, which means the angle isn't always the same.

If $\theta_i \neq \theta_r$, the atoms in a single plane will not make constructive interference upon their respective scattering. The result from a single plane will then be very limited scattering. The single plane scatters the vast majority of its scattered light, (which can even be somewhat minimal), in the direction where $\theta_i=\theta_r$. The rest, which is the vast majority of the initial light, simply goes straight through in the forward direction as the main beam. It is possible the parallel planes constructively interfere by satisfying $m \lambda=\Delta$, but there is not sufficient scattering in an arbitrary direction from each single plane (the calculation would be done by summing scattering amplitudes of single planes, multiplying by the number of planes, and then squaring) to result in much intensity. I believe it requires the specular reflection off of a single plane to get a peak. (It took me a little effort to figure this part out, but I think I now have it correct). This is part of the subject of crystallography. See: https://en.wikipedia.org/wiki/X-ray_crystallography $\\$ In general, you need to rotate the crystal w.r.t. the source to get a Bragg peak, but there are enough different parallel sets of crystal planes in a typical crystal, that there are often quite a number of Bragg peaks. (I edited this part, but I think I now have this part correct). $\\$ Alternatively, Bragg rings can be found in the powder method, where instead of rotating the crystal, essentially every angle of incidence is present in the powder.

 

[Gamdschiee]

Thank you.

If θi≠θr \theta_i \neq \theta_r , the atoms in a single plane will not make constructive interference upon their respective scattering. The result from a single plane will then be very limited scattering.

What do you mean by that? That ONLY in a single plain with $\theta_i \neq \theta_r$ you can't have constructive interference at all? What about parallel planes then?

It is possible the parallel planes constructively interfere by satisfying mλ=Δ m \lambda=\Delta , but there is not sufficient scattering in an arbitrary direction from each single plane to result in much intensity.

What do you mean with $\Delta$ here btw?
And also what do you mean that there is no sufficient scattering in an "arbitrary direction from each single plane"?

The single plane scatters the vast majority of its scattered light, (which can even be somewhat minimal), in the direction where θi=θr \theta_i=\theta_r

Does that mean, that when $\theta_i = \theta_r$ there will be constructive interference on a single plain only?

 

[Charles Link]

@Gamdschiee To answer your question(s): The total electric field amplitude is computed by summing the amplitudes (along with a phase factor) of all of the contributing atoms in the crystal. The summation can be done in many ways, but one of the simpler ways is to first take the sum over each plane and then sum the planes. If each plane does not have $\theta_i=\theta_r$, the phases of the individual atoms in that plane are going to be somewhat random and tend to cancel. Thereby, a single plane will have very low amplitude. Next these planes are added together, where there is constructive inteference and the phases line up, but each amplitude started out so small from an individual plane, that the resultant is considerably less than one that has $\theta_i=\theta_r$ across the plane, making the individual atoms in the plane constructively interfere with a $m=0$ reflective maximum. $\\$ For this latter case all of the atoms from a single plane are in phase upon reflection (with $m=0$), and then all of the parallel planes are also in phase (with some $m'$, where $m' \lambda=2d \cos(\theta)$ ) , making every atom of the crystal in phase for a Bragg peak.

 

[Gamdschiee]

So you basically can say that the cases (1) $\theta_i \neq \theta_r$ and (2) $\theta_i = \theta_r$ both appear upon diffraction, but the first one can be ignored due to low intensity in comparison with the second case?

For this latter case all of the atoms from a single plane are in phase upon reflection (with m=0 m=0 ), and then all of the parallel planes are also in phase (with some m′ m' , where m′λ=2dcos(θ) m' \lambda=2d \cos(\theta) ) , making every atom of the crystal in phase for a Bragg peak.

Why is there a single Bragg Peak for m=0, when you only consider a single plane? What about m=1,2,3...?
When you consider parallel planes there are orders of m=1,2,3... . But why?

 

[Charles Link]

@Gamdschiee Your second question is a good one, and it took me some careful thought to figure out what I think is the answer. A single plane is typically a square array of atoms, and $m=0$ allows a peak to occur at virtually any angle of incidence. There is plenty of freedom in aligning this angle up to met the requirement of $m' \lambda=2d \cos(\theta)$. I don't know that there is the same amount of freedom in where the $m=1$ maximum might appear. There could and/or perhaps should be cases where e.g. the $m=1$ maximum also occurs, but remember, our other equation actually reads $m' \lambda=d(\cos(\theta_i)+cos(\theta_r))$. Also, from a single plane, with $\theta_i \neq \theta_r$, we have a two dimensional array for which we are trying to find other maxima. It may be quite difficult to find a direction where all the atoms in the crystal constructively interfere, for this case where $\theta_i \neq \theta_r$. $\\$ One suggestion would be to construct such a crystal: Find the location of an $m=1$ type maximum, e.g. let the incoming ray be in the x-z plane at angle $\theta_i$, and the $x$ crystal spacing be such that it makes a $m=+1$ maximum for what will essentially be a grating of slits and/or reflective grooves in the plane. Determine $\theta_r$ for this $m=1$ case. Finally, determine what $d_z$ needs to be to also have the condition $m' \lambda=2 d_z (\cos( \theta_i)+\cos(\theta_r))$ satisfied. I don't know that this case has nearly the mathematical freedom of simply keeping $\theta_i=\theta_r$, (for any and all choices of azimuthal angle $\phi$), and varying $\theta_i$, (and/or $\phi$), until you find a peak. For the case of $\theta_i \neq \theta_r$, the choice of $\phi$ might be very important, and if you don't get it right, you might not get the peak. It would appear that there may be some cases where $\theta_i \neq \theta_r$, but they might be very hard to locate, and we can't write a simple formula for them like we can for the Bragg condition. $\\$ Alternatively, for this case with $\theta_i \neq \theta_r$, with arbitrary $d_z$, it appears, if we set $\phi=0$, and simply vary $\theta_i$, we should, in fact, get a peak, e.g. in a cubic lattice, where all the atoms constructively interfere for which the Bragg condition is not satisfied. I would need to consult the textbooks on x-ray crystallography=perhaps this case is indeed included. $\\$ Edit: And I worked out an example of this: At normal incidence, with incident beam in the z-direction, let a single plane be an array with $d_x=\sqrt{2} \lambda$, and $d_y$ anything else. This generates an $m= +1$ maximum, (and $m=-1$ ) maximum at angle $\theta_r =45^o$. Choose $d_z$ so it satisfies $(1)(\lambda)=d_z (\cos(0^o)+\cos(45^o))$, or even use $(2)(\lambda)$ on the left side. Then we have all of the scattered amplitudes from each of the atoms in phase, and there is no Bragg condition that is being satisfied here.

 

[Gamdschiee]

Thank you, I think I get the principal, but what do you mean with the angle $\phi$?

And could you explain again please, when to use a + sign before the cos() and a - sign before it? I can't imagine that part how I should work out this.

 

[Charles Link]

Thank you, I think I get the principal, but what do you mean with the angle $\phi$?

The $\theta$ is a polar type angle. If we are just working basically in two dimensions e.g. with a diffraction grating, the $\phi$ is omitted. Similarly if we reflect from each plane so that $\theta_i=\theta_r$, we can essentially ignore the effect of the geometry of how the atoms are arranged on that plane. (That is only the case for the $m=0$ maximum). Then we can consider the planes as uniform, and only need to concern ourselves with the polar angle $\theta_i$ (spherical coordinates) relative to the z-axis. If we are considering the $m=1$ case that occurs for reflection off of a single plane, it only occurs for $\theta_i$ where $(1)(\lambda)=d(\sin(\theta_i)+\sin(\theta_r))$ (e.g. a rectangular array of atoms) if the azimuthal $\phi$ angle is zero. $\\$If we were to test this with a diffraction grating using a pinhole type (monochromatic=laser) source instead of a slit with a parabolic mirror to collimate the beam, and rotated the grating to some $\theta_i$, so that it has a plane wave incident on it at angle $\theta_i$, we could then test for the effect on the resulting $m=1$ bright spot that is found in a spectrometer where the far-field pattern is observed on the exit slit because a second parabolic mirror is used to focus the far-field pattern in the plane of the exit slit. If the grating is tilted backwards, it will make the focused $m=1$ spot occur slightly elevated on the upper part of the exit slit, but if that angle is tilted too much, I think the focused spot quality would be greatly reduced. It would start to become a big blob rather then a small focused spot. $\\$ Here, again is where I would really need to consult an x-ray crystallography textbook to see if they treat the case of a peak that doesn't satisfy the Bragg condition.

 

[Charles Link]

This last item, which really came about because of your question @Gamdschiee in post 29, could use some further research. I was unable to locate any mention of it in a google search. Normally, researchers in this field of x-ray scattering are quite thorough. I think it is likely that there will indeed be peaks of this nature occurring on a somewhat regular basis, and I expect they do take them into account. This finer feature doesn't appear to be presented in most textbooks discussions on Bragg scattering, but mathematically, the conditions for constructive interference appear to be met by all of the atoms in this case, so I would expect occasionally such peaks would occur and be observed experimentally.