You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Volume integral Definition and 50 Threads
In mathematics (particularly multivariable calculus), a volume integral refers to an integral over a 3-dimensional domain; that is, it is a special case of multiple integrals. Volume integrals are especially important in physics for many applications, for example, to calculate flux densities.
View More On Wikipedia.org
View More On Wikipedia.org
-
P
I Change of Variables in Double Volume Integral
In Greiner's Classical Electromagnetism book (page 126) he has a derivation equivalent to the following. $$\int_V d^3r^{'} \nabla \int_V d^3r^{''}\frac {f(\bf r^{''})}{|\bf r + \bf r^{'}- \bf r^{''}|}$$ $$ \bf z = \bf r^{''} - \bf r^{'} $$ $$\int_V d^3r^{'} \nabla \int_V d^3z \frac {f(\bf z +... -
D
Volume integral of x^2 + (y-2)^2 +z^2 = 4 where x , y , z > 0
(a) i sketched a quarter of a sphere centred at x=0 , y=2 , z=0 (b ) ∫ ∫ √ (4-x2 - (y-2)2) dx dy with limits 0 < x < 2 and 0 < y <4 (c ) i converted to spherical polars and took the integrand as 1/r2 . the volume element is r2sinθ drdθd∅ This leads to the triple integral of sinθ with...- dyn
- Thread
- Replies: 21
- Forum: Calculus and Beyond Homework Help
-

Volume integral over a gradient (quantum mechanics)
Homework Statement 1) Calculate the density of states for a free particle in a three dimensional box of linear size L. 2) Show that ##\int f \nabla g \, d^3 x=-\int g \nabla f \, d^3 x## provided that ##lim_{r \rightarrow \inf} [f(x)g(x)]=0## 3) Calculate the integral ##\int...- astrocytosis
- Thread
- Replies: 2
- Forum: Advanced Physics Homework Help
-
M
I Why is this volume/surface integration unaffected by a singularity?
##\mathbf{M'}## is a vector field in volume ##V'## and ##P## be any point on the surface of ##V'## with position vector ##\mathbf {r}## Now by Gauss divergence theorem: \begin{align} \iiint_{V'} \left[ \nabla' . \left( \dfrac{\mathbf{M'}}{\left| \mathbf{r}-\mathbf{r'} \right|}... -

How to find the limits of a volume integral?
Homework Statement If ##\vec { F } = x \hat { i } + y \hat { j } + z \hat { k }## then find the value of ##\int \int _ { S } \vec { F } \cdot \hat { n } d s## where S is the sphere ##x ^ { 2 } + y ^ { 2 } + z ^ { 2 } = 4##. The Attempt at a Solution From gauss divergence theorem we know ##\int...- Hawkingo
- Thread
- Replies: 6
- Forum: Calculus and Beyond Homework Help
-

Problem solving this volume using Jacobi's Determinant
Homework Statement Find the value of the solid's volume given by the ecuation 3x+4y+2z=10 as ceiling,and the cilindric surfaces 2x^2=y x^2=3*y 4y^2=x y^2=3x and the xy plane as floor.The Attempt at a Solution I know that we have to give the ecuation this form: ∫∫z(x,y)dxdy= Volume So, in fact...- JorgeM
- Thread
- Replies: 1
- Forum: Calculus and Beyond Homework Help
-

Calculate the volume integral of divergence over a sphere
Homework Statement For the vector field F(r) = Ar3e-ar2rˆ+Br-3θ^ calculate the volume integral of the divergence over a sphere of radius R, centered at the origin. Homework Equations Volume of sphere V= ∫∫∫dV = ∫∫∫r2sinθdrdθdφ Force F(r) = Ar3e-ar2rˆ+Br-3θ^ where ^ denote basis (unit vectors)...- Vitani11
- Thread
- Replies: 13
- Forum: Advanced Physics Homework Help
-
A
How do integrals actually work?
Homework Statement Calculate the electrical energy required to assemble a spherical volume of radius R and charge Q, homogeneous density ρ the answer is (3/5)Q/R the textbook says you have to build the volume integral one layer of sphere at a time, I'll get back to that later. I like...- Aran Weizman
- Thread
- Replies: 4
- Forum: Calculus and Beyond Homework Help
-

Volume integral of a function over tetrahedron
Homework Statement Calculate the volume integral of the function $$f(x,y,z)=xyz^2$$ over the tetrahedron with corners at $$(0,0,1) (1,0,0) (0,1,0) (0,0,1)$$ Homework Equations I was able to solve it mathematically, but still can't figure out why the answer is so small. I only understand...- physkim
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
R
How can a volume integral yield a vector field?
I'm using the textbook Electricity and Magnetism by Purcell. In the section about continuous charge distributions I found the following formula \mathbf{E}(x,y,z)= \frac{1}{4\pi\epsilon_0 } \int \frac{\rho(x',y',z')\boldsymbol{\hat r} dx'dy'dz'}{r^{2}} . It's stated that (x,y,z) is fixed...- rcmps772
- Thread
- Replies: 8
- Forum: Other Physics Topics
-
D
How is the inverse of a volume integral denoted?
In 1-D the inverse of ∫ dx is dy/dx so how is the inverse of the volume integral ∫ d3x = ∫ dxdydz denoted ? Thanks -
F
How can divF=0, but the volume integral of divF=/=0
Homework Statement Basically, this is part C of a question where in part A we had to use the RHS of the divergence theorem below to calculate the LHS, and then in part B we had to calculate the divergene of F, which came to be 0. and part C asks us how can this be? Since in part A we used the...- FaraDazed
- Thread
- Replies: 7
- Forum: Advanced Physics Homework Help
-
W
Volume integral in cylindrical coordinates
Homework Statement OK, I thought once I knew what the question was asking I'd be able to do it. I was wrong! Consider the volume V inside the cylinder x2 +y2 = 4R2 and between z = (x2 + 3y2)/R and the (x,y) plane, where x, y, z are Cartesian coordinates and R is a constant. Write down a triple...- whatisreality
- Thread
- Replies: 9
- Forum: Calculus and Beyond Homework Help
-
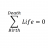
What is the closed volume integral symbol in Microsoft Word?
Hi everyone. I've been curious about a particular symbol, but I've never seen it used or mentioned in any context. I don't really have much information about its usage, so I thought I would ask around and see if anyone knew about its application. I saw this symbol in Microsoft word. How...- STEMucator
- Thread
- Replies: 5
- Forum: Calculus
-

Volume of a quarter cylinder between 2 planes
Homework Statement Find the volume V of the region that lies inside the quarter cylinder 0 ≤ r ≤ 1, 0 ≤ θ ⇐ 1/2 π and between the planes x+y+z=4 and z=0, where (r, θ, z) are cylindrical polar coordinates. Homework Equations integral dV = integral r drdθdz The Attempt at a Solution I...- Matternot
- Thread
- Replies: 3
- Forum: Calculus and Beyond Homework Help
-
C
Integrating a delta function with a spherical volume integral
Homework Statement Integrate $$\int_V \delta^3(\vec r)~ d\tau$$ over all of space by using V as a sphere of radius r centered at the origin, by having r go to infinity. Homework EquationsThe Attempt at a Solution This integral actually came up in a homework problem for my E&M class and I'm...- counterpoint1
- Thread
- Replies: 6
- Forum: Calculus and Beyond Homework Help
-
J
Area and volume integral of vector field
In 2 dimensions given a scalar field f(x,y) is possible to compute the line integral ##\int f ds## and area integral ##\iint f d^2A##. In 3D, given a scalar field f(x,y,z) is possible to compute the surface integral ##\iint f d^2S## and the volume integral too ##\iiint f d^3V##...- Jhenrique
- Thread
- Replies: 4
- Forum: Differential Geometry
-
A
Volume integral turned in to surface + line integral?
Hi, I have a book that makes the equality. \vec{B}dV = (\vec{e_1}B_1 + \vec{e_2}B_2 + \vec{e_1}B_2)dx_1 dx_2 dx_3 \\[1ex] = dx_1 \vec{e}_1(B_1 dx_2 dx_3 ) + dx_2 \vec{e}_2(B_2 dx_1 dx_3 ) + dx_3 \vec{e}_3 (B_3 dx_1 dx_2) = (\vec{B}\cdot d\vec{S}) d\vec{l}. I'm a bit confused as to how it...- AntiElephant
- Thread
- Replies: 2
- Forum: Calculus
-
V
Volume Integral Orthogonal Polynomials
Hello. Homework Statement Basically I want to evaluate the integral as shown in this document: Homework Equations The Attempt at a Solution The integral with the complex exponentials yields a Kronecker Delta. My question is whether this Delta can be taken inside the integral...- VVS
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
P
How can I solve a volume integral question with a trig substitution?
Hi, I was attempting a volume integral question out of a book. I know what the final answer is and what integral i am supposed to work out but I do not know how I am supposed to solve it. I have tried different ways such as integration by substitution and integration by parts but I do not seem... -
Y
Question regarding surface and volume integral
In page 3 of this articlehttp://faculty.uml.edu/cbaird/95.657%282012%29/Helmholtz_Decomposition.pdf I have two question: I use ##\vec r## for ##\vec x## and ##\vec r'## for ##\vec x'## in the article. \vec F(\vec r)=\frac {1}{4\pi}\nabla\left[\int_{v'}\nabla'\cdot\left(\frac{\vec F(\vec... -

Why is the volume integral of zero equal to zero?
If the integral of zero is a constant, then why is the volume integral of zero just zero?- tomwilliam2
- Thread
- Replies: 4
- Forum: General Math
-
J
Volume Integral Set Up for Rotating Objects - Calculus Problem Explained
hi http://www.calcchat.com/book/Calculus-ETF-5e/ part b they want you to rotate it about the y-axis and in part c about the line x = 3. I don't understand this difference in writing for part b... 3^2 - (y^2)^2 And in part c they write (3-y)^2 I don't get it. It is chapter 7 section 2...- Jbreezy
- Thread
-
- Tags
- Integral Set Volume Volume integral
- Replies: 9
- Forum: Calculus and Beyond Homework Help
-
U
Simple Volume Integral, limits of integration
Homework Statement Homework Equations The Attempt at a Solution Are my limits of integration right?- unscientific
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
A
A volume integral over a sphere
Homework Statement ∫∫∫∇.Fdv over x2+y2+z2≤25 F= (x2+y2+z2)(xi+yj+zk) Homework Equations ∫∫∫∇.Fdv = ∫∫ F.n dσ n=∇g/|∇g| The Attempt at a Solution g(x,y,z)=x2+y2+z2-25 taking the surface integral and replacing all (x2+y2+z2) with 25 i got 125 * ∫∫ dσ = 12500π But...- advphys
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
T
Some more volume integral questions
Formulas: Shell Method: dV = 2pi(radius) * (height) * (thickness) Disk method: dV = pi(radius)^2 * (thickness) Question 1 (26-3-15) Statement Using Shell method, find the volume generated by revolving the region bounded by the given curve about the x-axis. x = 4y - y^2 - 3, x = 0...- togo
- Thread
- Replies: 3
- Forum: Calculus and Beyond Homework Help
-
V
Can volume of a rotated function be calculated using definite integrals?
Can I calculate the volume of any function rotated once about the y-axis by multiplying the definite integral of that function by 2*pi*r? For example if we want to generate a solid 3d shape from the function -x^2+1 we multiply the integral of it, (x - x^3 / 3), by 2*pi*1. The reason r is one in...- Vodkacannon
- Thread
- Replies: 2
- Forum: Calculus
-
C
Field theory plane wave volume integral
Hi! I'm used to integrating over infinite spaces when working with QFT so far, but in an exercise I stumbled across a statement that \int_V d^3x e^{-i \vec x \cdot (\vec p - \vec p')} = V \delta_{\vec p \vec p'} It is clear that this is okay when p = p', but it does not seem to make sense...- center o bass
- Thread
- Replies: 5
- Forum: Quantum Physics
-
E
Volume integral of an ellipsoid with spherical coordinates.
Homework Statement By making two successive simple changes of variables, evaluate: I =\int\int\int x^{2} dxdydz inside the volume of the ellipsoid: \frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}+\frac{z^{2}}{c^{2}}=R^{2} Homework Equations dxdydz=r^2 Sin(phi) dphi dtheta dr The...- epiclier
- Thread
- Replies: 7
- Forum: Calculus and Beyond Homework Help
-
I
Area and Volume integral using polar coordinates
Hi I'm working on area and volume integrals. I was wondering, when you convert to do the integral in polar, cylindrical or spherical co-ordinates, is there a standard set of limits for the theta variable in each case? for example from 0 -pi for polar, 0-2pi for cylindrical? If not how... -
1
Calculating Soot Deposition in a Circular Pattern
Homework Statement The soot produced by a garbage incinerator spreads out in a circular pattern. The depth H(r) in millimeters, of the soot deposited each month at distance r kilometers from the incinerator is given by H(r) = 0.115e^(-2r) Write the definite integral for the amount of soot...- 1MileCrash
- Thread
- Replies: 9
- Forum: Calculus and Beyond Homework Help
-
G
How Do You Set the Integration Limits for x and y in a Cone's Volume Integral?
I have the cone x^2 + y^2 <= z^2 with |z| <= 2 The vector function F = (4x, 3z, 5y) With the divergence theorem I managed to reduce the equation to ∫∫∫ 4 dxdydz Now the problem is finding out the limits. I know z goes from 0 to 2, but what about x and y?- geft
- Thread
- Replies: 5
- Forum: Calculus and Beyond Homework Help
-
M
Representing a region as limits of a volume integral
Homework Statement i have the region given as being bounded by x2+y2=4 and z=0 and z=3. this problem asks to prove gauss divergence theorem for a given vector F Homework Equations The Attempt at a Solution As for the volume integral, i had no problem. But for the surface integral, how...- msslowlearner
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
N
Volume integral with 3 formulas mmn 15 3a
find the volume inclosed by z=0 x=y and y^2+z^2=x ?? i am having trouble drawing it i know i should look on th shadow of it on the x-y plane and integrate by it i can project on every plane i want to and build the integral appropriatly i jast can't imagine this shape if i can find...- nhrock3
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
N
Volume integral with 3 formulas mmn 15 3a
find the volume inclosed by z=0 x=y and y^2+z^2=x i am having trouble drawing it i know i should look on th shadow of it on the x-y plane and integrate by it- nhrock3
- Thread
- Replies: 1
- Forum: Calculus and Beyond Homework Help
-
L
Volume integral, how do I find the limits for my integral?
Homework Statement [PLAIN]http://img9.imageshack.us/img9/4537/unledow.png Homework Equations The Attempt at a Solution Hi, does anyone know how to find the integral that needs to be evaluated here? I can't understand how to find it from the region edit: Oh and this is from here, not a take...- LHS
- Thread
- Replies: 6
- Forum: Calculus and Beyond Homework Help
-
M
What is the meaning of the integral of volume?
I have been thinking about the meaning of integrals and derivatives. For instance, the area of a sphere is 4 pi r^2. I can get that. The derivative of the area is 8 pi r or 4 times the circumference of the sphere. The derivative of this is just 8 pi. I can kind of understand that too. Then you...- mljoslinak
- Thread
- Replies: 2
- Forum: Calculus
-
C
Lower and upper limits for a volume integral
Homework Statement I read an e-book about the classical mechanics and didn't know how to find the lower and upper limits for a volume integral. Homework Equations Perhaps, it may be related to the use of similar triangle. The Attempt at a Solution I calculated the x value...- chrisyuen
- Thread
- Replies: 2
- Forum: Introductory Physics Homework Help
-
J
Volume Integral for Vector Field in Spherical Coordinates
Homework Statement I'm stuck on the following vector integral Q(x)=INT[(p(y)/|x-y|)(dy)^3 For a sphere of uniform p (so it is not a function of y in this case). Where x is the position vector of a point lying outside the sphere and y is the position vector of a point lying inside the sphere...- jmz34
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
D
Volume integral of current density
Hi. Can anyone tell me what the volume integral of the current density is? I find it strange, but G.D. Mahan uses it in his book on page 30. He claims that this is in fact the current. I have attached the particular page.- daudaudaudau
- Thread
- Replies: 8
- Forum: Quantum Physics
-
M
Volume integral between sphere r = 1 and z=x^2
Homework Statement Set up the volume integral of x^2 + y^2 + z^2 between z=sqrt(1-x^2-y^2) and z=x^2 Homework Equations Cartesian differential volume element, spherical differential volume element The Attempt at a Solution In class I've been told when doing triple integrals to try...- Moopcan
- Thread
- Replies: 1
- Forum: Calculus and Beyond Homework Help
-
D
Volume integral, am I doing this right?
Hi all, evaluating \int\int\int \nabla . V d\tau over x^{2} + y^{2} \leq 6, 0 \leq z \leq 10 where V is a vector function of just \hat{x} and \hat{y}. Using the divergence theorem, and doing the dot product of V with the normal of the first surface, the two partials w.r.t x and y are...- Damascus Road
- Thread
- Replies: 1
- Forum: Calculus and Beyond Homework Help
-
S
Volume integral to spherical coords to contour integral
Homework Statement V(x) = \int \frac{d^3q}{(2\pi)^3} \frac{-g^2}{|\vec{q}|^2 + m^2} \exp^{i \vec{q} \cdot \vec{x}} = -\frac{g^2}{4\pi^2} \int_0^{\infty} dq q^2 \frac{exp^{iqr}-exp^{-iqr}}{iqr} \frac{1}{q^2+m^2} = \frac{-g^2}{4\pi^2 i r} \int_{-\infty}^{\infty} dq \frac{q...- sbh77
- Thread
- Replies: 4
- Forum: Calculus and Beyond Homework Help
-
I
How Do You Calculate the Mass of a Cone Using Volume Integrals?
Homework Statement "A solid cone is bounded by the surface \theta=\alpha in spherical polar coordinates and the surface z=a. Its mass density is p_0\cos(\theta). By evaluating a volume integral find the mass of the cone. Homework Equations The Attempt at a Solution I can't figure...- implet
- Thread
- Replies: 1
- Forum: Calculus and Beyond Homework Help
-
D
Solving for Volume in a Complex Figure: Ostrogradsky and Spherical Coordinates
Volume Integral! Help! I need help with this: Find volume of figure bounded with surface (x^2+y^2+z^2+1)^2=8*(x^2+y^2) I tried Ostrogradsky, and spherical coordinate system with it, but I can't find boundaries... PLEASE! HELP ME!- DianaSagita
- Thread
- Replies: 3
- Forum: Calculus and Beyond Homework Help
-
C
Calculate Volume of a Portion of Sphere with Given Constraints
Homework Statement Find the volume of the portion of the sphere x^2 + y^2 + z^2 = 4 for which y>1 (or equal to). Homework Equations I would like to do this using a triple integral. The Attempt at a Solution OK, so I tried integrating the element r^2sin(theta)drd(phi)d(theta)...- cpfoxhunt
- Thread
- Replies: 8
- Forum: Calculus and Beyond Homework Help
-
B
Volume integral with two intersecting shapes
The question I am dealing with has to do with the volume contained by two intersecting shapes I have created this integral and can't find a reasonable way of solving it. What is the best approach to solve this: \int_{r=0}^{\pi/2}\int_{u=0}^{2\cos\theta} \sqrt{9-r^2} * r drdu=- bobsmiters
- Thread
- Replies: 8
- Forum: Calculus
-

Compute Volume Integral of $\vec V = xe^{-r}\hat i$: What is 'r'?
I have this vector function: \vec V = xe^{-r}\hat i I have to obtain the volume integral: I = \int(\vec \nabla \cdot \vec V)d^3x What is that 'r' and how do I compute the volume integral?- Reshma
- Thread
- Replies: 10
- Forum: Advanced Physics Homework Help
-
I
Calculate Volume of Enclosed Region with Cylindrical Coordinates
"Find the volume of the region enclosed between the survaces z=x^2 + y^2 and z=2x" I figured that the simplest way of doing this was to switch to a cylindrical co-ordinate system. Can someone check that the limits of integration are then -\frac{\pi}{2}\leq \theta \leq\frac{\pi}{2} 0\leq\ r...- Imo
- Thread
- Replies: 3
- Forum: Introductory Physics Homework Help
-
J
What is the solution to this volume integral problem?
I'm have trouble trying to evaluate the volume integral (shown in question.gif). I've attempted integrating it a few different ways, either achieveing an answer of 3 or 5.75, and I'm not sure where I'm going wrong. (Some of what I've done is in attempted_solution.gif) Any comments...- johnwalton84
- Thread
- Replies: 3
- Forum: Introductory Physics Homework Help