Renormalization Definition and 184 Threads

Renormalization is a collection of techniques in quantum field theory, the statistical mechanics of fields, and the theory of self-similar geometric structures, that are used to treat infinities arising in calculated quantities by altering values of these quantities to compensate for effects of their self-interactions. But even if no infinities arose in loop diagrams in quantum field theory, it could be shown that it would be necessary to renormalize the mass and fields appearing in the original Lagrangian.For example, an electron theory may begin by postulating an electron with an initial mass and charge. In quantum field theory a cloud of virtual particles, such as photons, positrons, and others surrounds and interacts with the initial electron. Accounting for the interactions of the surrounding particles (e.g. collisions at different energies) shows that the electron-system behaves as if it had a different mass and charge than initially postulated. Renormalization, in this example, mathematically replaces the initially postulated mass and charge of an electron with the experimentally observed mass and charge. Mathematics and experiments prove that positrons and more massive particles like protons exhibit precisely the same observed charge as the electron – even in the presence of much stronger interactions and more intense clouds of virtual particles.
Renormalization specifies relationships between parameters in the theory when parameters describing large distance scales differ from parameters describing small distance scales. Physically, the pileup of contributions from an infinity of scales involved in a problem may then result in further infinities. When describing space-time as a continuum, certain statistical and quantum mechanical constructions are not well-defined. To define them, or make them unambiguous, a continuum limit must carefully remove "construction scaffolding" of lattices at various scales. Renormalization procedures are based on the requirement that certain physical quantities (such as the mass and charge of an electron) equal observed (experimental) values. That is, the experimental value of the physical quantity yields practical applications, but due to their empirical nature the observed measurement represents areas of quantum field theory that require deeper derivation from theoretical bases.
Renormalization was first developed in quantum electrodynamics (QED) to make sense of infinite integrals in perturbation theory. Initially viewed as a suspect provisional procedure even by some of its originators, renormalization eventually was embraced as an important and self-consistent actual mechanism of scale physics in several fields of physics and mathematics.
Today, the point of view has shifted: on the basis of the breakthrough renormalization group insights of Nikolay Bogolyubov and Kenneth Wilson, the focus is on variation of physical quantities across contiguous scales, while distant scales are related to each other through "effective" descriptions. All scales are linked in a broadly systematic way, and the actual physics pertinent to each is extracted with the suitable specific computational techniques appropriate for each. Wilson clarified which variables of a system are crucial and which are redundant.
Renormalization is distinct from regularization, another technique to control infinities by assuming the existence of new unknown physics at new scales.

View More On Wikipedia.org
Recent contents Top users
  1. I

    A Questions about scale dependence and renormalization schemes

    Hi, I have several related questions about scale dependence in different renormalization schemes. 1. Is there scale dependence in the on-shell (OS) scheme? Peskin & Schroeder chapter 10 goes through on-shell renormalization without involving an auxiliary scale, but other sources (see...
  2. E

    I Renormalization Scale in Loop Feynman Amplitudes

    I want some clarification on what is done about the ##\mu^{2\epsilon}## renormalization scale parameter in loop amplitudes. I am under the impression that it shows up to restore the mass dimension of an amplitude when the loop momentum integral is reduced from 4 to ##4-2\epsilon## dimensions. As...
  3. S

    A Relationship between bare and renormalized beta functions

    I'm looking at a proof of beta function universality in ##\phi^4## theory, and at one point they do the following step: after imposing that the renormalized coupling ##\lambda## is independent of the cutoff ##\Lambda##, we have $$0= \Lambda \frac {\text{d} \lambda}{\text{d} \Lambda} = \Lambda...
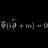
  4. qft-El

    A Solving renormalization group equation in QFT

    I'm learning about the RG equation and Callan-Symanzik equation. In ref.1 they claim to solve the RG equation via the method of characteristics for PDE. Here's a picture of the relevant part: First, the part I don't understand - the one underlined in red. What does "compatible" mean here...
  5. S

    A Renormalized vertex functions in terms of bare ones

    Let ##\Gamma[\varphi] = \Gamma_0[\sqrt{Z}\varphi ] = \Gamma_0[\varphi_0]## be the generating functional for proper vertex functions for a massless ##\phi##-##4## theory. The ##0## subscripts refer to bare quantities, while the quantities without are renormalized. Then $$\tilde{\Gamma}^{(n)}(p_i...
  6. Ramtin123

    A Renormalisation of the Fermionic triangle loop

    I am trying to renormalise the following loop diagram in the Standard Model: Using the Feynman rules, we can write the amplitude as follows: $$ \Gamma_f \sim - tr \int \frac{i}{\displaystyle{\not}\ell -m_f} \frac{i^2}{(\displaystyle{\not}\ell+ \displaystyle{\not}k -m_f)^2} \frac{d^4 \ell}{(2...
  7. E

    A The μ in dimensional regularization

    I have a question about the ##\mu## in dimensional regularization and how it is related to renormalization conditions. I follow the same notation and conventions as in Schwartz. Take QED as an example: $$\mathcal{L} =-\frac{1}{4}\left( F_{0}^{\mu \nu }\right)^{2} +\overline{\psi }_{0}\left(...
  8. AndreasC

    A Main Theorem of Renormalization, but in physicist-speak

    I have been reading a few things about the mathematical formulation of perturbative QFT, specifically in terms of the Stuckelberg-Petermann RG, the Gell-Mann-Low RG, and their difference. Unfortunately I lack the mathematical background to understand these things in depth, and I'm having a...
  9. T

    I QED Renormalization Counterterm Confusion

    Hey all, When looking at the renormalization conditions for QED (see page 332, equation 10.40 from Peskin), there is a condition that requires the photon propagator at ##q^2 = 0## to evaluate to 0. But looking at the expression for the photon propagator counterterm: ##-i(g^{\mu\nu}q^2 -...
  10. S

    I 1-loop Fermion mass correction in toy EFT

    Where does the ##m## in ##(3.2)## come from? It doesn’t seem to enter anywhere in Feynman rules for the given diagram
  11. S

    I Expansion at first order in QCD counterterm

    What is the meaning of the expansion at first order in ##\delta_2## and ##\delta_3## at the second step in the last line? These quantities are not "small" - on the contrary, the entire point is to then take the ##\epsilon \to 0## limit and the counterterms blow up
  12. G

    A Relationship between Wilson's RG and the Callan-Symanzik Equation

    I have taken a Quantum Field Theory course recently in which we first derived the Callan-Symanzik equation and then discussed Wilson's Renormalization. However, I don't think I have a clear understanding of the procedures and how they relate to each other. For the sake of this question, let's...
  13. L

    Renormalization Group:NiemeijerVan Leeuwen Method-Ising Square Lattice

    Hello, I have to solve this problem. I will apply the Niemeijer Van Leeuwen method once I have the probability distribution proper to the renormalization group ,P(s,s'). For example, in the case of a triangular lattice, this distribution is: where I is the block index. However, it is very...
  14. Diracobama2181

    A Perturbative Renormalization in Phi 4 Theory

    For example, after the Lagrangian is renormalized at 1-loop order, it is of the form $$\mathcal{L}=\frac{1}{2}\partial^{\mu}\Phi\partial_{\mu}\Phi-\frac{1}{2}m^2\Phi^2-\frac{\lambda\Phi^4}{4!}-\frac{1}{2}\delta_m^2\Phi^2-\frac{\delta_{\lambda}\Phi^4}{4!}$$. So if I were to attempt to find the...
  15. theittsco

    A Renormalization with hard cutoff of a loop diagram with single vertex

    Trying to solve for the loop contribution when renormalizing a one loop ##\frac{\lambda}{4!}\phi^4## diagram with two external lines in ##d=4## dimensions. After writing down the Feynman rule I see that: $$\frac{(-i\lambda)}{2}\int d^4q \frac{i}{q^2-m_{\phi}^2+i\epsilon} $$ But I see no way to...
  16. P

    A $\phi^4$ in $4 - \epsilon$ dimension renormalization beta function

    Hi all, I am currently studying renormalization group and beta functions. Since I'm not in school there is no one to fix my mis-understandings if any, so I'd really appreciate some feedback. PART I: I wrote this short summary of what I understand of the beta function: Is this reasoning...
  17. R

    I Recommendations for learning renormalization?

    I’ve tried multiple times to learn the methods of renormalization without success. Assume I know quantum mechanics at the level of Griffith’s, Introduction to Quantum Mechanics what’s a good intro to learning renormalization? Robert Clark
  18. K

    I Pauli-Villars regularization for Vacuum Polarization

    Hello! I am currently reading Itzykson Zuber QFT book and on Chapter 7 where for the first time loops are considered. Particular method of dealing with divergences namely Pauli-Villars regularization is considered in section 7-1-1 considering vacuum polarization diagram. I do understand physics...
  19. J

    A Connection between QFT renormalization and field regularization?

    While in QFT we remove infinite energy problem with renormalization procedure, asking e.g. "what is mean energy density in given distance from charged particle", electric filed alone would say $$\rho \propto |E|^2 \propto 1/r^4 $$ But such energy density would integrate to infinity due to...
  20. DaniV

    RG flow of quadrupole coupling in 6+1 dimension electrostatic problem

    I tried to do a Euler Lagrange equation to our Lagrangian: $$\frac{S_\text{eff}}{T}=\int d^6x\left[(\nabla \phi)^2+(\nabla \sigma)^2+\lambda\sigma (\nabla \phi)^2\right]+\frac{S_{p.p}}{T}$$ and then I would like to solve the equation using perturbation theory when ##Q## or somehow...
  21. M

    Mass correction in ##\phi^4##-theory

    Before I start, let me say that I have looked into textbooks and I know this is a standard problem, but I just can't get the result right... My attempt goes as follows: We notice that the amplitude of this diagram is given by $$\begin{align*}K_2(p) &= \frac{i(-i...
  22. M

    A Understanding Counter Term Renormalization in Quantum Field Theory

    Currently, I am reading about counter term renormalization used to eliminate the infinities in the loop calculations involved in QFT calculations. I found somewhere that the insertion of mass counter terms in one loop diagrams is equivalent to the derivative of one loop diagram multiplied with...
  23. tomdodd4598

    I QED - running of coupling (beta function)

    Hey there, I am a little confused about the way most textbooks and notes I've read find the beta function for QED. They find it by looking at how the photon propagator varies with momentum ##q##, in particular in the context of a ##2\rightarrow2## scattering process which is proportional to...
  24. S

    I Wilson's RG trajectories, Lagrangians and many worlds?

    In this article [1] we can read an explanation about Wilson's approach to renormalization I have read that Kenneth G Wilson favoured the path integral/many histories interpretation of Feynman in quantum mechanics to explain it. I was wondering if he did also consider that multiple worlds...
  25. W

    I Renormalization of scalar field theory

    I was reading about the renormalization of ##\phi^4## theory and it was mentioned that in order to renormalize the 2-point function ##\Gamma^{(2)}(p)## we add the counterterm : \delta \mathcal{L}_1 = -\dfrac{gm^2}{32\pi \epsilon^2}\phi^2 to the Lagrangian, which should give rise to a...
  26. tomdodd4598

    I Renormalisation scale and running of the φ^3 coupling constant

    I am still rather new to renormalising QFT, still using the cut-off scheme with counterterms, and have only looked at the ##\varphi^4## model to one loop order (in 4D). In that case, I can renormalise with a counterterm to the one-loop four-point 1PI diagram at a certain energy scale. I can...
  27. Diracobama2181

    How Does Time-Reversal Invariance Affect Phi 4 Theory Renormalization?

    Let ##\phi_{+}=\frac{d^3k}{2\omega_k (2\pi)^3}\int(\hat{a}^{\dagger}(\overrightarrow{k})e^{ikx})## and ##\phi_{-}=\frac{d^3k}{2\omega_k (2\pi)^3}\int(\hat{a}(\overrightarrow{k})e^{-ikx})##. Then ##\phi^4=\phi_{1}\phi_{2}\phi_{3}\phi_{4}=(\phi_{1+}+\phi_{1-})(\phi_{2+}+\phi_{2-}...
  28. S

    A Confusions on QED renormalization

    In many QFT textbooks, we usually see the calculations of vertex function, vacuum polarization and electron self-energy. For example, one calculates the vacuum polarization to correct photon propagator $\langle{\Omega}|T\{A_{\mu}A_{\nu}\}|\Omega\rangle$, where $|\Omega\rangle$ is the ground...
  29. G

    A Renormalization Conditions of QED

    Hello, I'm studying the renormalization of QED. I have the Lagrangian $$\mathscr{L}_{QED}=\mathscr{L}_{physical}+\mathscr{L}_{counterterms}$$ where $$\mathscr{L}_{physical}=-\frac{1}{4}F_{\mu\nu}F^{\mu\nu}+\bar{\psi}(i\gamma^\mu\partial_\mu - m)\psi - e \bar{\psi}\gamma^\mu\psi A_\mu$$...
  30. M

    I Field Renormalization vs. Interaction Picture

    When introducing renormalization of fields, we define the "free Lagrangian" to be the kinetic and mass terms, using the renormalized fields. The remaining kinetic term is treated as an "interaction" counterterm. If we write down the Hamiltonian, the split between "free" and "interaction" terms...
  31. bhobba

    A Does The Use Of The Zeta Function Bypass Renormalization

    I am trying to figure out if the use of the Zeta function allows renormalization to be bypassed. I have formed a preliminary view but would like to hear what others think: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.570.4579&rep=rep1&type=pdf Thanks Bill
  32. G

    A Is renormalization the ideal solution?

    Quantum gravity theories and GUTs are nonrenormalizable theories, but does this actually mean that these theories must be flawed, or does it mean that renormalization must be a flawed concept, or is this not actually a problem? If it is impossible to produce a renormalizable quantum gravity...
  33. R

    I Young physicist in seek of guidance

    Is there anyone on here who could help me fill in my gaps in quantum field theory up to renormalization? I know how to canonically quantize a theory and how to use scalars (spin 0), vectors (spin 1) and spinors (spin 1/2) but lack more advanced knowledge like renormalization which I could...
  34. M

    Beta-function for the Gross-Neveu model

    In the Peskin & Schroeder textbook, the ##\beta## function for the Gross-Neveu model is discussed in problem 12.2. After computing it, I have tried checking my results with some solutions found online. My problem is that they all disagree among each other (something quite recurrent for this book...
  35. A

    A Renormalization (Electron self energy)

    Hello everybody! I have a big question about the renormalization: I do not understand why the "renormalization condition" is to impose the tree level result. Now I will explain it better. Let's take, for example, the electron self energy. The tree-level contribution is the simple fermionic...
  36. A

    What Are the Conditions for On-Shell Renormalization in QFT?

    Homework Statement Show that, after considering all 1 particle irreducible diagrams, the bare scalar propagator becomes: $$D_F (p)=\frac{i}{p^2-m^2-\Sigma (p^2)}$$ And that the residue of the pole is shifted to a new value, and beomes...
  37. Angel Kld

    A On non-perturbative renormalization and gravity

    If we were to find some way to make the graviton self-interaction easily calculable, would applying non-perturbative renormalization on it seem any promising?
  38. A

    I What is the point of regularization?

    Take for example dimensional regularization. Is it correct to say that the main point of the dimensional regularization of divergent momentum integrals in QFT is to express the divergence of these integrals in such a way that they can be absorbed into the counterterms? Can someone tell me what...
  39. Ken Gallock

    A QED: redshifting light and infrared divergence

    I am looking for some resources describing the following content: A light with wavelength ##\lambda## is propagating in flat spacetime. The light redshifts as its wavelength gets larger and larger. In quantum field theory, this causes an infrared divergence of the field. What I want to know...
  40. ohwilleke

    I Is there new LHC data on coupling constant running?

    Question Has the LHC released any papers or reports on the observed running of any of the three Standard Model coupling constants with energy scale from either Run-1 or Run-2 data (or both data sets)? Last time I looked I couldn't find any data As of January 2014, I had not locate any papers...
  41. MathematicalPhysicist

    Renormalization in Two Dimensions

    Homework Statement I am looking for references for the scalar field theory in one-space, one-time dimension defined by: $$\mathcal{L}=-\frac{1}{2}(\partial_{\mu}\phi)^2-\frac{1}{2}m_{2,0}^2\phi^2-\frac{1}{4!}m_{4,0}^2\phi^4-\frac{1}{6!}m_{6,0}^2\phi^6$$ That explains why the only divergences...
  42. ohwilleke

    I Proton Decay At The Highest Possible Energies

    Proton decay has not been observed and has been constrained to be extremely rare in ordinary low temperature situations, if it happens at all (the Standard Model says it doesn't happen at all, because there are no lighter decay products that would not violate conservation of baryon number)...
  43. Giulio Prisco

    A Did nature or physicists invent the renormalization group?

    Or in other words: The renormalization group is a systematic theoretical framework and a set of elegant (and often effective) mathematical techniques to build effective field theories, valid at large scales, by smoothing out irrelevant fluctuations at smaller scales. But does the...
  44. D

    A Renormalisation: what are the physical observables?

    I'm trying to understand renormalisation properly, however, I've run into a few stumbling blocks. To set the scene, I've been reading Matthew Schwartz's "Quantum Field Theory & the Standard Model", in particular the section on mass renormalisation in QED. As I understand it, in order to tame the...
  45. Urs Schreiber

    Mathematical Quantum Field Theory - Renormalization - Comments

    Greg Bernhardt submitted a new PF Insights post Mathematical Quantum Field Theory - Renormalization Continue reading the Original PF Insights Post.
  46. hilbert2

    A Coupling constants with fractional dimensions

    Most QFT texts, such as Peskin&Schroeder and D. Tong's lecture notes, contain a mention that the renormalizability of an interacting theory requires the coupling constants to have correct dimensions, making scalar fields with ##\phi^5 , \phi^6, \dots## interactions uninteresting. Maybe there are...
  47. A

    I IR divergences and total energies....

    I've done some recent reading on IR divergences (propagators becoming singular, etc.). I believe I understand collinear divergences (to some extent)... but I'm not sure about total energies for (primarily) soft photons. In all scattering experiments, total energy should be conserved - but if...
  48. diegzumillo

    A LSZ, perturbation and renormalization

    My current understanding of renormalization is that the LSZ formula requires normalized fields. So when you normalize them you get some extra parameters from the regularization procedure you encounter along the way. It's an upgrade on my previous understanding of it as some artificial way of...
  49. T

    A Quantum Gravity: Renormalization vs. Effective Field Theory

    In quantum gravity, I get 'mixed signals' as regards renormalizability. My state of confusion is being caused, I suspect, by an incomplete understanding of what is covered under t'Hooft's 1972 proof that non-Abelian gauge theories are renormalizable. ( = Nobel Prize 1999). Specifically, some...
  50. J

    A How are renormalizability and locality connected?

    In his paper Quantum Field Theory: renormalization and the renormalization group Zinn-Justin states: Low energy physics does not depend on all the details of the microscopic model because some RG has an IR fixed point or at least a low dimension fixed surface. Of course at this stage the next...
Back
Top