You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
What is Statistical phyisics: Definition and 27 Discussions
No Wikipedia entry exists for this tag
-

Spectral Intensity Formula by Using Frequency
I found there is kind of solution in Pointon's book: An Introduction to Statistical Physics for Students. But I don't know how to find intensity by using frequency.- benagastov
- Thread
- Replies: 5
- Forum: Advanced Physics Homework Help
-
F
A Fourier transform and Cosmic variance - a few precisions
I cite an original report of a colleague : 1) I can't manage to proove that the statistical error is formulated like : ##\dfrac{\sigma (P (k))}{P(k)} = \sqrt{\dfrac {2}{N_{k} -1}}_{\text{with}} N_{k} \approx 4\pi \left(\dfrac{k}{dk}\right)^{2}## and why it is considered like a relative error ... -
L
Can we study an odd number sized lattice model at half filling?
So one can numerically study (I am interested in exact diagonalization) any 1D lattice model with ##L## sites and ##N## number of particles. At half filling, ##L/N = 2##. My question to a professor was that can we study a system of size ##L = 31## at half filling? He replied yes, there is a way...- Luqman Saleem
- Thread
- Replies: 1
- Forum: Atomic and Condensed Matter
-
How to compute a mean square average
Homework Statement We know that $$< (x_1 - x_2)^2 > = \frac{K_bT}{K}$$ $$< (x_2 - x_3)^2 > = \frac{K_bT}{\gamma}$$ What's ##< (x_3 - x_1)^2 >## equal to? Homework EquationsThe Attempt at a Solution I have tried: ##< (x_2 - x_3)^2 > - < (x_1 - x_2)^2 >## but did not get ##< (x_3 - x_1)^2...- JD_PM
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
J
Expectation Value of a Stochastic Quantity
Homework Statement I'm working on a process similar to geometric brownian motion (a process with multiplicative noise), and I need to calculate the following expectation/mean; \langle y \rangle=\langle e^{\int_{0}^{x}\xi(t)dt}\rangle Where \xi(t) is delta-correlated so that... -

Canonical ensemble of a simplified DNA representation
Question Form the canoncial partition using the following conditions: 2 N-particles long strands can join each other at the i-th particle to form a double helix chain. Otherwise, the i-th particle of each strand can also be left unattached, leaving the chain "open" An "open" link gives the...- zexxa
- Thread
- Replies: 3
- Forum: Advanced Physics Homework Help
-

A The Future of "Soft Matter Physics"
Hi all, I am trying to learn more about this field. Whether you work or have worked in this area or not, I would like to know where Soft Matter is going in terms of theory and applications. Thanks, A.D. *I apologize in advance if there are already many threads specifically addressing this...- AD MCFC
- Thread
- Replies: 5
- Forum: Atomic and Condensed Matter
-
S
I Is there a frequency cutoff for Debye theory of capillary waves?
I calculated the energy density of capillary waves with Debye method (pretty much Debye model in 2D), and I assumed there is a frequency cutoff for capillary waves as well. However, when I checked my work with solution I was quite surprised that the solution suggests there is no such a cuttoff...- Shing
- Thread
- Replies: 1
- Forum: Atomic and Condensed Matter
-

XXh spin chain, colleration function
Homework Statement So given XXh chain: $$\hat{H} = -J \sum ( S^x_j S^x_{j+1} +S^y_j S^y_{j+1}) + h \sum S^z_j $$ Requred to find $$\langle g| S^z_{j} S^z_{j+n} | g \rangle$$, where g is ground state. 2. The attempt at a solution Using Jordan-Wigner transform firstly I abtain: $$\hat{H} =...- Illuminatio fit
- Thread
- Replies: 1
- Forum: Advanced Physics Homework Help
-

Find the maximum of E_a when the entropy is at its maximum
Homework Statement Show that \Gamma_T is maximum at E_a = \frac{N_aE}{N_a+N_b} Homework Equations The expression for \Gamma(E) when N\gg 1 \Gamma_T = C_aC_b exp \left( -\frac{E_a^2}{2N_a\mu_B^2h^2} \right) exp \left[ - \frac{(E-E_a)^2}{2N_b\mu_B^2H^2} \right] where C_a and C_b are...- WeiShan Ng
- Thread
- Replies: 3
- Forum: Advanced Physics Homework Help
-
L
Is average time between and after collision same for a gas?
I am stuck on this concept in my physics book where the author claims that in a low density ionic gas the average of the time between collision and average of the time taken from last collision in ions is same. He further states that the average time to the next collision is same as the average...- Linop
- Thread
- Replies: 2
- Forum: Mechanics
-
M
Internal Energy of virial expansion
Hello, I have some trouble understanding the virial expansion of the ideal gas. 1. Homework Statement I have given the state equation: $$ pV = N k_b T \left(1+\frac{A\left(T\right)}{V}\right) $$Homework Equations [/B] and a hint how to calculate the caloric equation of state $$...- Mikhail_MR
- Thread
- Replies: 10
- Forum: Advanced Physics Homework Help
-
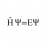
Ideal gas pressure from Maxwell-Boltzmann distribution
Hi everyone I'm having trouble with solving an exercise in statistical physics. I need to argue why the average number of particles with a velocity between ##v## and ##v+dv## that hit a surface area ##A## on the container wall in a time interval ##\Delta t## is $$N_{collision}=v_{x}A\Delta t...- H Psi equal E Psi
- Thread
- Replies: 4
- Forum: Electromagnetism
-

A What is wrong with particle acceleration based fusion?
Recently, I was thinking about fusion and this thought struck my mind. In tokamaks, the plasma is heated to extremely high temperatures in order to supply enough energy to the ions for them to fuse. But since, the plasma follows a Boltzmann maxwell distribution curve,only a few ions have have...- chandrahas
- Thread
- Replies: 5
- Forum: High Energy, Nuclear, Particle Physics
-

I Calculating Collision Cross Section in Fusion Reactors
Most Fusion reactors, and the leading ones like JET, use high temp. plasma and confine it. So, the plasma would approximate the Maxwell- Boltzmann distribution. This means that only a small portion of the plasma has enough energy to fuse. But, collisions are much more often, right? Since not all...- chandrahas
- Thread
- Replies: 29
- Forum: High Energy, Nuclear, Particle Physics
-
C
Other Statistical Physics and Biology
I'm undergrad physics student and I have read some statistical physics like equilibrium statistical physics, Langevin model and Fokker-Planck equation. I have developed interest in application of statistical physics in biology like protein folding. So what are the other research topics that lie...- cKatke
- Thread
- Replies: 1
- Forum: STEM Academic Advising
-

I Relation between energy levels and volume.
I've just started with statistical mechanics and arrived at the part where they relate entropy to the number of microstates for a given system. The derivation starts of by adding an amount of heat ##\delta Q## to a system and observing the resulting change in internal energy : $$\delta U =...- weezy
- Thread
- Replies: 6
- Forum: Classical Physics
-

A Where does statistical physics/mechanics fit in with QFT,GR?
We have two theories namely,Quantum Field Theory which works very well at sub-atomic scales, and the General Relativity which works very well at very large scales.So, my question is where does statistical physics/mechanics fit in? What role statistical physics/mechanics play in today's modern...- Indiana
- Thread
- Replies: 1
- Forum: Quantum Physics
-
J
Heat capacity under constant pressure or volume question
HOMEWORK POSTED IN WRONG FORUM, SO NO TEMPLATE I have encountered a problem at the university in which there is a thermally isolated container of constant volume in which the number of particles and temperature change with time(the temperature increases). The change in particle number ensures...- Joker93
- Thread
- Replies: 61
- Forum: Introductory Physics Homework Help
-
Z
Show interparticle spacing is much greater than wavelength
I have a past paper question from statistical physics: By assuming that ##\hbar^2 k^2=p^2##, I arrived at the result: The interparticle spacing, ##a^3 =\frac{V}{N}## is $$ a^3 >> e^{-\frac{p^2}{2mk_B T}} \lambda_{deB}^3$$ Is my assumption correct? and does the result complete the purpose of...- zeeshahmad
- Thread
- Replies: 1
- Forum: Advanced Physics Homework Help
-

Understanding the Uniform Probability Distribution in Statistical Ensembles
Homework Statement Confused about what a statistical ensemble actually means. Why does the ensemble have to have a uniform probability distribution at equilibrium? [If my definition of an ensemble is correct] The Attempt at a Solution This is what I understand so far: [/B] For any given...- bananabandana
- Thread
- Replies: 231
- Forum: Quantum Physics
-
L
Other Research topics in Statistical Physics
Currently I'm in the last year of the Physics course and I've been thinking about working in a project of undergraduate research, specifically in Statistical Physics. Two years ago I've already done a project like that in Fluid Mechanics combined with Gauge Theories and in that project I've... -

Relationship between density and probability in diffusion
Homework Statement Consider the diffusion of a drop of ink in a water vase. The density of the ink is ## \rho (\vec{r}, t) ##, and the probability ##P(\vec{r}, t)## obeys the diffusion equation. What is the relationship between ##\rho (\vec{r}, t)## and ##P(\vec{r}, t)##? Homework...- It's me
- Thread
- Replies: 6
- Forum: Introductory Physics Homework Help
-
P
Probability that a magnetic dipole is oriented with theta
1. the problem goes like this : The energy of interaction of a classical magnetic dipole with the magnetic field B is given by E = −μ·B. The sum over microstates becomes an integral over all directions of μ. The direction of μ in three dimensions is given by the angles θ and φ of a spherical...- potatowhisperer
- Thread
- Replies: 1
- Forum: Advanced Physics Homework Help
-

A Normalization constant of Fermi Dirac distribution function
Fermi-Dirac distribution function is given by f(E)=(1)/(Aexp{E/k_{B}T}+1) here A is the normalization constant? How we can get A? E is the energy, k_{B} is the Boltzmann constant and T is the temperature. thank you -
S
Probability density function of simple Mass-Spring system.
Homework Statement We know that after long run of simple mass-spring system, there should be a probability of finding the mass at certain points between -A and A.. Obviously in probability of finding the particle near A or -A is higher than finding the particle at 0, because the speed is the... -
J
Magnetic moment of paramagnetic crystal
Hello, I've been having some trouble with a paramagnetism problem from my Statistical Mechanics class textbook (F. Mandl, Statistical Physics, 2nd edition, p. 25). The problem is as follows 1. Homework Statement 2. Homework Equations 1. The temperature parameter \displaystyle{ \beta =...